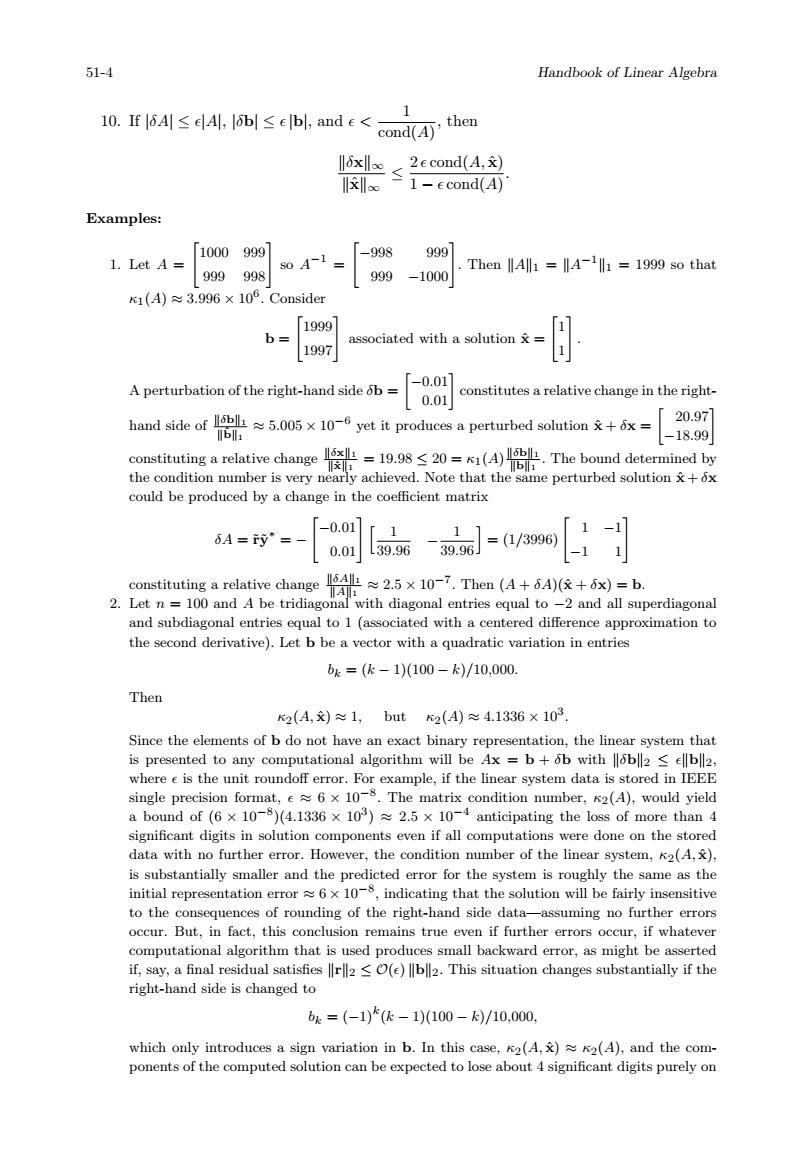
51-4 Handbook of Linear Algebra 10.If15A≤dAl,l5bl≤ebl,ande<eomd④then s x Examples: [1000999] 1.Let A= 999998 0A1= 「-9989991 999 1000 Then IlAll =A-=1999 so that K1(A)≈3.996×10.Consider associated with a solution&= 1997 Aperturbation of the right-hand side b.01 「-0.011 constitutes a relative change in the right. hand side of微t≈5.05x10-6 erodcerbd soion文+ix= 「20.97 -18.99 constituting a relative change=19.9820=(A).The bound determined by the condition number is very nearly achieved.Note that the same perturbed solution+6x could be produced by a change in the coefficient matrix -0.01 6A==- ,学aab and subdiagonal entries equal to 1 (associated with a centered difference approximation to the second derivative).Let b be a vector with a quadratic variation in entries bk=(k-1)(100-k)/10,000, Then 2(4,)≈1，but2(A)≈4.1336×103 Since the elements of b do not have an exact binary representation,the linear system that is presented to any computational algorithm will be Ax b+6b with llob2 ellbl2 where e is the unit roundoff error.For example,if the linear system data is stored in IEE single precision format The matrix condition num (A),would yield bound of (6 x 10 1336 ng the digit 2 stantially 08 to th But in fact this clus of the orithm that is used true back ht be if s s substantially if the right-hand side is changed to bk=(-1)(k-1)(100-)/10.000, which only introduces a sign variation in b.In this case .(A.文)≈(A).and the com ponents of the computed solution can be expected to lose about 4 significant digits purely on51-4 Handbook of Linear Algebra 10. If |δA| ≤ |A|, |δb| ≤  |b|, and  < 1 cond(A) , then kδxk∞ kxˆk∞ ≤ 2  cond(A, xˆ) 1 −  cond(A) . Examples: 1. Let A = " 1000 999 999 998# so A −1 = " −998 999 999 −1000# . Then kAk1 = kA −1 k1 = 1999 so that κ1(A) ≈ 3.996 × 106 . Consider b = " 1999 1997# associated with a solution xˆ = " 1 1 # . A perturbation of the right-hand side δb =  −0.01 0.01 constitutes a relative change in the right￾hand side of kδbk1 kbˆk1 ≈ 5.005 × 10−6 yet it produces a perturbed solution xˆ + δx =  20.97 −18.99 constituting a relative change kδxk1 kxˆk1 = 19.98 ≤ 20 = κ1(A) kδbk1 kbk1 . The bound determined by the condition number is very nearly achieved. Note that the same perturbed solution xˆ +δx could be produced by a change in the coefficient matrix δA = ˜ry˜ ∗ = − " −0.01 0.01# h 1 39.96 − 1 39.96 i = (1/3996) " 1 −1 −1 1# constituting a relative change kδAk1 kAk1 ≈ 2.5 × 10−7 . Then (A + δA)(xˆ + δx) = b. 2. Let n = 100 and A be tridiagonal with diagonal entries equal to −2 and all superdiagonal and subdiagonal entries equal to 1 (associated with a centered difference approximation to the second derivative). Let b be a vector with a quadratic variation in entries bk = (k − 1)(100 − k)/10,000. Then κ2(A, xˆ) ≈ 1, but κ2(A) ≈ 4.1336 × 103 . Since the elements of b do not have an exact binary representation, the linear system that is presented to any computational algorithm will be Ax = b + δb with kδbk2 ≤ kbk2, where  is the unit roundoff error. For example, if the linear system data is stored in IEEE single precision format,  ≈ 6 × 10−8 . The matrix condition number, κ2(A), would yield a bound of (6 × 10−8 )(4.1336 × 103 ) ≈ 2.5 × 10−4 anticipating the loss of more than 4 significant digits in solution components even if all computations were done on the stored data with no further error. However, the condition number of the linear system, κ2(A, xˆ), is substantially smaller and the predicted error for the system is roughly the same as the initial representation error ≈ 6 × 10−8 , indicating that the solution will be fairly insensitive to the consequences of rounding of the right-hand side data—assuming no further errors occur. But, in fact, this conclusion remains true even if further errors occur, if whatever computational algorithm that is used produces small backward error, as might be asserted if, say, a final residual satisfies krk2 ≤ O() kbk2. This situation changes substantially if the right-hand side is changed to bk = (−1)k (k − 1)(100 − k)/10,000, which only introduces a sign variation in b. In this case, κ2(A, xˆ) ≈ κ2(A), and the com￾ponents of the computed solution can be expected to lose about 4 significant digits purely on