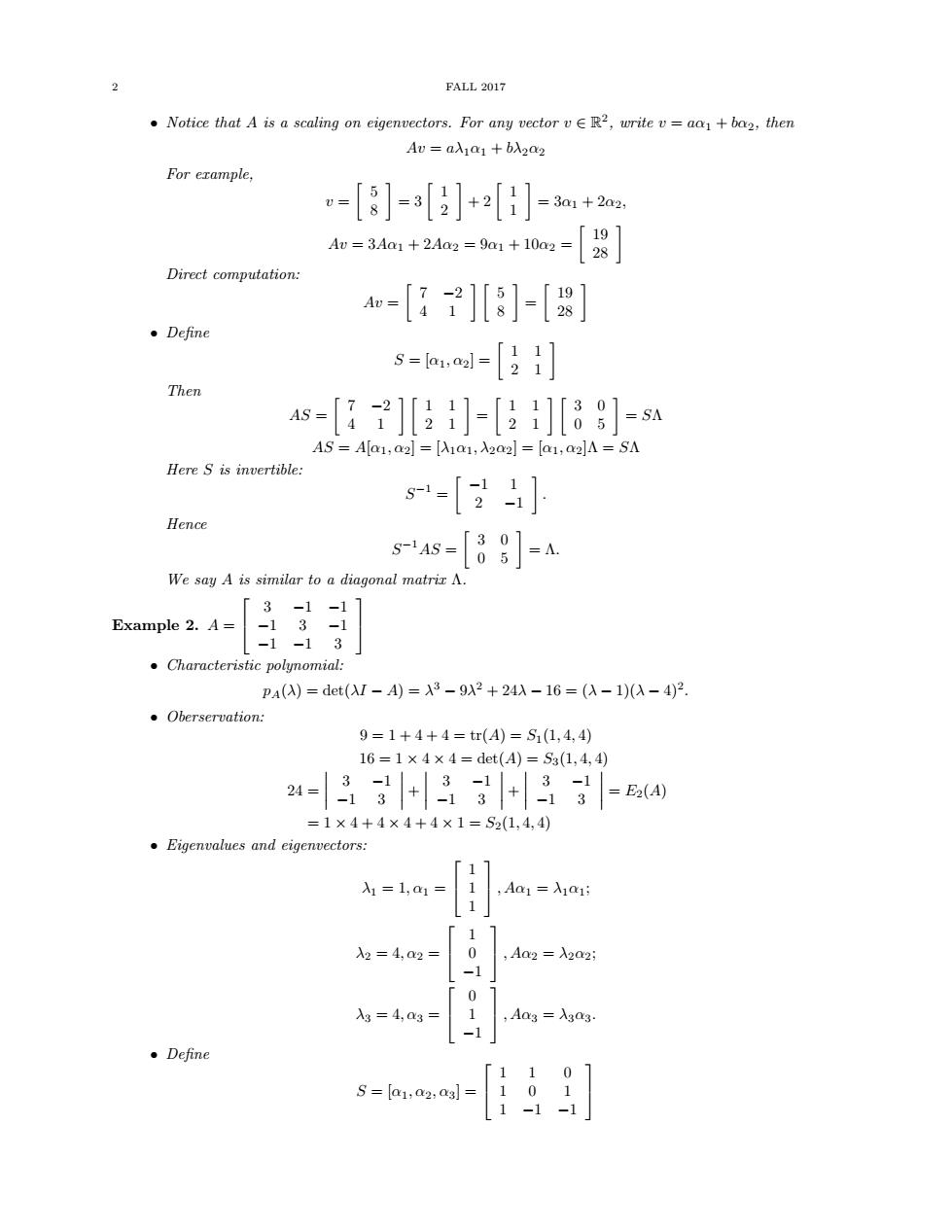
2 FALL 2017 .Notice that A is a scaling on eigenvectors.For any vectorR2,write+bo,then Av=aA101+bA202 For erample, e=[8]=3[2l+2[1=a+2ae 4e=3ha+2a:=4a+i0ag=[28] Direct computation -[][8]-[] ·Define s=a,al=[21] Then 4s-[2][:]-[&l[88]-sm AS A[a1,a2]=[A1a1.A2a2][a1,a21A=SA Here S is invertible: s-[1] Hence S-AS-05-A. We say A is similar to a diagonal matrirA. Example 2.A= .Characteristic polymomial: PA()=det(M-A)=X3-9A2+24A-16=(A-1(A-4)2. .Oberservation. 9=1+4+4=tr(4)=S(1,4,4) 16=1×4×4=det(4)=S5(1,4,4) =1×4+4×4+4×1=S2(1.4,4) .Eigenvalues and eigenvectors: 「1 1=1,01=1 :Ao1 =A01: 1 2=4,a2 0 -1 0 g=4,a3= Ao3 =Ag03 -1 ·Define S=[a1,02,03]=2 FALL 2017 • Notice that A is a scaling on eigenvectors. For any vector v ∈ R 2 , write v = aα1 + bα2, then Av = aλ1α1 + bλ2α2 For example, v =  5 8  = 3  1 2  + 2  1 1  = 3α1 + 2α2, Av = 3Aα1 + 2Aα2 = 9α1 + 10α2 =  19 28  Direct computation: Av =  7 −2 4 1   5 8  =  19 28  • Define S = [α1, α2] =  1 1 2 1  Then AS =  7 −2 4 1   1 1 2 1  =  1 1 2 1   3 0 0 5  = SΛ AS = A[α1, α2] = [λ1α1, λ2α2] = [α1, α2]Λ = SΛ Here S is invertible: S −1 =  −1 1 2 −1  . Hence S −1AS =  3 0 0 5  = Λ. We say A is similar to a diagonal matrix Λ. Example 2. A =   3 −1 −1 −1 3 −1 −1 −1 3   • Characteristic polynomial: pA(λ) = det(λI − A) = λ 3 − 9λ 2 + 24λ − 16 = (λ − 1)(λ − 4)2 . • Oberservation: 9 = 1 + 4 + 4 = tr(A) = S1(1, 4, 4) 16 = 1 × 4 × 4 = det(A) = S3(1, 4, 4) 24 =     3 −1 −1 3     +     3 −1 −1 3     +     3 −1 −1 3     = E2(A) = 1 × 4 + 4 × 4 + 4 × 1 = S2(1, 4, 4) • Eigenvalues and eigenvectors: λ1 = 1, α1 =   1 1 1   , Aα1 = λ1α1; λ2 = 4, α2 =   1 0 −1   , Aα2 = λ2α2; λ3 = 4, α3 =   0 1 −1   , Aα3 = λ3α3. • Define S = [α1, α2, α3] =   1 1 0 1 0 1 1 −1 −1  