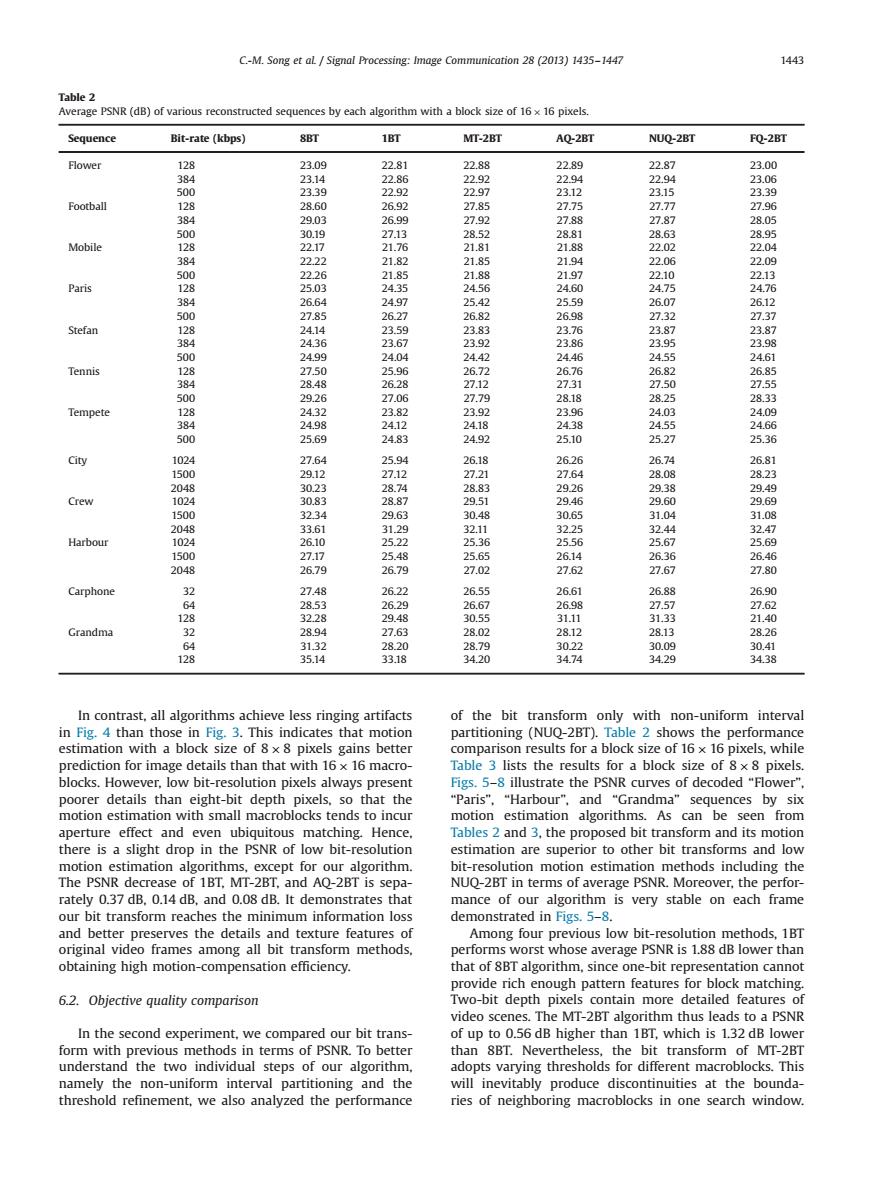
C.-M.Song et aL Signal Processing:Image Communication 28 (2013)1435-1447 1443 Table 2 Average PSNR(dB)of various reconstructed sequences by each algorithm with a block size of 16 x 16 pixels Sequence Bit-rate(kbps) 8BT 1BT MT-2BT AQ-2BT NUQ-2BT FQ-2BT Flower 128 23.09 22.81 22.88 22.89 22.87 23.00 384 23.14 22.86 22.92 22.94 22.94 23.06 500 23.39 22.92 22.97 23.12 2315 23.39 Football 128 28.60 26.92 27.85 27.75 27.77 27.96 384 29.03 26.99 27.92 27.88 27.87 28.05 500 3019 27.13 28.52 28.81 28.63 28.95 Mobile 128 22.17 21.76 21.81 21.88 22.02 22.04 384 22.22 21.82 21.85 2194 22.06 22.09 500 22.26 21.85 21.88 21.97 22.10 22.13 Paris 128 25.03 24.35 24.56 24.60 24.75 24.76 384 26.64 24.97 25.42 25.59 26.07 26.12 500 27.85 26.27 26.82 26.98 27.32 27.37 Stefan 128 24.14 23.59 23.83 23.76 23.87 23.87 384 24.36 23.67 23.92 23.86 23.95 23.98 500 24.99 24.04 24.42 24.46 24.55 24.61 Tennis 128 27.50 25.96 26.72 26.76 26.82 26.85 384 28.48 26.28 27.12 2731 27.50 27.55 500 29.26 27.06 27.79 28.18 28.25 28.33 Tempete 128 24.32 23.82 23.92 23.96 24.03 24.09 384 24.98 2412 24.18 24.38 24.55 24.66 500 25.69 24.83 24.92 25.10 25.27 25.36 City 1024 27.64 25.94 26.18 2626 26.74 26.81 1500 29.12 27.12 27.21 27.64 28.08 28.23 2048 30.23 28.74 28.83 2926 29.38 29.49 Crew 1024 30.83 28.87 29.51 29.46 29.60 29.69 1500 32.34 29.63 30.48 30.65 31.04 31.08 2048 33.61 31.29 32.11 32.25 32.44 32.47 Harbour 1024 26.10 25.22 25.36 25.56 25.67 25.69 1500 27.17 25.48 25.65 26.14 26.36 26.46 2048 26.79 26.79 27.02 27.62 27.67 27.80 Carphone 27.48 26.22 26.55 26.61 26.88 26.90 4 28.53 26.29 26.67 26.98 27.57 27.62 12 32.28 29.48 30.55 31.11 31.33 21.40 Grandma 2 28.94 27.63 28.02 28.12 28.13 28.26 64 31.32 28.20 28.79 3022 30.09 30.41 128 35.14 33.18 34.20 34.74 34.29 34.38 In contrast,all algorithms achieve less ringing artifacts of the bit transform only with non-uniform interval in Fig.4 than those in Fig.3.This indicates that motion partitioning (NUQ-2BT).Table 2 shows the performance estimation with a block size of 8 x 8 pixels gains better comparison results for a block size of 16 x 16 pixels,while prediction for image details than that with 16 x 16 macro- Table 3 lists the results for a block size of 8x8 pixels. blocks.However,low bit-resolution pixels always present Figs.5-8 illustrate the PSNR curves of decoded "Flower", poorer details than eight-bit depth pixels.so that the "Paris","Harbour",and "Grandma"sequences by six motion estimation with small macroblocks tends to incur motion estimation algorithms.As can be seen from aperture effect and even ubiquitous matching.Hence, Tables 2 and 3,the proposed bit transform and its motion there is a slight drop in the PSNR of low bit-resolution estimation are superior to other bit transforms and low motion estimation algorithms,except for our algorithm. bit-resolution motion estimation methods including the The PSNR decrease of 1BT,MT-2BT,and AQ-2BT is sepa- NUQ-2BT in terms of average PSNR.Moreover,the perfor- rately 0.37 dB.0.14 dB,and 0.08 dB.It demonstrates that mance of our algorithm is very stable on each frame our bit transform reaches the minimum information loss demonstrated in Figs.5-8. and better preserves the details and texture features of Among four previous low bit-resolution methods,1BT original video frames among all bit transform methods, performs worst whose average PSNR is 1.88 dB lower than obtaining high motion-compensation efficiency. that of 8BT algorithm,since one-bit representation cannot provide rich enough pattern features for block matching. 6.2.Objective quality comparison Two-bit depth pixels contain more detailed features of video scenes.The MT-2BT algorithm thus leads to a PSNR In the second experiment,we compared our bit trans- of up to 0.56 dB higher than 1BT,which is 1.32 dB lower form with previous methods in terms of PSNR.To better than 8BT.Nevertheless,the bit transform of MT-2BT understand the two individual steps of our algorithm, adopts varying thresholds for different macroblocks.This namely the non-uniform interval partitioning and the will inevitably produce discontinuities at the bounda- threshold refinement,we also analyzed the performance ries of neighboring macroblocks in one search window.In contrast, all algorithms achieve less ringing artifacts in Fig. 4 than those in Fig. 3. This indicates that motion estimation with a block size of 8 8 pixels gains better prediction for image details than that with 16 16 macro￾blocks. However, low bit-resolution pixels always present poorer details than eight-bit depth pixels, so that the motion estimation with small macroblocks tends to incur aperture effect and even ubiquitous matching. Hence, there is a slight drop in the PSNR of low bit-resolution motion estimation algorithms, except for our algorithm. The PSNR decrease of 1BT, MT-2BT, and AQ-2BT is sepa￾rately 0.37 dB, 0.14 dB, and 0.08 dB. It demonstrates that our bit transform reaches the minimum information loss and better preserves the details and texture features of original video frames among all bit transform methods, obtaining high motion-compensation efficiency. 6.2. Objective quality comparison In the second experiment, we compared our bit trans￾form with previous methods in terms of PSNR. To better understand the two individual steps of our algorithm, namely the non-uniform interval partitioning and the threshold refinement, we also analyzed the performance of the bit transform only with non-uniform interval partitioning (NUQ-2BT). Table 2 shows the performance comparison results for a block size of 16 16 pixels, while Table 3 lists the results for a block size of 8 8 pixels. Figs. 5–8 illustrate the PSNR curves of decoded “Flower”, “Paris”, “Harbour”, and “Grandma” sequences by six motion estimation algorithms. As can be seen from Tables 2 and 3, the proposed bit transform and its motion estimation are superior to other bit transforms and low bit-resolution motion estimation methods including the NUQ-2BT in terms of average PSNR. Moreover, the perfor￾mance of our algorithm is very stable on each frame demonstrated in Figs. 5–8. Among four previous low bit-resolution methods, 1BT performs worst whose average PSNR is 1.88 dB lower than that of 8BT algorithm, since one-bit representation cannot provide rich enough pattern features for block matching. Two-bit depth pixels contain more detailed features of video scenes. The MT-2BT algorithm thus leads to a PSNR of up to 0.56 dB higher than 1BT, which is 1.32 dB lower than 8BT. Nevertheless, the bit transform of MT-2BT adopts varying thresholds for different macroblocks. This will inevitably produce discontinuities at the bounda￾ries of neighboring macroblocks in one search window. Table 2 Average PSNR (dB) of various reconstructed sequences by each algorithm with a block size of 16 16 pixels. Sequence Bit-rate (kbps) 8BT 1BT MT-2BT AQ-2BT NUQ-2BT FQ-2BT Flower 128 23.09 22.81 22.88 22.89 22.87 23.00 384 23.14 22.86 22.92 22.94 22.94 23.06 500 23.39 22.92 22.97 23.12 23.15 23.39 Football 128 28.60 26.92 27.85 27.75 27.77 27.96 384 29.03 26.99 27.92 27.88 27.87 28.05 500 30.19 27.13 28.52 28.81 28.63 28.95 Mobile 128 22.17 21.76 21.81 21.88 22.02 22.04 384 22.22 21.82 21.85 21.94 22.06 22.09 500 22.26 21.85 21.88 21.97 22.10 22.13 Paris 128 25.03 24.35 24.56 24.60 24.75 24.76 384 26.64 24.97 25.42 25.59 26.07 26.12 500 27.85 26.27 26.82 26.98 27.32 27.37 Stefan 128 24.14 23.59 23.83 23.76 23.87 23.87 384 24.36 23.67 23.92 23.86 23.95 23.98 500 24.99 24.04 24.42 24.46 24.55 24.61 Tennis 128 27.50 25.96 26.72 26.76 26.82 26.85 384 28.48 26.28 27.12 27.31 27.50 27.55 500 29.26 27.06 27.79 28.18 28.25 28.33 Tempete 128 24.32 23.82 23.92 23.96 24.03 24.09 384 24.98 24.12 24.18 24.38 24.55 24.66 500 25.69 24.83 24.92 25.10 25.27 25.36 City 1024 27.64 25.94 26.18 26.26 26.74 26.81 1500 29.12 27.12 27.21 27.64 28.08 28.23 2048 30.23 28.74 28.83 29.26 29.38 29.49 Crew 1024 30.83 28.87 29.51 29.46 29.60 29.69 1500 32.34 29.63 30.48 30.65 31.04 31.08 2048 33.61 31.29 32.11 32.25 32.44 32.47 Harbour 1024 26.10 25.22 25.36 25.56 25.67 25.69 1500 27.17 25.48 25.65 26.14 26.36 26.46 2048 26.79 26.79 27.02 27.62 27.67 27.80 Carphone 32 27.48 26.22 26.55 26.61 26.88 26.90 64 28.53 26.29 26.67 26.98 27.57 27.62 128 32.28 29.48 30.55 31.11 31.33 21.40 Grandma 32 28.94 27.63 28.02 28.12 28.13 28.26 64 31.32 28.20 28.79 30.22 30.09 30.41 128 35.14 33.18 34.20 34.74 34.29 34.38 C.-M. Song et al. / Signal Processing: Image Communication 28 (2013) 1435–1447 1443