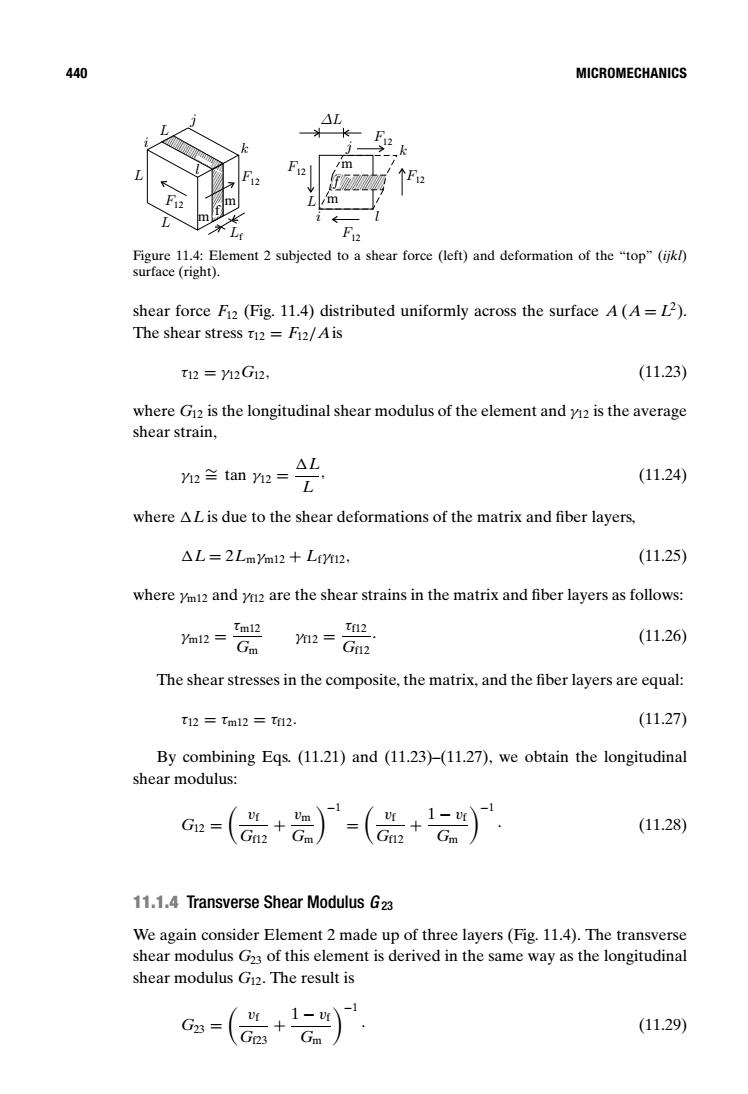
440 MICROMECHANICS /m 12 /m Figure 11.4:Element 2 subjected to a shear force (left)and deformation of the "top"(ijkl) surface(right). shear force F2(Fig.11.4)distributed uniformly across the surface A(A=L2). The shear stress t12 Fi2/Ais T12=12G12, (11.23) where G2 is the longitudinal shear modulus of the element and yi2 is the average shear strain, △L h2兰tanh2= (11.24) where AL is due to the shear deformations of the matrix and fiber layers, △L=2Lmym12+Lf12, (11.25) where ymi2 and yn2 are the shear strains in the matrix and fiber layers as follows: Ym12 Tm12 T12 Gm 12= (11.26) Gn2 The shear stresses in the composite,the matrix,and the fiber layers are equal: T12=Tm12=Tf12, (11.27) By combining Eqs.(11.21)and (11.23)-(11.27),we obtain the longitudinal shear modulus: G12= U (11.28) G12 Gm G12 Gm 11.1.4 Transverse Shear Modulus G23 We again consider Element 2 made up of three layers(Fig.11.4).The transverse shear modulus G23 of this element is derived in the same way as the longitudinal shear modulus G12.The result is G3= 1- (11.29) G3440 MICROMECHANICS L L F12 ∆L m m f j k l i F12 Lf F12 L j k i l F12 F12 F12 f m m L Figure 11.4: Element 2 subjected to a shear force (left) and deformation of the “top” (ijkl) surface (right). shear force F12 (Fig. 11.4) distributed uniformly across the surface A (A = L2). The shear stress τ12 = F12/Ais τ12 = γ12G12, (11.23) where G12 is the longitudinal shear modulus of the element and γ12 is the average shear strain, γ12 ∼= tan γ12 = L L , (11.24) where L is due to the shear deformations of the matrix and fiber layers, L = 2Lmγm12 + Lfγf12, (11.25) where γm12 and γf12 are the shear strains in the matrix and fiber layers as follows: γm12 = τm12 Gm γf12 = τf12 Gf12 . (11.26) The shear stresses in the composite, the matrix, and the fiber layers are equal: τ12 = τm12 = τf12. (11.27) By combining Eqs. (11.21) and (11.23)–(11.27), we obtain the longitudinal shear modulus: G12 =  vf Gf12 + vm Gm −1 =  vf Gf12 + 1 − vf Gm −1 . (11.28) 11.1.4 Transverse Shear Modulus G 23 We again consider Element 2 made up of three layers (Fig. 11.4). The transverse shear modulus G23 of this element is derived in the same way as the longitudinal shear modulus G12. The result is G23 =  vf Gf23 + 1 − vf Gm −1 . (11.29)