正在加载图片...
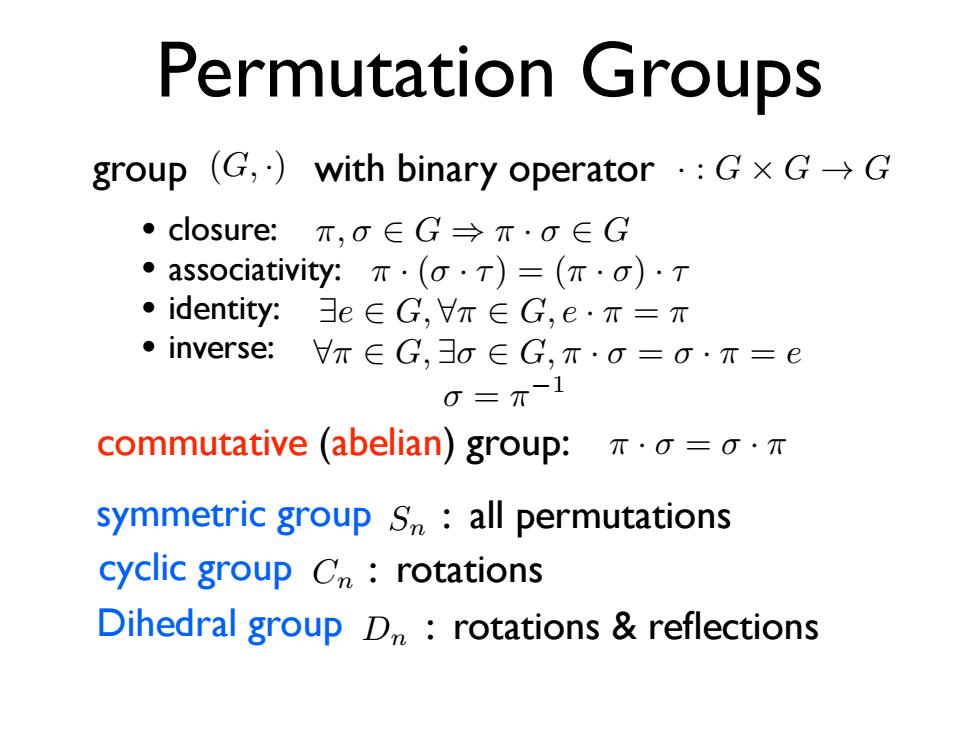
Permutation Groups group(G,)with binary operator·:G×G→G ·closure:π,o∈G→π·o∈G ·associativity::π·(o·T)=(π·o)·T 。identity:e∈G,Vπ∈G,e·T=π 。inverse:Vπ∈G,o∈G,π·o=o·π=e 0=π-1 commutative (abelian)group: π·0=0·π symmetric group S.:all permutations cyclic group Cn:rotations Dihedral group D:rotations reflectionsPermutation Groups group (G, ·) with binary operator · : G ⇥ G ! G • closure: • associativity: • identity: • inverse: ⇡, 2 G ) ⇡ · 2 G ⇡ · ( · ⌧ )=(⇡ · ) · ⌧ = ⇡1 8⇡ 2 G, 9 2 G, ⇡ · = · ⇡ = e 9e 2 G, 8⇡ 2 G, e · ⇡ = ⇡ commutative (abelian) group: ⇡ · = · ⇡ symmetric group cyclic group Dihedral group Sn Cn Dn : all permutations : rotations : rotations & reflections