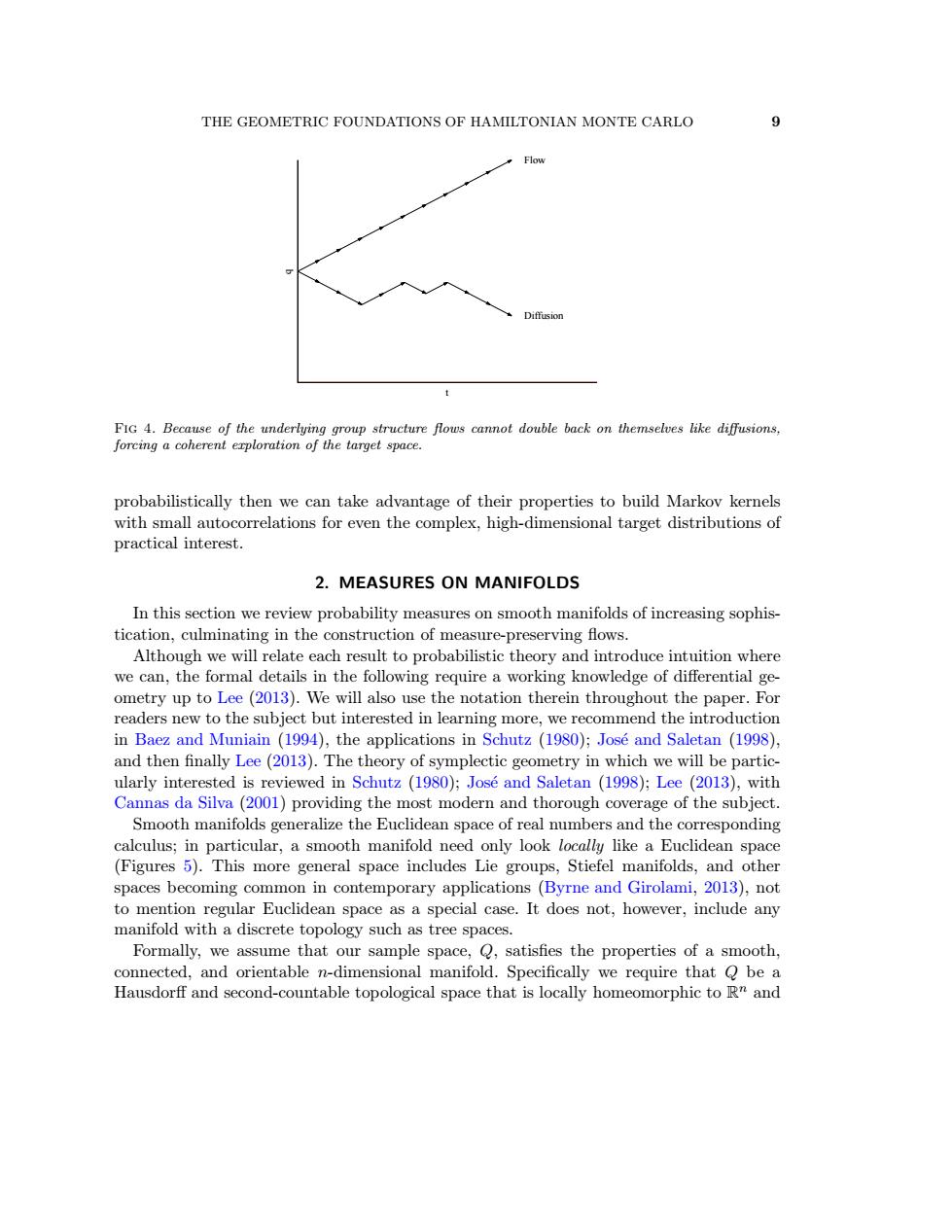
THE GEOMETRIC FOUNDATIONS OF HAMILTONIAN MONTE CARLO 9 Flow Diffusio cdbe eck on themce ueo probabilistically then we can take advantage of their properties to build Markov kernels with small autocorrelations for even the complex,high-dimensional target distributions of practical interest. 2.MEASURES ON MANIFOLDS In this section epreserving flows the foll uce int ferent etry up to e(2 e will also ion therein through the pa er.Fo rs nev in lear Bac and Muniain 1994 applications in Schutz(1980);J nd Saletan (1998 and then finally Lee(2013).The theory of symp ctic geometry in which we will be partic d Saletan (1998);Lee (2 13 with aing the most moc I thorou n coverage e of the su Smooth manifolds generalize the Euclidean space of real nd the corresponding calculus;in particular,a smooth manifold only look locally like a E clidea n space (Figures 5).This more general space includes Lie groups,Stiefel manifolds,and other spaces becoming common in contemporary applications (Byrne and Girolami,2013),not to mention regular Euclidean space as a special case.It does not,however,include any manifold with a discrete topology such as tree spaces. Formally,we assume that our sample space,satisfies the properties of a smooth, connected,and orientable n-dimensional manifold.Specifically we require that Q be a Hausdorff and second-countable topological space that is locally homeomorphic to R"and THE GEOMETRIC FOUNDATIONS OF HAMILTONIAN MONTE CARLO 9 q t Flow Diffusion Fig 4. Because of the underlying group structure flows cannot double back on themselves like diffusions, forcing a coherent exploration of the target space. probabilistically then we can take advantage of their properties to build Markov kernels with small autocorrelations for even the complex, high-dimensional target distributions of practical interest. 2. MEASURES ON MANIFOLDS In this section we review probability measures on smooth manifolds of increasing sophis￾tication, culminating in the construction of measure-preserving flows. Although we will relate each result to probabilistic theory and introduce intuition where we can, the formal details in the following require a working knowledge of differential ge￾ometry up to Lee (2013). We will also use the notation therein throughout the paper. For readers new to the subject but interested in learning more, we recommend the introduction in Baez and Muniain (1994), the applications in Schutz (1980); Jos´e and Saletan (1998), and then finally Lee (2013). The theory of symplectic geometry in which we will be partic￾ularly interested is reviewed in Schutz (1980); Jos´e and Saletan (1998); Lee (2013), with Cannas da Silva (2001) providing the most modern and thorough coverage of the subject. Smooth manifolds generalize the Euclidean space of real numbers and the corresponding calculus; in particular, a smooth manifold need only look locally like a Euclidean space (Figures 5). This more general space includes Lie groups, Stiefel manifolds, and other spaces becoming common in contemporary applications (Byrne and Girolami, 2013), not to mention regular Euclidean space as a special case. It does not, however, include any manifold with a discrete topology such as tree spaces. Formally, we assume that our sample space, Q, satisfies the properties of a smooth, connected, and orientable n-dimensional manifold. Specifically we require that Q be a Hausdorff and second-countable topological space that is locally homeomorphic to R n and