正在加载图片...
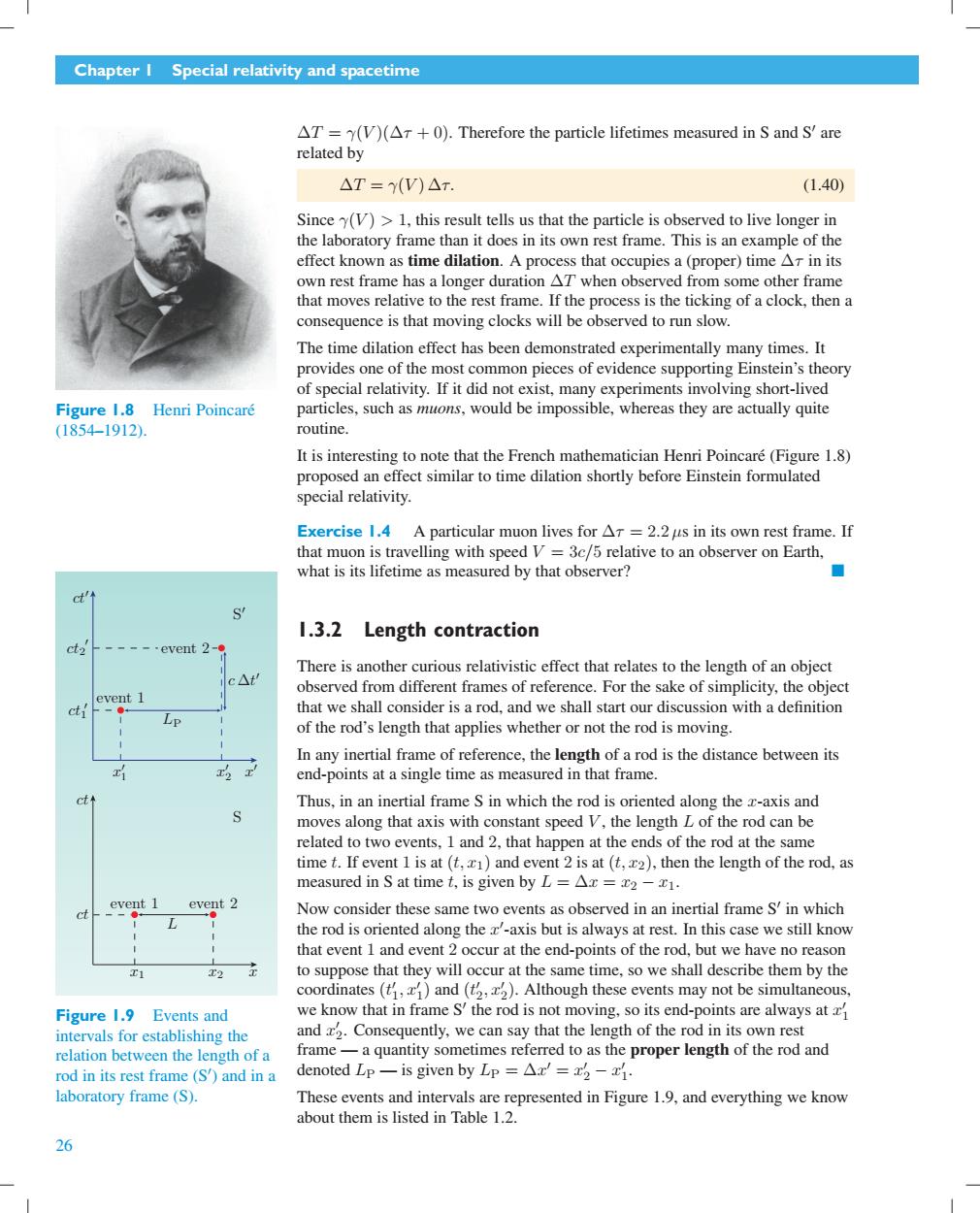
Chapter I Special relativity and spacetime AT=(V)(Ar+0).Therefore the particle lifetimes measured in S and S'are related by △T=Y(V)△r (1.40) Since (V)>1,this result tells us that the particle is observed to live longer in the laboratory frame than it does in its own rest frame.This is an example of the effect known as time dilation.A process that occupies a (proper)time Ar in its own rest frame has a longer duration AT when observed from some other frame that moves relative to the rest frame.If the process is the ticking of a clock,then a consequence is that moving clocks will be observed to run slow. The time dilation effect has been demonstrated experimentally many times.It provides one of the most common pieces of evidence supporting Einstein's theory of special relativity.If it did not exist,many experiments involving short-lived Figure 1.8 Henri Poincare particles,such as muons,would be impossible,whereas they are actually quite (18541912). routine. It is interesting to note that the French mathematician Henri Poincare(Figure 1.8) proposed an effect similar to time dilation shortly before Einstein formulated special relativity. Exercise 1.4 A particular muon lives for Ar =2.2 us in its own rest frame.If that muon is travelling with speed V=3c/5 relative to an observer on Earth, what is its lifetime as measured by that observer? ■ 1.3.2 Length contraction ct2 ----event2-。 There is another curious relativistic effect that relates to the length of an object c△t' observed from different frames of reference.For the sake of simplicity,the object event 1 cti that we shall consider is a rod,and we shall start our discussion with a definition Lp of the rod's length that applies whether or not the rod is moving. In any inertial frame of reference,the length of a rod is the distance between its T2 end-points at a single time as measured in that frame. ct Thus,in an inertial frame S in which the rod is oriented along the -axis and moves along that axis with constant speed V,the length L of the rod can be related to two events,1 and 2,that happen at the ends of the rod at the same time t.If event 1 is at(t,1)and event 2 is at (t,x2),then the length of the rod,as measured in S at time t,is given by L Ax x2-x1. event 1 event 2 ct Now consider these same two events as observed in an inertial frame S'in which ”一一指 the rod is oriented along the z'-axis but is always at rest.In this case we still know that event 1 and event 2 occur at the end-points of the rod,but we have no reason 1 T2 to suppose that they will occur at the same time,so we shall describe them by the coordinates (,and (t2,2).Although these events may not be simultaneous, Figure 1.9 Events and we know that in frame S'the rod is not moving,so its end-points are always at intervals for establishing the and x2.Consequently,we can say that the length of the rod in its own rest relation between the length of a frame-a quantity sometimes referred to as the proper length of the rod and rod in its rest frame(S')and in a denoted Lp一is given by Lp=△x'=z2-ti laboratory frame(S). These events and intervals are represented in Figure 1.9,and everything we know about them is listed in Table 1.2. 26Chapter 1 Special relativity and spacetime ΔT = γ(V )(Δτ + 0). Therefore the particle lifetimes measured in S and S % are related by ΔT = γ(V ) Δτ. (1.40) Since γ(V ) > 1, this result tells us that the particle is observed to live longer in the laboratory frame than it does in its own rest frame. This is an example of the effect known as time dilation. A process that occupies a (proper) time Δτ in its own rest frame has a longer duration ΔT when observed from some other frame that moves relative to the rest frame. If the process is the ticking of a clock, then a consequence is that moving clocks will be observed to run slow. The time dilation effect has been demonstrated experimentally many times. It provides one of the most common pieces of evidence supporting Einstein’s theory of special relativity. If it did not exist, many experiments involving short-lived particles, such as muons, would be impossible, whereas they are actually quite routine. Figure 1.8 Henri Poincare´ (1854–1912). It is interesting to note that the French mathematician Henri Poincare ( ´ Figure 1.8) proposed an effect similar to time dilation shortly before Einstein formulated special relativity. Exercise 1.4 A particular muon lives for Δτ = 2.2 µs in its own rest frame. If that muon is travelling with speed V = 3c/5 relative to an observer on Earth, what is its lifetime as measured by that observer? ■ 1.3.2 Length contraction There is another curious relativistic effect that relates to the length of an object observed from different frames of reference. For the sake of simplicity, the object that we shall consider is a rod, and we shall start our discussion with a definition of the rod’s length that applies whether or not the rod is moving. In any inertial frame of reference, the length of a rod is the distance between its end-points atasingle time as measured in that frame. Thus, in an inertial frame S in which the rod is oriented along the x-axis and moves along that axis with constant speed V , the length L of the rod can be related to two events, 1 and 2, that happen at the ends of the rod at the same time t. If event 1 is at (t, x1) and event 2 is at (t, x2), then the length of the rod, as measured in S at time t, is given by L = Δx = x2 − x1. Now consider these same two events as observed in an inertial frame S % in which the rod is oriented along the x % -axis but is always at rest. In this case we still know that event 1 and event 2 occur at the end-points of the rod, but we have no reason to suppose that they will occur at the same time, so we shall describe them by the coordinates (t % 1 , x% 1 ) and (t % 2 , x% 2 ). Although these events may not be simultaneous, we know that in frame S % the rod is not moving, so its end-points are always at x % 1 and x % 2 . Consequently, we can say that the length of the rod in its own rest frame —aquantity sometimes referred to as the proper length of the rod and denoted LP — is given by LP = Δx % = x % 2 − x % 1 . These events and intervals are represented in Figure 1.9, and everything we know about them is listed in Table 1.2. x " 2 x1 x2 ct ct S S " x " ct" x c Δt " event 1 event 2 event 1 event 2 L LP ct2 ct1 " ct" x " 11 Figure 1.9 Events and intervals for establishing the relation between the length of a rod in its rest frame (S% ) and in a laboratory frame (S). 26