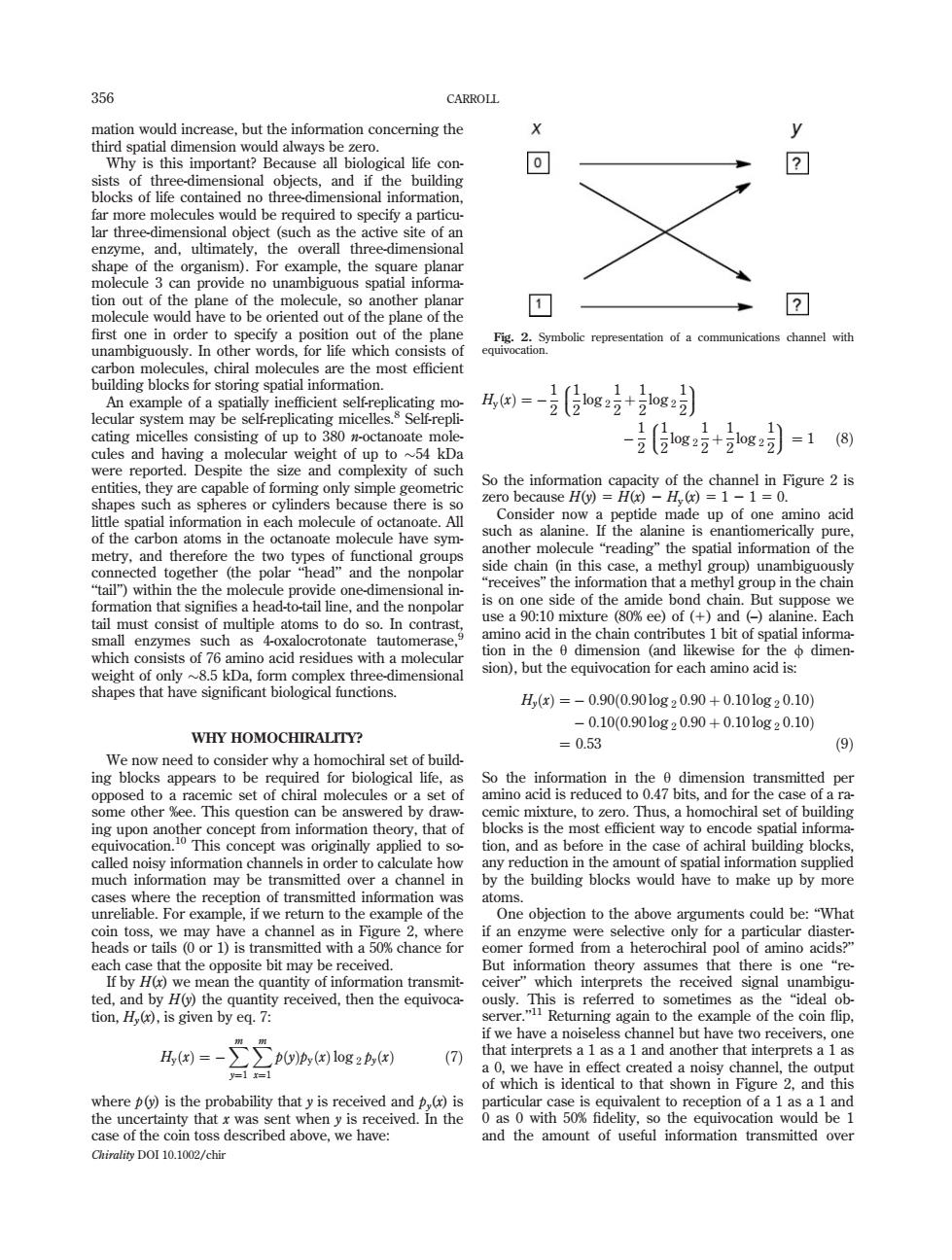
356 CARROLL con 回 and f te building ld be required to specify a part and.ultimatel For example, first one in order to ecify a sition out of the repre tion of a chiral mole th HG)og+log2 up to -[g吃+g】=1图 cause there is ow Setinmet6pofomeaminonacd another molecule reading the spatial information of the metry.and ail)withi the the nsional in is on de of the tail must consist of In cont 9010 acid in the chain contrib enzymes tauto three-dimensional om)-but the chn。 H,(x)=-0.90(0.901og20.90+0.101og20.10) -0.10(0.901 20.90+0.101og20.10 WHY HOMOCHIRALITY 053 (9 ing b sed toap r of chiral e other .This question noth can be This con tion,and as or re in the case of cases where to the abov ents could be:"Wha tawe may wh )is t for if an enzyme were se tive for ach that theo site bit may be But informat ion her mes an th that there is one th sly.Thi d to ming ag gain to H)=- (7) that interprets a l as a 1 and anothe af0wwhaedecedgremtedanoisync中anne2hea the rece tion of a I as ase of the coin toss described above,we have: and the amount of useful information transmitted over Chirality DOI 10.1002/chir mation would increase, but the information concerning the third spatial dimension would always be zero. Why is this important? Because all biological life con￾sists of three-dimensional objects, and if the building blocks of life contained no three-dimensional information, far more molecules would be required to specify a particu￾lar three-dimensional object (such as the active site of an enzyme, and, ultimately, the overall three-dimensional shape of the organism). For example, the square planar molecule 3 can provide no unambiguous spatial informa￾tion out of the plane of the molecule, so another planar molecule would have to be oriented out of the plane of the first one in order to specify a position out of the plane unambiguously. In other words, for life which consists of carbon molecules, chiral molecules are the most efficient building blocks for storing spatial information. An example of a spatially inefficient self-replicating mo￾lecular system may be self-replicating micelles.8 Self-repli￾cating micelles consisting of up to 380 n-octanoate mole￾cules and having a molecular weight of up to 54 kDa were reported. Despite the size and complexity of such entities, they are capable of forming only simple geometric shapes such as spheres or cylinders because there is so little spatial information in each molecule of octanoate. All of the carbon atoms in the octanoate molecule have sym￾metry, and therefore the two types of functional groups connected together (the polar ‘‘head’’ and the nonpolar ‘‘tail’’) within the the molecule provide one-dimensional in￾formation that signifies a head-to-tail line, and the nonpolar tail must consist of multiple atoms to do so. In contrast, small enzymes such as 4-oxalocrotonate tautomerase,9 which consists of 76 amino acid residues with a molecular weight of only 8.5 kDa, form complex three-dimensional shapes that have significant biological functions. WHY HOMOCHIRALITY? We now need to consider why a homochiral set of build￾ing blocks appears to be required for biological life, as opposed to a racemic set of chiral molecules or a set of some other %ee. This question can be answered by draw￾ing upon another concept from information theory, that of equivocation.10 This concept was originally applied to so￾called noisy information channels in order to calculate how much information may be transmitted over a channel in cases where the reception of transmitted information was unreliable. For example, if we return to the example of the coin toss, we may have a channel as in Figure 2, where heads or tails (0 or 1) is transmitted with a 50% chance for each case that the opposite bit may be received. If by H(x) we mean the quantity of information transmit￾ted, and by H(y) the quantity received, then the equivoca￾tion, Hy(x), is given by eq. 7: HyðxÞ¼
Xm y¼1 Xm x¼1 pðyÞpyðxÞlog 2 pyðxÞ ð7Þ where p(y) is the probability that y is received and py(x) is the uncertainty that x was sent when y is received. In the case of the coin toss described above, we have: HyðxÞ¼
1 2 1 2 log 2 1 2 þ 1 2 log 2 1 2 8 >: 9 >;
1 2 1 2 log 2 1 2 þ 1 2 log 2 1 2 8 >: 9 >; ¼ 1 ð8Þ So the information capacity of the channel in Figure 2 is zero because H(y) 5 H(x) 2 Hy(x) 5 1 2 1 5 0. Consider now a peptide made up of one amino acid such as alanine. If the alanine is enantiomerically pure, another molecule ‘‘reading’’ the spatial information of the side chain (in this case, a methyl group) unambiguously ‘‘receives’’ the information that a methyl group in the chain is on one side of the amide bond chain. But suppose we use a 90:10 mixture (80% ee) of (1) and (–) alanine. Each amino acid in the chain contributes 1 bit of spatial informa￾tion in the y dimension (and likewise for the f dimen￾sion), but the equivocation for each amino acid is: HyðxÞ¼
0:90ð0:90 log 2 0:90 þ 0:10 log 2 0:10Þ
0:10ð0:90 log 2 0:90 þ 0:10 log 2 0:10Þ ¼ 0:53 ð9Þ So the information in the y dimension transmitted per amino acid is reduced to 0.47 bits, and for the case of a ra￾cemic mixture, to zero. Thus, a homochiral set of building blocks is the most efficient way to encode spatial informa￾tion, and as before in the case of achiral building blocks, any reduction in the amount of spatial information supplied by the building blocks would have to make up by more atoms. One objection to the above arguments could be: ‘‘What if an enzyme were selective only for a particular diaster￾eomer formed from a heterochiral pool of amino acids?’’ But information theory assumes that there is one ‘‘re￾ceiver’’ which interprets the received signal unambigu￾ously. This is referred to sometimes as the ‘‘ideal ob￾server.’’11 Returning again to the example of the coin flip, if we have a noiseless channel but have two receivers, one that interprets a 1 as a 1 and another that interprets a 1 as a 0, we have in effect created a noisy channel, the output of which is identical to that shown in Figure 2, and this particular case is equivalent to reception of a 1 as a 1 and 0 as 0 with 50% fidelity, so the equivocation would be 1 and the amount of useful information transmitted over Fig. 2. Symbolic representation of a communications channel with equivocation. 356 CARROLL Chirality DOI 10.1002/chir