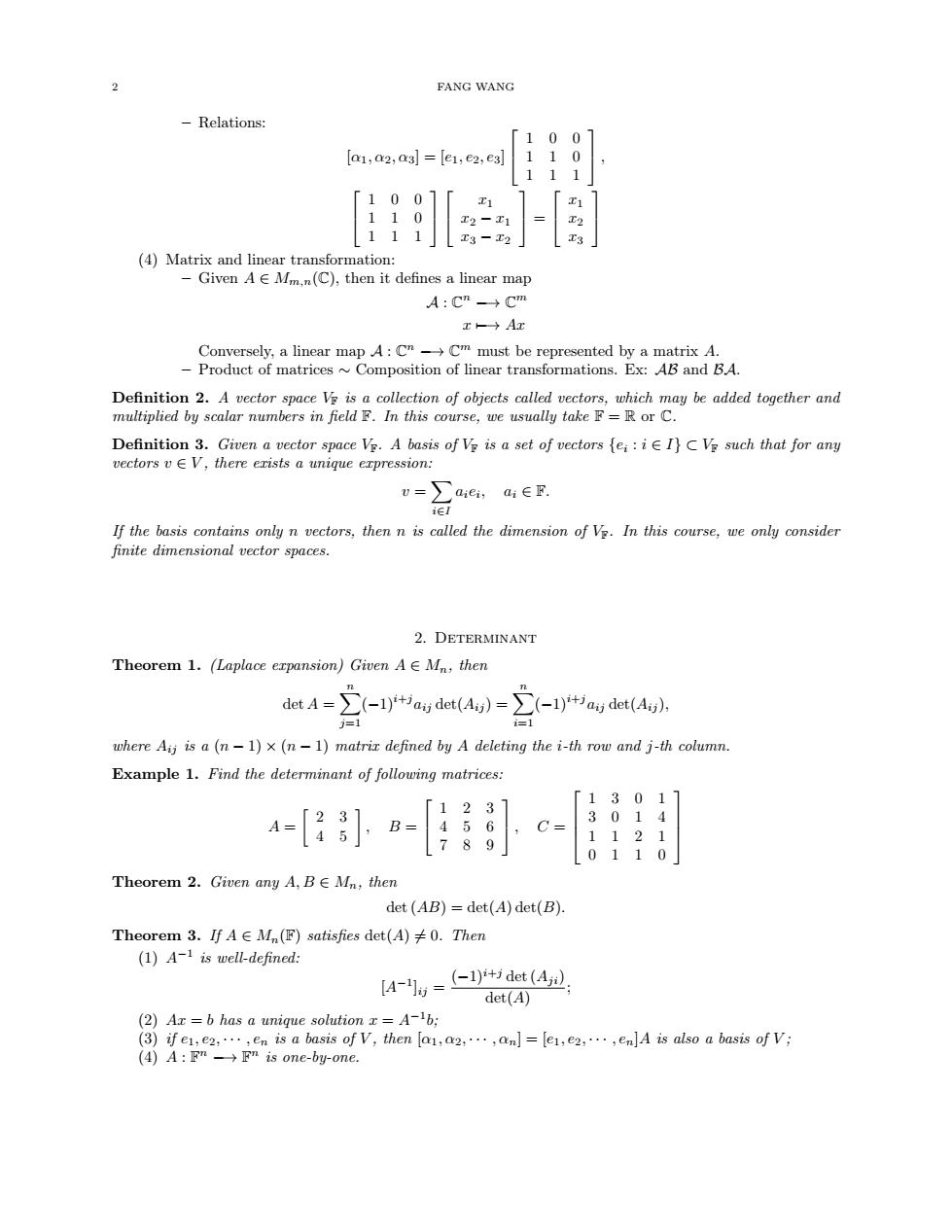
2 PANG WANG Relations: 「1001 a1,a2,agl=[e1,e2,e]110 111 [- 111g- (4)Matrix and linear transformation: -Given AM(C),then it defines a linear map A:Cm-→Cm IAr pm子m片o管2长rod n=∑ae,aieE If the basis contains only n vectors,then n is called the dimension of Ve.In this course,we only consider finite dimensional vector spaces. 2.DETERMINANT Theorem 1.(Laplace erpansion)Given AE Mn,then det A= C（-lr+ade(4）=∑-i)det(Al where Aj is a(n-1)x(n-1)matrir defined by A deleting the i-th row and j-th column Example 1.Find the determinant of following matrices: 3894 Theorem 2.Given any A,BE Mn,then det(AB)=det(A)det(B). Thec rem3.fAeL.(图stisfies det(≠0.hem (1)A-1 is well-defined A-hw=-1)det(4 det(A) (d):.2 FANG WANG – Relations: [α1, α2, α3] = [e1, e2, e3]   1 0 0 1 1 0 1 1 1   ,   1 0 0 1 1 0 1 1 1     x1 x2 − x1 x3 − x2   =   x1 x2 x3   (4) Matrix and linear transformation: – Given A ∈ Mm,n(C), then it defines a linear map A : C n −→ C m x 7−→ Ax Conversely, a linear map A : C n −→ C m must be represented by a matrix A. – Product of matrices ∼ Composition of linear transformations. Ex: AB and BA. Definition 2. A vector space VF is a collection of objects called vectors, which may be added together and multiplied by scalar numbers in field F. In this course, we usually take F = R or C. Definition 3. Given a vector space VF. A basis of VF is a set of vectors {ei : i ∈ I} ⊂ VF such that for any vectors v ∈ V , there exists a unique expression: v = X i∈I aiei , ai ∈ F. If the basis contains only n vectors, then n is called the dimension of VF. In this course, we only consider finite dimensional vector spaces. 2. Determinant Theorem 1. (Laplace expansion) Given A ∈ Mn, then det A = Xn j=1 (−1)i+j aij det(Aij ) = Xn i=1 (−1)i+j aij det(Aij ), where Aij is a (n − 1) × (n − 1) matrix defined by A deleting the i-th row and j-th column. Example 1. Find the determinant of following matrices: A =  2 3 4 5  , B =   1 2 3 4 5 6 7 8 9   , C =     1 3 0 1 3 0 1 4 1 1 2 1 0 1 1 0     Theorem 2. Given any A, B ∈ Mn, then det (AB) = det(A) det(B). Theorem 3. If A ∈ Mn(F) satisfies det(A) 6= 0. Then (1) A−1 is well-defined: [A −1 ]ij = (−1)i+j det (Aji) det(A) ; (2) Ax = b has a unique solution x = A−1 b; (3) if e1, e2, · · · , en is a basis of V , then [α1, α2, · · · , αn] = [e1, e2, · · · , en]A is also a basis of V ; (4) A : F n −→ F n is one-by-one