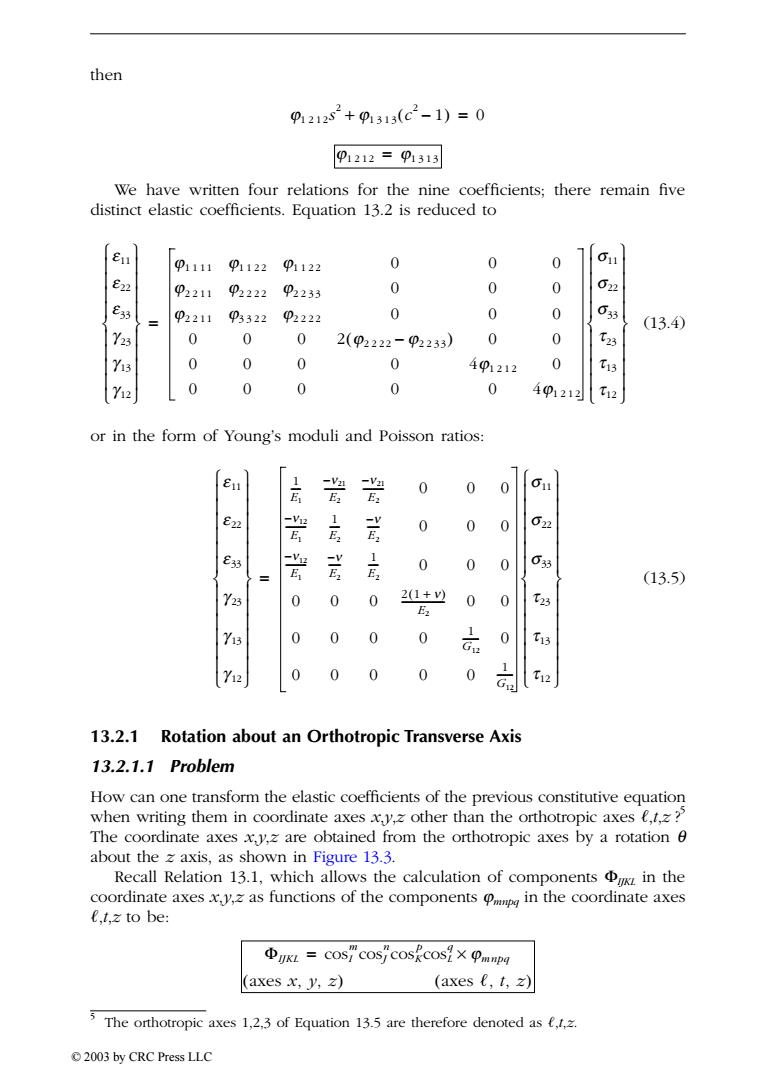
then p1212s2+p1313(c2-1)=0 p1212=91313 We have written four relations for the nine coefficients;there remain five distinct elastic coefficients.Equation 13.2 is reduced to En p1111p1122 p1122 0 0 0 611 e22 02211 φ2222 P2233 0 0 0 02 e33 02211 P3322 2222 0 0 0 65 (13.4) Y23 0 0 0 2(p2222-p2233) 0 0 (的 yis 0 0 0 0 401212 0 T13 Y12 0 0 0 0 0 401212 T12 or in the form of Young's moduli and Poisson ratios: 0 0 611 E e22 0 0 62 e3 E 言 0 0 633 (13.5) Y2s 0 0 0 2(1+2 0 0 T23 Y13 0 0 0 0 T13 Y12 0 0 0 0 G T12 13.2.1 Rotation about an Orthotropic Transverse Axis 13.2.1.1 Problem How can one transform the elastic coefficients of the previous constitutive equation when writing them in coordinate axes,z other than the orthotropic axes ,,z The coordinate axes x,z are obtained from the orthotropic axes by a rotation 0 about the z axis,as shown in Figure 13.3. Recall Relation 13.1,which allows the calculation of components Puk in the coordinate axesx,z as functions of the componentsp in the coordinate axes e,t,z to be: ΦL=Cos"coS”coscos1×pmp9 (axes x,y,z) (axes t,t,z) 5 The orthotropic axes 1,2.3 of Equation 13.5 are therefore denoted as ., 2003 by CRC Press LLCthen We have written four relations for the nine coefficients; there remain five distinct elastic coefficients. Equation 13.2 is reduced to (13.4) or in the form of Young’s moduli and Poisson ratios: (13.5) 13.2.1 Rotation about an Orthotropic Transverse Axis 13.2.1.1 Problem How can one transform the elastic coefficients of the previous constitutive equation when writing them in coordinate axes x,y,z other than the orthotropic axes
,t,z ? 5 The coordinate axes x,y,z are obtained from the orthotropic axes by a rotation q about the z axis, as shown in Figure 13.3. Recall Relation 13.1, which allows the calculation of components FIJKL in the coordinate axes x,y,z as functions of the components jmnpq in the coordinate axes
,t,z to be: 5 The orthotropic axes 1,2,3 of Equation 13.5 are therefore denoted as
,t,z. j1212s 2 j1313 c 2 + ( ) – 1 = 0 j1212 = j1313 e 11 e 22 e 33 g 23 g 13 g 12 Ó ˛ Ô Ô Ô Ô Ô Ô Ô Ô Ì ˝ Ô Ô Ô Ô Ô Ô Ô Ô Ï ¸ j1111 j1122 j1122 0 00 j2211 j2222 j2233 0 00 j2211 j3322 j2222 0 00 0 0 02 j2222 – j2233 ( ) 0 0 000 0 4j1212 0 0 0 0 0 04j1212 s11 s22 s33 t 23 t 13 t 12 Ó ˛ Ô Ô Ô Ô Ô Ô Ô Ô Ì ˝ Ô Ô Ô Ô Ô Ô Ô Ô Ï ¸ = e 11 e 22 e 33 g 23 g 13 g 12 Ó ˛ Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ì ˝ Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ï ¸ 1 E1 ---- –n21 E2 --------- –n21 E2 --------- 0 00 –n12 E1 --------- 1 E2 ---- –n E2 ----- 0 00 –n12 E1 --------- –n E2 ----- 1 E2 ---- 0 00 000 2 1( ) + n E2 -------------------- 0 0 000 0 1 G12 ------- 0 000 0 0 1 G12 ------- s11 s22 s33 t 23 t 13 t 12 Ó ˛ Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ì ˝ Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ï ¸ = FIJKL cosI mcosJ n cosK p cosL q = ¥ jmnpq ( ) axes x, y, z ( ) axes
, t, z TX846_Frame_C13 Page 264 Monday, November 18, 2002 12:29 PM © 2003 by CRC Press LLC