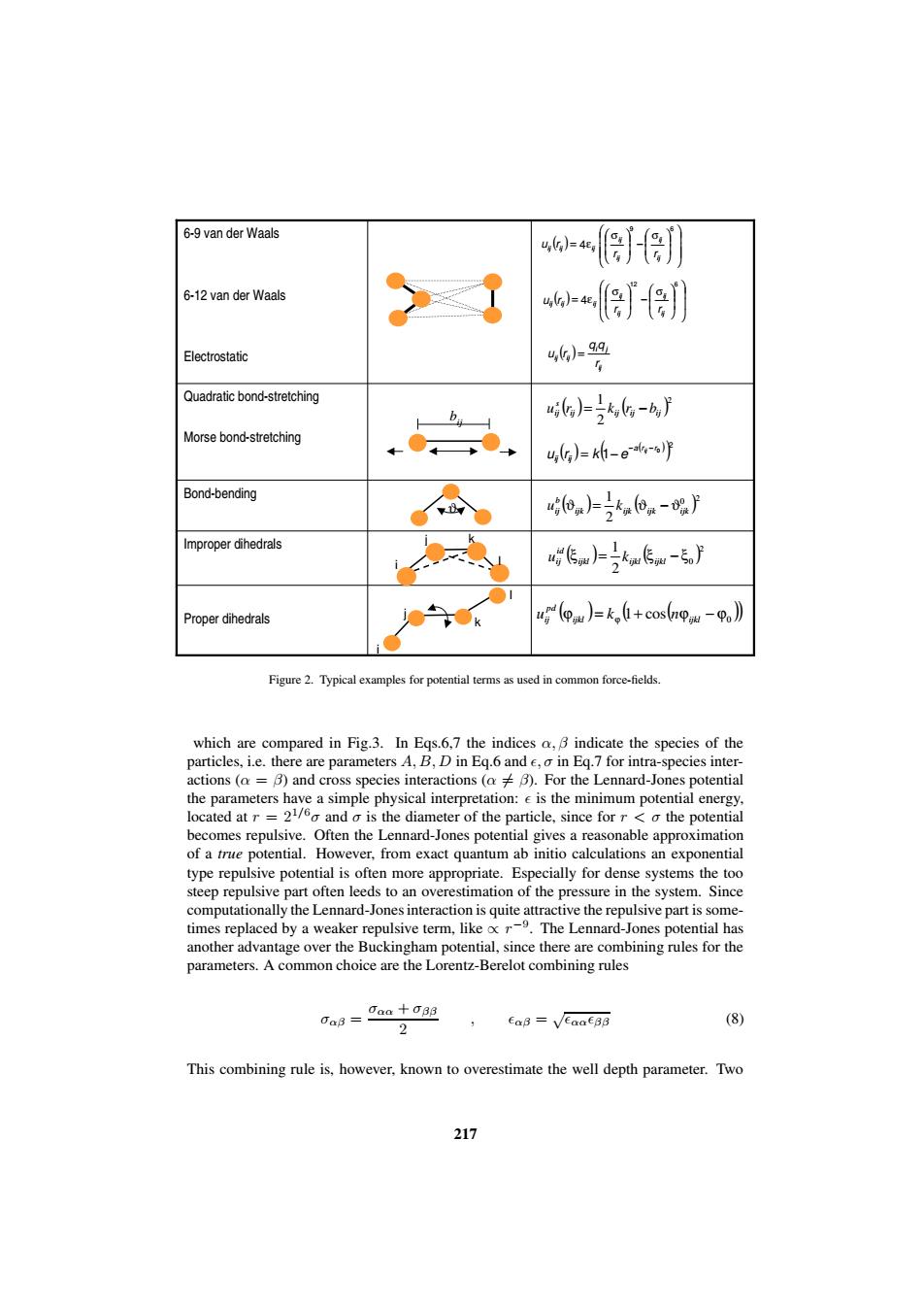
6-9 van der Waals -e{e 6-12 van der Waals 6- Electrostatic 4)=99 Quadratic bond-stretching 6)上6-6 Morse bond-stretching u,g)=kh-e-明 Bond-bending 4e,)上®.a-喝 Improper dihedrals ea上naw-d Proper dihedrals )=k (+cos(n) Figure 2.Typical examples for potential terms as used in common force-fields. which are compared in Fig.3.In Eqs.6.7 the indices a,B indicate the species of the particles,i.e.there are parameters A,B,D in Eq.6 and e,o in Eq.7 for intra-species inter- actions (a =B)and cross species interactions (aB).For the Lennard-Jones potential the parameters have a simple physical interpretation:e is the minimum potential energy, located at r=21/6 and o is the diameter of the particle,since forr<o the potential becomes repulsive.Often the Lennard-Jones potential gives a reasonable approximation of a true potential.However,from exact quantum ab initio calculations an exponential type repulsive potential is often more appropriate.Especially for dense systems the too steep repulsive part often leeds to an overestimation of the pressure in the system.Since computationally the Lennard-Jones interaction is quite attractive the repulsive part is some- times replaced by a weaker repulsive term,like r-9.The Lennard-Jones potential has another advantage over the Buckingham potential,since there are combining rules for the parameters.A common choice are the Lorentz-Berelot combining rules 0aB=Jaa +aga 2 EaB VEaaEBB (8) This combining rule is,however,known to overestimate the well depth parameter.Two 217Proper dihedrals Improper dihedrals Bond-bending Quadratic bond-stretching Morse bond-stretching 6-9 van der Waals 6-12 van der Waals Electrostatic ( )                   σ −         σ = ε 9 6 4 ij ij ij ij ij ij ij r r u r ( )                 σ −         σ = ε 12 6 4 ij ij ij ij ij ij ij r r u r ( ) ij i j ij ij r q q u r = bij ( ) ( ) 2 2 1 ij ij ij ij s uij r = k r − b ( ) ( ) ( ) 2 0 1 a r r ij ij ij u r k e − − = − ϑ ( ) ( ) 2 0 2 1 ijk ijk ijk ijk b ij u ϑ = k ϑ − ϑ i j k l ( ) ( ) 2 0 2 1 ξijkl = ijkl ξijkl − ξ id uij k i j k l ( ) ( ( )) 0 ϕijkl = ϕ 1+ cos ϕijkl − ϕ pd uij k n Figure 2. Typical examples for potential terms as used in common force-fields. which are compared in Fig.3. In Eqs.6,7 the indices α, β indicate the species of the particles, i.e. there are parameters A, B, D in Eq.6 and , σ in Eq.7 for intra-species inter￾actions (α = β) and cross species interactions (α 6= β). For the Lennard-Jones potential the parameters have a simple physical interpretation:  is the minimum potential energy, located at r = 2 1/6σ and σ is the diameter of the particle, since for r < σ the potential becomes repulsive. Often the Lennard-Jones potential gives a reasonable approximation of a true potential. However, from exact quantum ab initio calculations an exponential type repulsive potential is often more appropriate. Especially for dense systems the too steep repulsive part often leeds to an overestimation of the pressure in the system. Since computationally the Lennard-Jonesinteraction is quite attractive the repulsive part is some￾times replaced by a weaker repulsive term, like ∝ r −9 . The Lennard-Jones potential has another advantage over the Buckingham potential, since there are combining rules for the parameters. A common choice are the Lorentz-Berelot combining rules σαβ = σαα + σββ 2 , αβ = √ ααββ (8) This combining rule is, however, known to overestimate the well depth parameter. Two 217