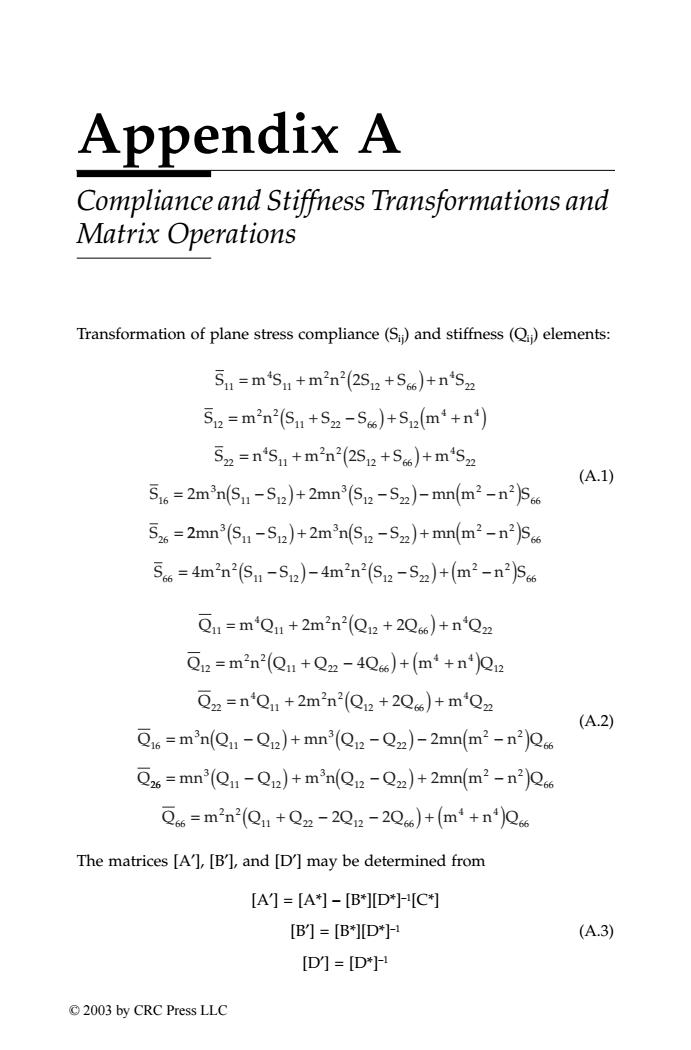
Appendix A Compliance and Stiffness Transformations and Matrix Operations Transformation of plane stress compliance(S)and stiffness(Q)elements: 51:=mts1+m2n2(2s2+S6)+nts2 52=m2n2(Su+Sz-S)+S2(m+n) 52=ns1+m2n2(2S2+S6)+m4S22 (A.1) 56=2m3n(51:-Sz)+2mn3(52-S2a)-mn(m2-n256 52=2mn(Sn-S2)+2mn(S2-S2)+mn(m2-n2)Sc 56=4m2n251-S2)-4m2n2(52-s2z)+(m2-n2）56 Q1=m*Q1+2m2n2(Q2+206)+nQ2 02=m2n2(Q1+Q2-4Q6)+(m+n)Q2 z=nQu+2mn2(Qn2+2Qc)+mQz (A.2) Q=m'n(Q-Q2)+mn(Q2-Qz)-2mn(m2-n2)Qc z=mn(Q-Q2)+mn(Q2-Qz)+2mn(m2)6 Q6=m2n2(Q1+Q2-2Q12-20s)+(m+n)0s The matrices [A],[B'],and [D']may be determined from [A]=[A]-[B*][D*-[C] [B]=[B][D*H (A.3) [D]=[D*H ©2003 by CRC Press LLCAppendix A Compliance and Stiffness Transformations and Matrix Operations Transformation of plane stress compliance (Sij) and stiffness (Qij) elements: (A.1) (A.2) The matrices [A′], [B′], and [D′] may be determined from [A′] = [A*] – [B*][D*]–1[C*] [B′] = [B*][D*]–1 (A.3) [D′] = [D*]–1 S =m S m n S S n S S mn S S S S m n S nS mn S S mS S m n S S mn S S mn m n S S 11 4 11 2 2 12 66 4 22 12 2 2 11 22 66 12 4 4 22 4 11 2 2 12 66 4 22 16 3 11 12 3 12 22 2 2 66 26 2 2 2 2 + + ( ) + = +− ( ) + + ( ) =+ + ( ) + = − ( ) + − ( ) − − ( ) = 2 2 4 4 3 11 12 3 12 22 2 2 66 66 2 2 11 12 2 2 12 22 2 2 66 mn S S m n S S mn m n S S mn S S mn S S m n S ( ) − + − ( ) + − ( ) = − ( ) − − ( ) + − ( ) Q =m Q m n Q Q n Q Q mn Q Q Q m n Q Q nQ mn Q Q mQ Q m n Q Q mn Q Q mn m n Q Q 11 4 11 2 2 12 66 4 22 12 2 2 11 22 66 4 4 12 22 4 11 2 2 12 66 4 22 16 3 11 12 3 12 22 2 2 66 2 2 4 2 2 2 + + ( ) + = +− ( ) + + ( ) =+ + ( ) + = − ( ) + − ( ) − − ( ) 26 3 11 12 3 12 22 2 2 66 66 2 2 11 22 12 66 4 4 66 2 2 2 = − ( ) + − ( ) + − ( ) = +− − ( ) + + ( ) mn Q Q m n Q Q mn m n Q Q mn Q Q Q Q m n Q TX001_AppA_Frame Page 213 Saturday, September 21, 2002 5:11 AM © 2003 by CRC Press LLC