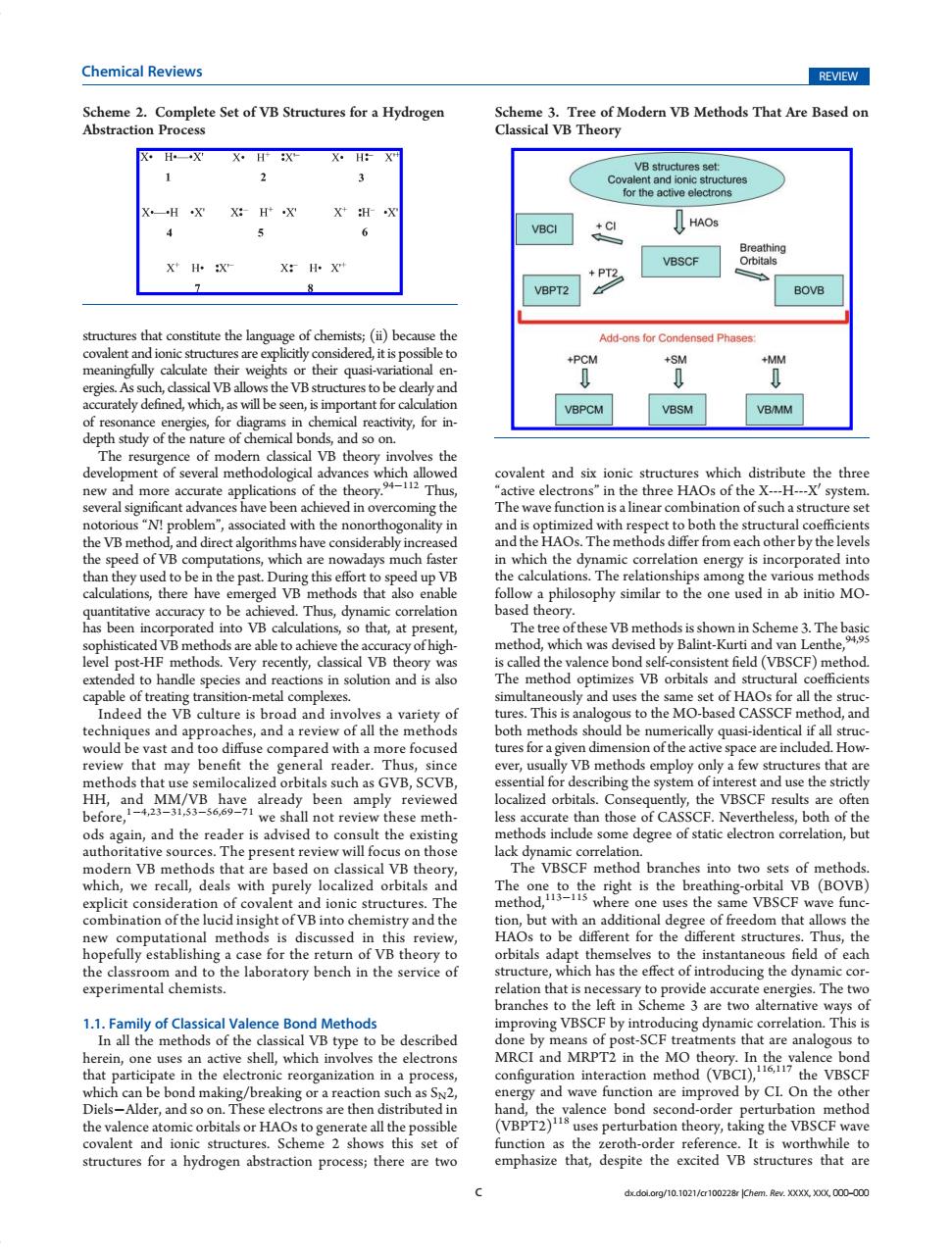
Chemical Reviews REVIEW Scheme 2.Complete Set of VB Structures for a Hydrogen Scheme 3.Tree of Modern VB Methods That Are Based on Abstraction Process Classical VB Theory X· 日一X X·H产X X·HX VB structures set: Covalent and ionic structures for the active electrons XHX' X:HX X H X 5 6 VBCI +CI HAOS Breathing VBSCF Orbitals X'H.:X X:H·X PT2 VBPT2 BOVB structures that constitute the language of chemists;(ii)because the Add-ons for Condensed Phases covalent and ionic structures are explicitly considered,it is possible to +PCM +SM +MM meaningfully calculate their weights or their quasi-variational en- ergies.As such,classical VB allows the VB structures to be dearly and accurately defined,which,as will be seen,is important for calculation VBPCM VBSM VB/MM of resonance energies,for diagrams in chemical reactivity,for in- depth study of the nature of chemical bonds,and so on. The resurgence of modern classical VB theory involves the development of several methodological advances which allowed covalent and six ionic structures which distribute the three new and more accurate applications of the theoryThus, "active electrons"in the three HAOs of the X---H---X'system. several significant advances have been achieved in overcoming the The wave function is a linear combination of such a structure set notorious"N!problem",associated with the nonorthogonality in and is optimized with respect to both the structural coefficients the VB method,and direct algorithms have considerably increased and the HAOs.The methods differ from each other by the levels the speed of VB computations,which are nowadays much faster in which the dynamic correlation energy is incorporated into than they used to be in the past.During this effort to speed up VB the calculations.The relationships among the various methods calculations,there have emerged VB methods that also enable follow a philosophy similar to the one used in ab initio MO- quantitative accuracy to be achieved.Thus,dynamic correlation based theory. has been incorporated into VB calculations,so that,at present, The tree ofthese VB methods is shown in Scheme 3.The basic sophisticated VB methods are able to achieve the accuracy of high- method,which was devised by Balint-Kurti and van Lenthe 94s level post-HF methods.Very recently,classical VB theory was is called the valence bond self-consistent field(VBSCF)method. extended to handle species and reactions in solution and is also The method optimizes VB orbitals and structural coefficients capable of treating transition-metal complexes. simultaneously and uses the same set of HAOs for all the struc- Indeed the VB culture is broad and involves a variety of tures.This is analogous to the MO-based CASSCF method,and techniques and approaches,and a review of all the methods both methods should be numerically quasi-identical if all struc- would be vast and too diffuse compared with a more focused tures for a given dimension of the active space are included.How- review that may benefit the general reader.Thus,since ever,usually VB methods employ only a few structures that are methods that use semilocalized orbitals such as GVB,SCVB essential for describing the system of interest and use the strictly HH,and MM/VB have already been amply reviewed localized orbitals.Consequently,the VBSCF results are often before,1-4,23-31,53-56,69-71 we shall not review these meth- less accurate than those of CASSCF.Nevertheless,both of the ods again,and the reader is advised to consult the existing methods include some degree of static electron correlation,but authoritative sources.The present review will focus on those lack dynamic correlation. modern VB methods that are based on classical VB theory, The VBSCF method branches into two sets of methods which,we recall,deals with purely localized orbitals and The one to the right is the breathing-orbital VB (BOVB) explicit consideration of covalent and ionic structures.The method,1 where one uses the same VBSCF wave func- combination of the lucid insight of VB into chemistry and the tion,but with an additional degree of freedom that allows the new computational methods is discussed in this review, HAOs to be different for the different structures.Thus,the hopefully establishing a case for the return of VB theory to orbitals adapt themselves to the instantaneous field of each the classroom and to the laboratory bench in the service of structure,which has the effect of introducing the dynamic cor- experimental chemists. relation that is necessary to provide accurate energies.The two branches to the left in Scheme 3 are two alternative ways of 1.1.Family of Classical Valence Bond Methods improving VBSCF by introducing dynamic correlation.This is In all the methods of the classical VB type to be described done by means of post-SCF treatments that are analogous to herein,one uses an active shell,which involves the electrons MRCI and MRPT2 in the MO theory.In the valence bond that participate in the electronic reorganization in a process, configuration interaction method (VBCI),7 the VBSCE which can be bond making/breaking or a reaction such as SN2, energy and wave function are improved by CI.On the other Diels-Alder,and so on.These electrons are then distributed in hand,the valence bond second-order perturbation method the valence atomic orbitals or HAOs to generate all the possible (VBPT2)118 uses perturbation theory,taking the VBSCF wave covalent and ionic structures.Scheme 2 shows this set of function as the zeroth-order reference.It is worthwhile to structures for a hydrogen abstraction process;there are two emphasize that,despite the excited VB structures that are dx.dol.org/10.1021/cr100228r Chem.Rev.XXXX,XXX,000-000C dx.doi.org/10.1021/cr100228r |Chem. Rev. XXXX, XXX, 000–000 Chemical Reviews REVIEW structures that constitute the language of chemists; (ii) because the covalent and ionic structures are explicitly considered, it is possible to meaningfully calculate their weights or their quasi-variational en￾ergies. As such, classical VB allows the VB structures to be clearly and accurately defined, which, as will be seen, is important for calculation of resonance energies, for diagrams in chemical reactivity, for in￾depth study of the nature of chemical bonds, and so on. The resurgence of modern classical VB theory involves the development of several methodological advances which allowed new and more accurate applications of the theory.94
112 Thus, several significant advances have been achieved in overcoming the notorious “N! problem”, associated with the nonorthogonality in the VB method, and direct algorithms have considerably increased the speed of VB computations, which are nowadays much faster than they used to be in the past. During this effort to speed up VB calculations, there have emerged VB methods that also enable quantitative accuracy to be achieved. Thus, dynamic correlation has been incorporated into VB calculations, so that, at present, sophisticated VB methods are able to achieve the accuracy of high￾level post-HF methods. Very recently, classical VB theory was extended to handle species and reactions in solution and is also capable of treating transition-metal complexes. Indeed the VB culture is broad and involves a variety of techniques and approaches, and a review of all the methods would be vast and too diffuse compared with a more focused review that may benefit the general reader. Thus, since methods that use semilocalized orbitals such as GVB, SCVB, HH, and MM/VB have already been amply reviewed before,1
4,23
31,53
56,69
71 we shall not review these meth￾ods again, and the reader is advised to consult the existing authoritative sources. The present review will focus on those modern VB methods that are based on classical VB theory, which, we recall, deals with purely localized orbitals and explicit consideration of covalent and ionic structures. The combination of the lucid insight of VB into chemistry and the new computational methods is discussed in this review, hopefully establishing a case for the return of VB theory to the classroom and to the laboratory bench in the service of experimental chemists. 1.1. Family of Classical Valence Bond Methods In all the methods of the classical VB type to be described herein, one uses an active shell, which involves the electrons that participate in the electronic reorganization in a process, which can be bond making/breaking or a reaction such as SN2, Diels
Alder, and so on. These electrons are then distributed in the valence atomic orbitals or HAOs to generate all the possible covalent and ionic structures. Scheme 2 shows this set of structures for a hydrogen abstraction process; there are two covalent and six ionic structures which distribute the three “active electrons” in the three HAOs of the X---H---X0 system. The wave function is a linear combination of such a structure set and is optimized with respect to both the structural coefficients and the HAOs. The methods differ from each other by the levels in which the dynamic correlation energy is incorporated into the calculations. The relationships among the various methods follow a philosophy similar to the one used in ab initio MO￾based theory. The tree of these VB methods is shown in Scheme 3. The basic method, which was devised by Balint-Kurti and van Lenthe,94,95 is called the valence bond self-consistent field (VBSCF) method. The method optimizes VB orbitals and structural coefficients simultaneously and uses the same set of HAOs for all the struc￾tures. This is analogous to the MO-based CASSCF method, and both methods should be numerically quasi-identical if all struc￾tures for a given dimension of the active space are included. How￾ever, usually VB methods employ only a few structures that are essential for describing the system of interest and use the strictly localized orbitals. Consequently, the VBSCF results are often less accurate than those of CASSCF. Nevertheless, both of the methods include some degree of static electron correlation, but lack dynamic correlation. The VBSCF method branches into two sets of methods. The one to the right is the breathing-orbital VB (BOVB) method,113
115 where one uses the same VBSCF wave func￾tion, but with an additional degree of freedom that allows the HAOs to be different for the different structures. Thus, the orbitals adapt themselves to the instantaneous field of each structure, which has the effect of introducing the dynamic cor￾relation that is necessary to provide accurate energies. The two branches to the left in Scheme 3 are two alternative ways of improving VBSCF by introducing dynamic correlation. This is done by means of post-SCF treatments that are analogous to MRCI and MRPT2 in the MO theory. In the valence bond configuration interaction method (VBCI),116,117 the VBSCF energy and wave function are improved by CI. On the other hand, the valence bond second-order perturbation method (VBPT2)118 uses perturbation theory, taking the VBSCF wave function as the zeroth-order reference. It is worthwhile to emphasize that, despite the excited VB structures that are Scheme 2. Complete Set of VB Structures for a Hydrogen Abstraction Process Scheme 3. Tree of Modern VB Methods That Are Based on Classical VB Theory