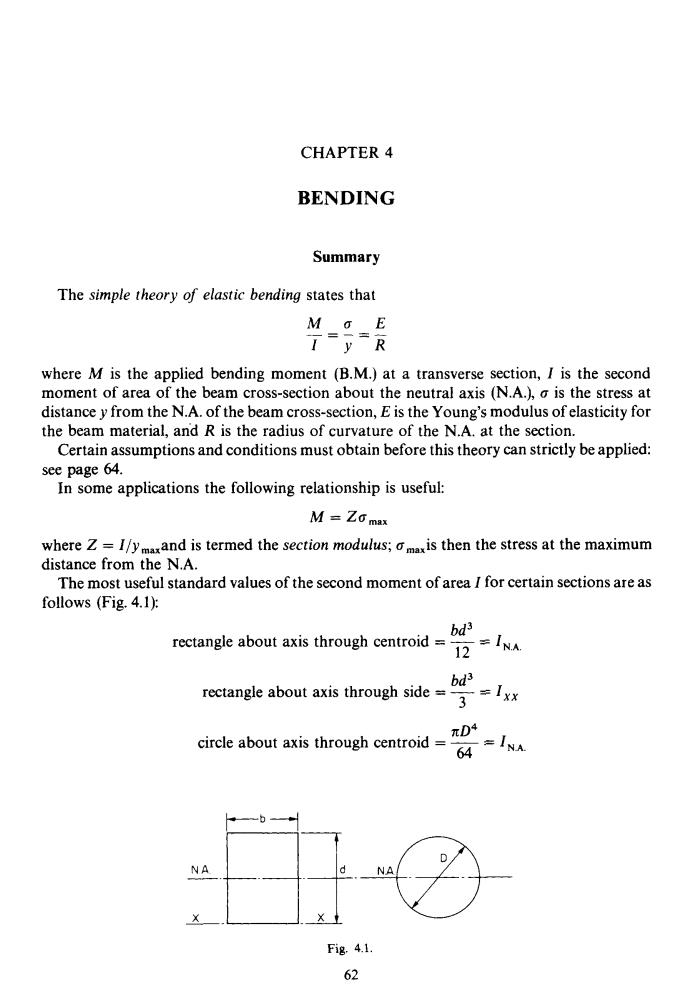
CHAPTER 4 BENDING Summary The simple theory of elastic bending states that M G E T-y-R where M is the applied bending moment(B.M.)at a transverse section,I is the second moment of area of the beam cross-section about the neutral axis(N.A.),o is the stress at distance y from the N.A.of the beam cross-section,E is the Young's modulus of elasticity for the beam material,and R is the radius of curvature of the N.A.at the section. Certain assumptions and conditions must obtain before this theory can strictly be applied: see page 64. In some applications the following relationship is useful: M=Zo max where Z=I/ymaxand is termed the section modulus;omaxis then the stress at the maximum distance from the N.A. The most useful standard values of the second moment of area for certain sections are as follows(Fig.4.1片 bd3 rectangle about axis through centroid 2=14 b rectangle about axis through side 3=1xx circle about axis throun 64 =INA NA NA X Fig.4.1. 62CHAPTER 4 BENDING Summary The simple theory of elastic bending states that MaE _- _- _- IYR where M is the applied bending moment (B.M.) at a transverse section, I is the second moment of area of the beam cross-section about the neutral axis (N.A.), 0 is the stress at distance y from the N.A. of the beam cross-section, E is the Young’s modulus of elasticity for the beam material, and R is the radius of curvature of the N.A. at the section. Certain assumptions and conditions must obtain before this theory can strictly be applied: see page 64. In some applications the following relationship is useful: M = Zomax where Z = Z/y,,,and is termed the section modulus; amaxis then the stress at the maximum distance from the N.A. The most useful standard values of the second moment of area I for certain sections are as follows (Fig. 4.1): bd3 12 rectangle about axis through centroid = ~ = ZN,A, bd3 3 nD4 64 rectangle about axis through side = __ = I,, circle about axis through centroid = - = ZN,A, Fig. 4.1. 62