正在加载图片...
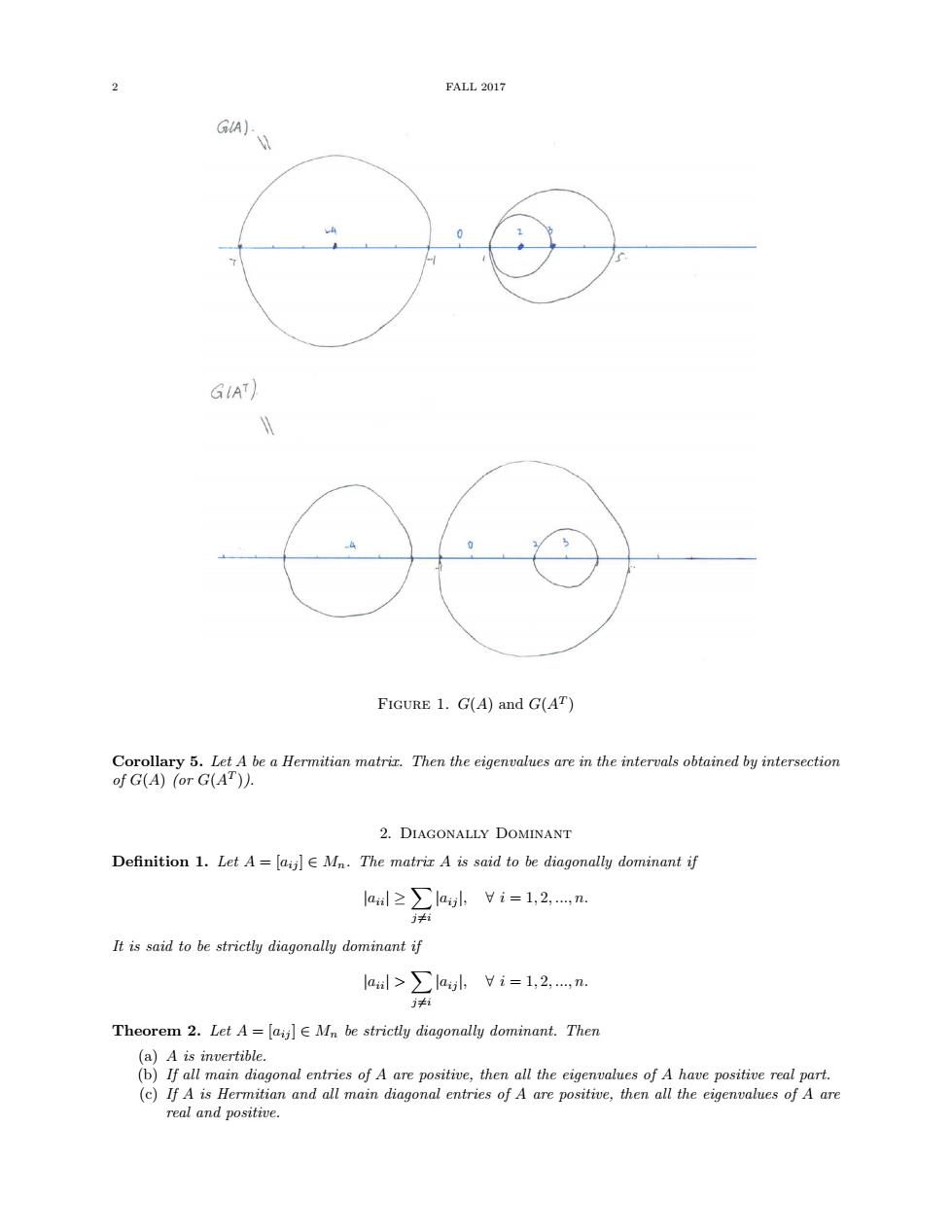
2 FALL 2017 GA) GIAT) FIGURE 1.G(A)and G(AT) Corollary 5.Let A be a Hermitian matrir.Then the eigenvalues are in the intervals obtained by intersection 时G(4)(or G(AT) 2.DIAGONALLY DOMINANT Definition 1.Let A=Mn.The matrir A is said to be diagonally dominantif al≥∑ali=1,2…,n It is said to be strictly diagonally dominant if laidl laigl,vi=1.2.....n. Theorem 2.Let A=aMn be strictly diagonally dominant.Then (a)A is invertible. (b)If all main dingonal entries of A are positive,then all the cigenvalues of A have positive real part. (c)If A is Hermitian and all main diagonal entries of A are positive,then all the eigenvalues of A are2 FALL 2017 Figure 1. G(A) and G(AT ) Corollary 5. Let A be a Hermitian matrix. Then the eigenvalues are in the intervals obtained by intersection of G(A) (or G(AT )). 2. Diagonally Dominant Definition 1. Let A = [aij ] ∈ Mn. The matrix A is said to be diagonally dominant if |aii| ≥ X j6=i |aij |, ∀ i = 1, 2, ..., n. It is said to be strictly diagonally dominant if |aii| > X j6=i |aij |, ∀ i = 1, 2, ..., n. Theorem 2. Let A = [aij ] ∈ Mn be strictly diagonally dominant. Then (a) A is invertible. (b) If all main diagonal entries of A are positive, then all the eigenvalues of A have positive real part. (c) If A is Hermitian and all main diagonal entries of A are positive, then all the eigenvalues of A are real and positive