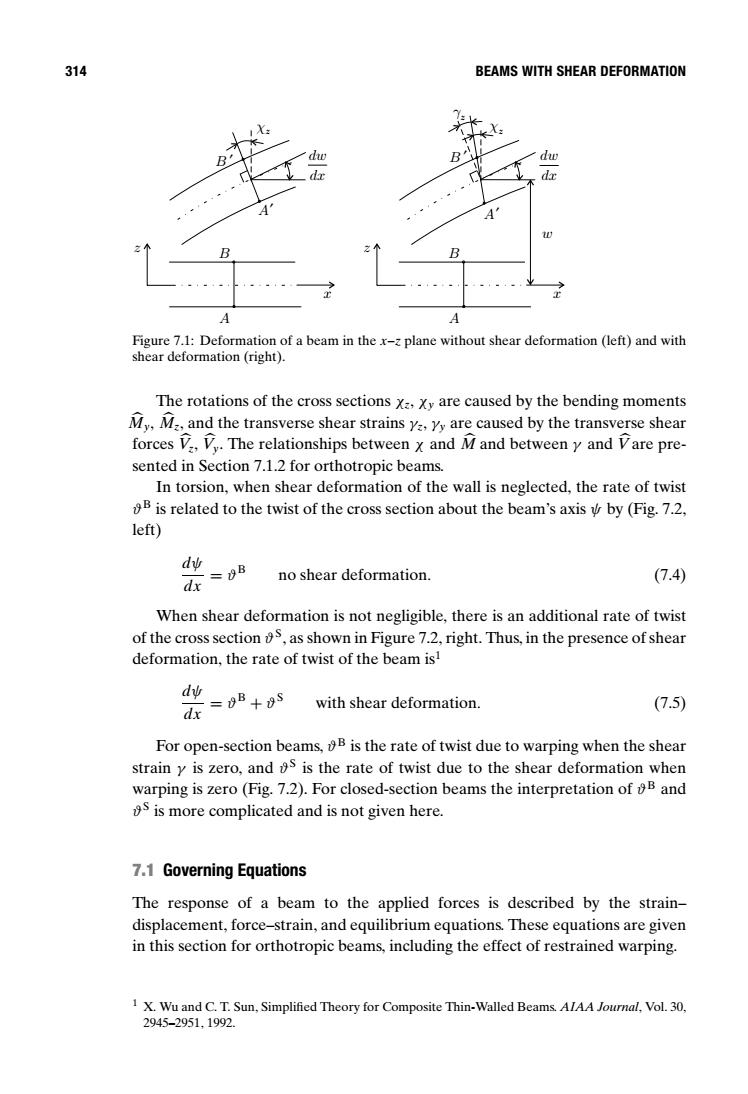
314 BEAMS WITH SHEAR DEFORMATION X主 dw dw B B y Figure 7.1:Deformation of a beam in the x-z plane without shear deformation(left)and with shear deformation(right). The rotations of the cross sections x=,xy are caused by the bending moments My,M,and the transverse shear strains y Yy are caused by the transverse shear forces V.,V.The relationships between x and M and betweeny and Vare pre- sented in Section 7.1.2 for orthotropic beams. In torsion,when shear deformation of the wall is neglected,the rate of twist B is related to the twist of the cross section about the beam's axis by(Fig.7.2, left) dψ dx =B no shear deformation. (7.4) When shear deformation is not negligible,there is an additional rate of twist of the cross section s,as shown in Figure 7.2,right.Thus,in the presence of shear deformation,the rate of twist of the beam is! d地=B+9s d with shear deformation (7.5) For open-section beams,B is the rate of twist due to warping when the shear strain y is zero,and s is the rate of twist due to the shear deformation when warping is zero(Fig.7.2).For closed-section beams the interpretation ofB and s is more complicated and is not given here. 7.1 Governing Equations The response of a beam to the applied forces is described by the strain- displacement,force-strain,and equilibrium equations.These equations are given in this section for orthotropic beams,including the effect of restrained warping. 1 X.Wu and C.T.Sun,Simplified Theory for Composite Thin-Walled Beams.AlAA Journal,Vol.30, 2945-2951,1992.314 BEAMS WITH SHEAR DEFORMATION w γz χz dx dw dx dw x z B A x z B A χz A′ A′ B ′ B ′ Figure 7.1: Deformation of a beam in the x–z plane without shear deformation (left) and with shear deformation (right). The rotations of the cross sections χz, χy are caused by the bending moments M
y, M
z, and the transverse shear strains γz, γy are caused by the transverse shear forces V
z, V
y. The relationships between χ and M
and between γ and V
are pre￾sented in Section 7.1.2 for orthotropic beams. In torsion, when shear deformation of the wall is neglected, the rate of twist ϑB is related to the twist of the cross section about the beam’s axis ψ by (Fig. 7.2, left) dψ dx = ϑB no shear deformation. (7.4) When shear deformation is not negligible, there is an additional rate of twist of the cross section ϑS, as shown in Figure 7.2, right. Thus, in the presence of shear deformation, the rate of twist of the beam is1 dψ dx = ϑB + ϑS with shear deformation. (7.5) For open-section beams, ϑB is the rate of twist due to warping when the shear strain γ is zero, and ϑS is the rate of twist due to the shear deformation when warping is zero (Fig. 7.2). For closed-section beams the interpretation of ϑB and ϑS is more complicated and is not given here. 7.1 Governing Equations The response of a beam to the applied forces is described by the strain– displacement, force–strain, and equilibrium equations. These equations are given in this section for orthotropic beams, including the effect of restrained warping. 1 X. Wu and C. T. Sun, Simplified Theory for Composite Thin-Walled Beams. AIAA Journal, Vol. 30, 2945–2951, 1992