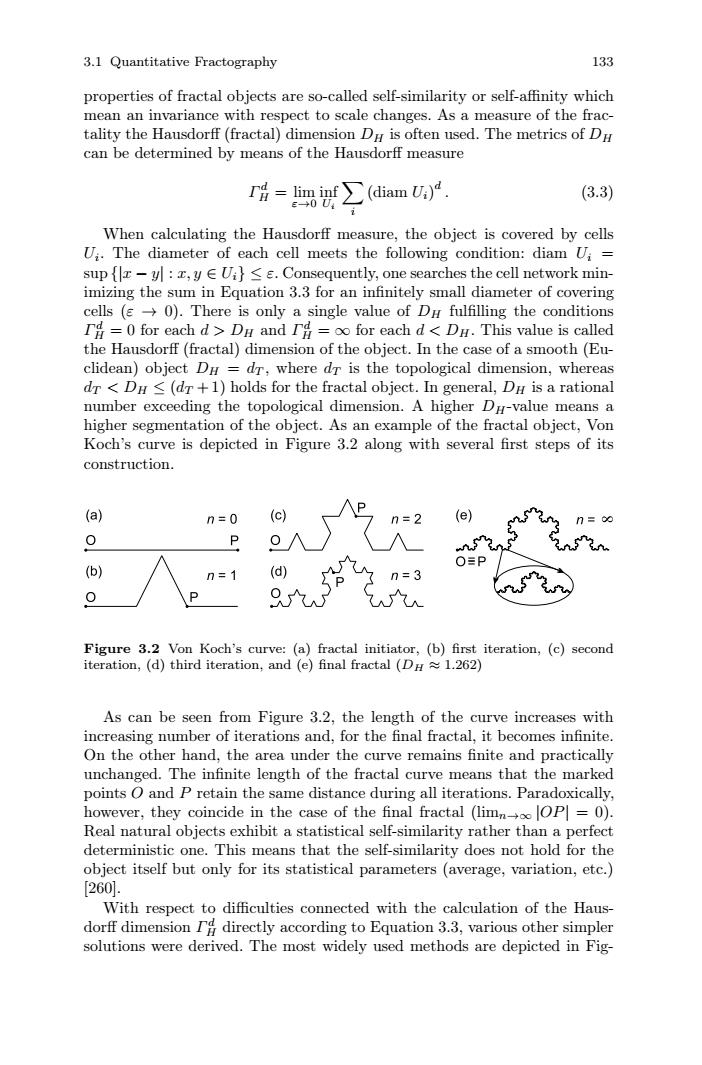
3.1 Quantitative Fractography 133 properties of fractal objects are so-called self-similarity or self-affinity which mean an invariance with respect to scale changes.As a measure of the frac- tality the Hausdorff(fractal)dimension Dy is often used.The metrics of D can be determined by means of the Hausdorff measure T哈=lmi#∑(diam U,)4. (3.3) E-00 When calculating the Hausdorff measure,the object is covered by cells Ui.The diameter of each cell meets the following condition:diam Ui= sup {-yl:,yEUi}<s.Consequently,one searches the cell network min- imizing the sum in Equation 3.3 for an infinitely small diameter of covering cells (->0).There is only a single value of D fulfilling the conditions T=0 for each d>D and Ta=oo for each d<DH.This value is called the Hausdorff(fractal)dimension of the object.In the case of a smooth(Eu- clidean)object D=dr,where dr is the topological dimension,whereas dr D<(dr+1)holds for the fractal object.In general,DH is a rational number exceeding the topological dimension.A higher Dy-value means a higher segmentation of the object.As an example of the fractal object,Von Koch's curve is depicted in Figure 3.2 along with several first steps of its construction. (a) n=0 n=2 (e） 7=∞ 0 P (b) n=1 (d) n=3 0 Figure 3.2 Von Koch's curve:(a)fractal initiator,(b)first iteration,(c)second iteration,(d)third iteration,and (e)final fractal(D1.262) As can be seen from Figure 3.2,the length of the curve increases with increasing number of iterations and,for the final fractal,it becomes infinite. On the other hand,the area under the curve remains finite and practically unchanged.The infinite length of the fractal curve means that the marked points O and P retain the same distance during all iterations.Paradoxically, however,they coincide in the case of the final fractal (limnOP=0). Real natural objects exhibit a statistical self-similarity rather than a perfect deterministic one.This means that the self-similarity does not hold for the object itself but only for its statistical parameters (average,variation,etc.) [2601. With respect to difficulties connected with the calculation of the Haus- dorff dimension I directly according to Equation 3.3,various other simpler solutions were derived.The most widely used methods are depicted in Fig-3.1 Quantitative Fractography 133 properties of fractal objects are so-called self-similarity or self-affinity which mean an invariance with respect to scale changes. As a measure of the frac￾tality the Hausdorff (fractal) dimension DH is often used. The metrics of DH can be determined by means of the Hausdorff measure Γd H = limε→0 inf Ui  i (diam Ui) d . (3.3) When calculating the Hausdorff measure, the object is covered by cells Ui. The diameter of each cell meets the following condition: diam Ui = sup {|x − y| : x, y ∈ Ui} ≤ ε. Consequently, one searches the cell network min￾imizing the sum in Equation 3.3 for an infinitely small diameter of covering cells (ε → 0). There is only a single value of DH fulfilling the conditions Γd H = 0 for each d>DH and Γd H = ∞ for each d<DH. This value is called the Hausdorff (fractal) dimension of the object. In the case of a smooth (Eu￾clidean) object DH = dT , where dT is the topological dimension, whereas dT < DH ≤ (dT + 1) holds for the fractal object. In general, DH is a rational number exceeding the topological dimension. A higher DH-value means a higher segmentation of the object. As an example of the fractal object, Von Koch’s curve is depicted in Figure 3.2 along with several first steps of its construction. Figure 3.2 Von Koch’s curve: (a) fractal initiator, (b) first iteration, (c) second iteration, (d) third iteration, and (e) final fractal (DH ≈ 1.262) As can be seen from Figure 3.2, the length of the curve increases with increasing number of iterations and, for the final fractal, it becomes infinite. On the other hand, the area under the curve remains finite and practically unchanged. The infinite length of the fractal curve means that the marked points O and P retain the same distance during all iterations. Paradoxically, however, they coincide in the case of the final fractal (limn→∞ |OP| = 0). Real natural objects exhibit a statistical self-similarity rather than a perfect deterministic one. This means that the self-similarity does not hold for the object itself but only for its statistical parameters (average, variation, etc.) [260]. With respect to difficulties connected with the calculation of the Haus￾dorff dimension Γd H directly according to Equation 3.3, various other simpler solutions were derived. The most widely used methods are depicted in Fig-