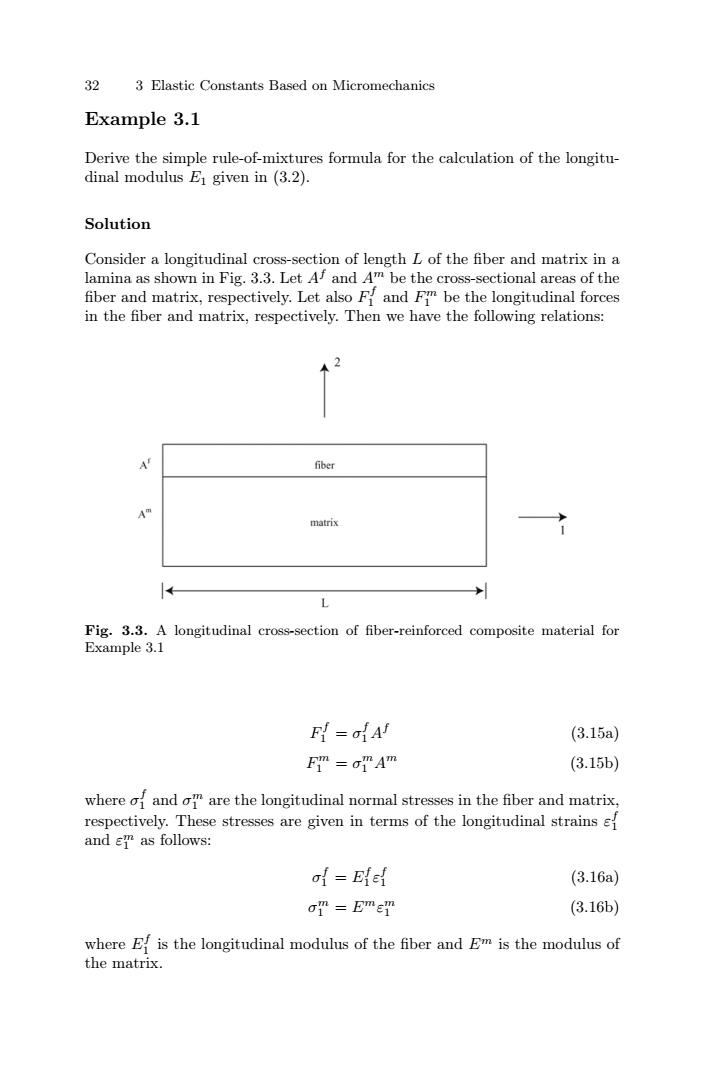
32 3 Elastic Constants Based on Micromechanics Example 3.1 Derive the simple rule-of-mixtures formula for the calculation of the longitu- dinal modulus E given in (3.2). Solution Consider a longitudinal cross-section of length L of the fiber and matrix in a lamina as shown in Fig.3.3.Let Af and Am be the cross-sectional areas of the fiber and matrix,respectively.Let also F and Fm be the longitudinal forces in the fiber and matrix,respectively.Then we have the following relations: fiber Am matrix L Fig.3.3.A longitudinal cross-section of fiber-reinforced composite material for Example 3.1 Ff=afA! (3.15a) Fi=om Am (3.15b) where of and o are the longitudinal normal stresses in the fiber and matrix, respectively.These stresses are given in terms of the longitudinal strainsf and em as follows: of=Efef (3.16a) am =Emem (3.16b) where Ef is the longitudinal modulus of the fiber and Em is the modulus of the matrix.32 3 Elastic Constants Based on Micromechanics Example 3.1 Derive the simple rule-of-mixtures formula for the calculation of the longitu￾dinal modulus E1 given in (3.2). Solution Consider a longitudinal cross-section of length L of the fiber and matrix in a lamina as shown in Fig. 3.3. Let Af and Am be the cross-sectional areas of the fiber and matrix, respectively. Let also Ff 1 and F m 1 be the longitudinal forces in the fiber and matrix, respectively. Then we have the following relations: Fig. 3.3. A longitudinal cross-section of fiber-reinforced composite material for Example 3.1 Ff 1 = σf 1 Af (3.15a) F m 1 = σm 1 Am (3.15b) where σf 1 and σm 1 are the longitudinal normal stresses in the fiber and matrix, respectively. These stresses are given in terms of the longitudinal strains ε f 1 and εm 1 as follows: σf 1 = Ef 1 ε f 1 (3.16a) σm 1 = Emεm 1 (3.16b) where Ef 1 is the longitudinal modulus of the fiber and Em is the modulus of the matrix