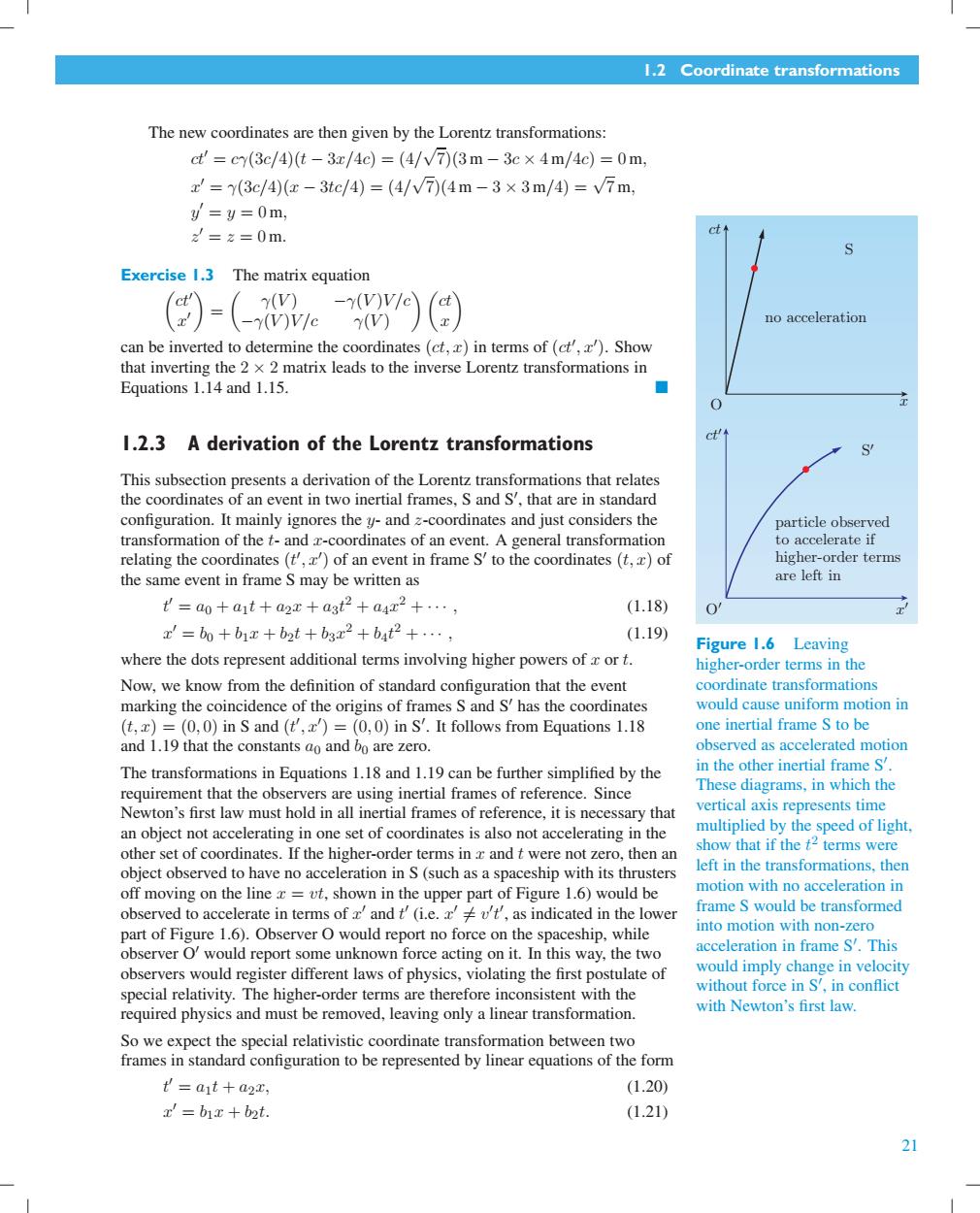
.2 Coordinate transformations The new coordinates are then given by the Lorentz transformations: ct'=cy(3c/4)t-3x/4c)=(4/W7)(3m-3c×4m/4c)=0m, x'=y(3c/4)(x-3tc/4)=(4/W7)(4m-3×3m/4)=V7m ==0m, =z=0m. ct Exercise 1.3 The matrix equation x= x(V) -(V)V/c ct Y(V)V/e Y() （x no acceleration can be inverted to determine the coordinates(ct,)in terms of(ct',)Show that inverting the 2 x 2 matrix leads to the inverse Lorentz transformations in Equations 1.14 and 1.15. ■ 1.2.3 A derivation of the Lorentz transformations ct This subsection presents a derivation of the Lorentz transformations that relates the coordinates of an event in two inertial frames,S and S',that are in standard configuration.It mainly ignores the y-and z-coordinates and just considers the particle observed transformation of the t-and x-coordinates of an event.A general transformation to accelerate if relating the coordinates (t',x')of an event in frame S'to the coordinates (t,x)of higher-order terms the same event in frame S may be written as are left in t=ao+at+azz+a3t2+ax2+.., (1.18) 0 =bo+b1x+b2t+b322+b4t2+.. (1.19) Figure 1.6 Leaving where the dots represent additional terms involving higher powers of x or t. higher-order terms in the Now,we know from the definition of standard configuration that the event coordinate transformations marking the coincidence of the origins of frames S and S'has the coordinates would cause uniform motion in (t,)=(0,0)in S and (t',')=(0,0)in S'.It follows from Equations 1.18 one inertial frame S to be and 1.19 that the constants ao and bo are zero. observed as accelerated motion The transformations in Equations 1.18 and 1.19 can be further simplified by the in the other inertial frame S'. requirement that the observers are using inertial frames of reference.Since These diagrams,in which the Newton's first law must hold in all inertial frames of reference,it is necessary that vertical axis represents time an object not accelerating in one set of coordinates is also not accelerating in the multiplied by the speed of light other set of coordinates.If the higher-order terms in x and t were not zero,then an show that if the t2 terms were object observed to have no acceleration in S(such as a spaceship with its thrusters left in the transformations.then off moving on the line c=vt,shown in the upper part of Figure 1.6)would be motion with no acceleration in observed to accelerate in terms of x'and t'(i.e.''t,as indicated in the lower frame S would be transformed part of Figure 1.6).Observer O would report no force on the spaceship,while into motion with non-zero observer O'would report some unknown force acting on it.In this way,the two acceleration in frame S'.This observers would register different laws of physics,violating the first postulate of would imply change in velocity special relativity.The higher-order terms are therefore inconsistent with the without force in S',in conflict required physics and must be removed,leaving only a linear transformation. with Newton's first law. So we expect the special relativistic coordinate transformation between two frames in standard configuration to be represented by linear equations of the form t'=at a2x, (1.20) x'=bix+b2t. (1.21) 211.2 Coordinate transformations The new coordinates are then given by the Lorentz transformations: ct% = cγ(3c/4)(t − 3x/4c) = (4/ √ 7)(3 m − 3c × 4 m/4c) = 0 m, x % = γ(3c/4)(x − 3tc/4) = (4/ √ 7)(4 m − 3 × 3 m/4) = √ 7 m, y % = y = 0 m, z % = z = 0 m. Exercise 1.3 The matrix equation ? ct% x % @ = ? γ(V ) −γ(V )V /c −γ(V )V /c γ(V ) @ ?ct x @ can be inverted to determine the coordinates (ct, x) in terms of (ct% , x% ). Show that inverting the 2 × 2 matrix leads to the inverse Lorentz transformations in Equations 1.14 and 1.15. ■ 1.2.3 A derivation of the Lorentz transformations This subsection presents a derivation of the Lorentz transformations that relates the coordinates of an event in two inertial frames, S and S % , that are in standard configuration. It mainly ignores the y- and z-coordinates and just considers the transformation of the t- and x-coordinates of an event. A general transformation relating the coordinates (t % , x% ) of an event in frame S % to the coordinates (t, x) of the same event in frame S may be written as t % = a0 + a1t + a2x + a3t 2 + a4x 2 + ··· , (1.18) x % = b0 + b1x + b2t + b3x 2 + b4t 2 + ··· , (1.19) where the dots represent additional terms involving higher powers of x or t. Now, we know from the definition of standard configuration that the event marking the coincidence of the origins of frames S and S% has the coordinates (t, x) = (0, 0) in S and (t % , x% )=(0, 0) in S % . It follows from Equations 1.18 and 1.19 that the constants a0 and b0 are zero. ct S S " x " ct" no acceleration x particle observed to accelerate if higher-order terms are left in O O" Figure 1.6 Leaving higher-order terms in the coordinate transformations would cause uniform motion in one inertial frame S to be observed as accelerated motion in the other inertial frame S % . These diagrams, in which the vertical axis represents time multiplied by the speed of light, show that if the t 2 terms were left in the transformations, then motion with no acceleration in frame S would be transformed into motion with non-zero acceleration in frame S % . This would imply change in velocity without force in S % , in conflict with Newton’s first law. The transformations in Equations 1.18 and 1.19 can be further simplified by the requirement that the observers are using inertial frames of reference. Since Newton’s first law must hold in all inertial frames of reference, it is necessary that an object not accelerating in one set of coordinates is also not accelerating in the other set of coordinates. If the higher-order terms in x and t were not zero, then an object observed to have no acceleration in S (such as a spaceship with its thrusters off moving on the line x = vt, shown in the upper part of Figure 1.6) would be observed to accelerate in terms of x % and t % (i.e. x % =4 v % t % , as indicated in the lower part of Figure 1.6). Observer O would report no force on the spaceship, while observer O% would report some unknown force acting on it. In this way, the two observers would register different laws of physics, violating the first postulate of special relativity. The higher-order terms are therefore inconsistent with the required physics and must be removed, leaving only a linear transformation. So we expect the special relativistic coordinate transformation between two frames in standard configuration to be represented by linear equations of the form t % = a1t + a2x, (1.20) x % = b1x + b2t. (1.21) 21