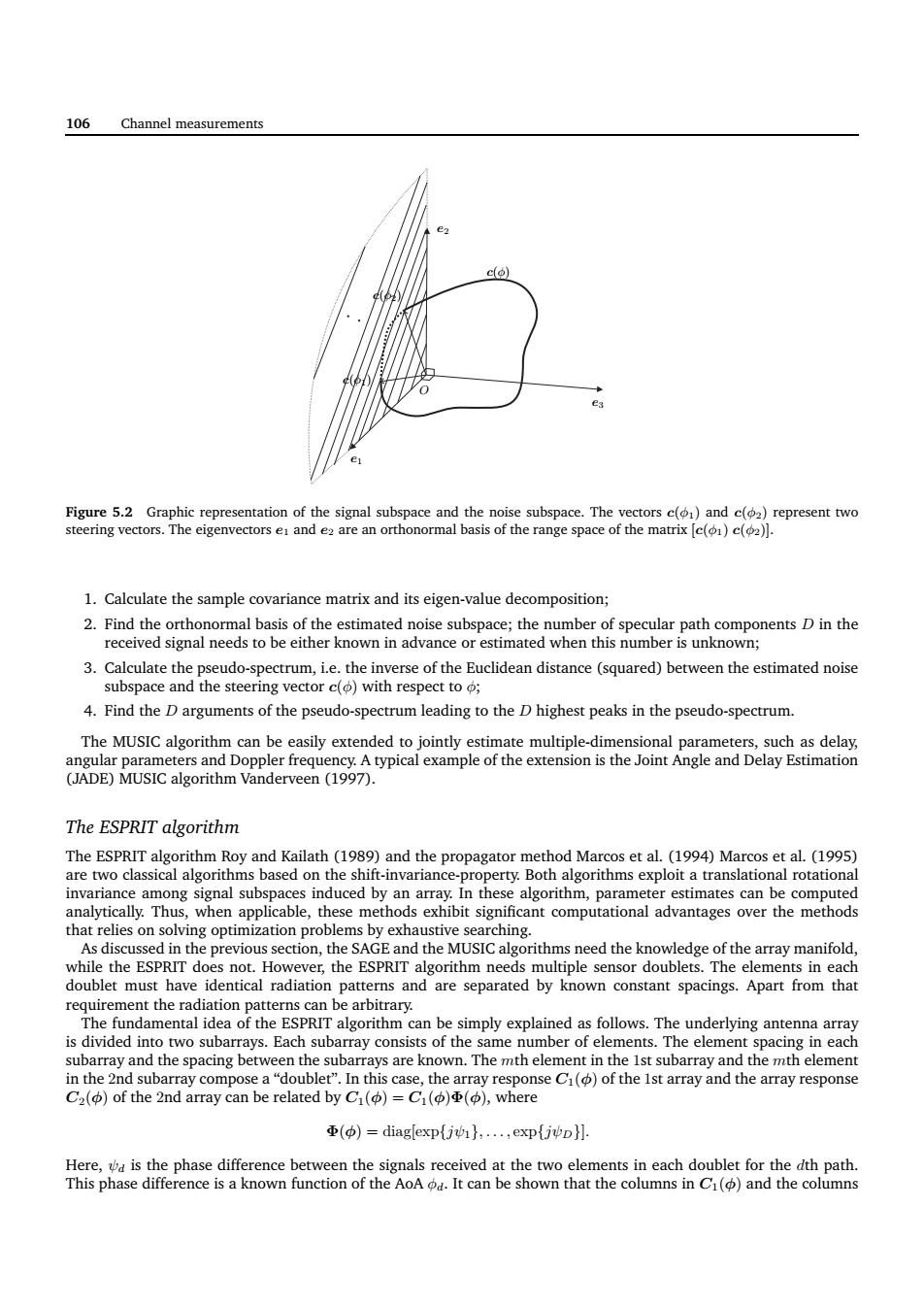
106 Channel measurements 1.Calculate the sample covariance matrix and its eigen-value decomposition: 2.Find the orthonormal basis of the estimated noise subspace;the number of specular path components D in the received signal needs to be either known in advance or estimated when this number is unknown; 3.Calculate the pseudo-spectrum,i.e.the inverse of the Euclidean distance (squared)between the estimated noise subspace and the steering vector c()with respect to; 4.Find the D arguments of the pseudo-spectrum leading to the D highest peaks in the pseudo-spectrum. (JADE)MUSIC algorithm Vanderveen (1997). The ESPRIT algorithm The ESPRIT algorithm Roy and Kailath(198)and the propagator method Marcos et al.(199)Marcos et al.(1995) are two classical basedn the shif-invariance- es on s ation pro while the ESPRIT does not.However,the ESPRIT algorithm needs multiple sensor doublets.The elements in eac doublet must have dentical radiation patters and are separated by known constant spacings.Apart from that rays.Each subarray consists of the ame number of elements.The elemen pacing in eac in the 2nd subarray composeadoubler"In this case.thea response C)of the ist array and the array response C2(中)of the2 nd array can be related by C1(中)=C(中)Φ(p),where ()diaglexp(jvi}.....exp(jp 106 Channel measurements e1 e2 e3 c(φ1) c(φ2) c(φ) O Figure 5.2 Graphic representation of the signal subspace and the noise subspace. The vectors c(φ1) and c(φ2) represent two steering vectors. The eigenvectors e1 and e2 are an orthonormal basis of the range space of the matrix [c(φ1) c(φ2)]. 1. Calculate the sample covariance matrix and its eigen-value decomposition; 2. Find the orthonormal basis of the estimated noise subspace; the number of specular path components D in the received signal needs to be either known in advance or estimated when this number is unknown; 3. Calculate the pseudo-spectrum, i.e. the inverse of the Euclidean distance (squared) between the estimated noise subspace and the steering vector c(φ) with respect to φ; 4. Find the D arguments of the pseudo-spectrum leading to the D highest peaks in the pseudo-spectrum. The MUSIC algorithm can be easily extended to jointly estimate multiple-dimensional parameters, such as delay, angular parameters and Doppler frequency. A typical example of the extension is the Joint Angle and Delay Estimation (JADE) MUSIC algorithm Vanderveen (1997). The ESPRIT algorithm The ESPRIT algorithm Roy and Kailath (1989) and the propagator method Marcos et al. (1994) Marcos et al. (1995) are two classical algorithms based on the shift-invariance-property. Both algorithms exploit a translational rotational invariance among signal subspaces induced by an array. In these algorithm, parameter estimates can be computed analytically. Thus, when applicable, these methods exhibit significant computational advantages over the methods that relies on solving optimization problems by exhaustive searching. As discussed in the previous section, the SAGE and the MUSIC algorithms need the knowledge of the array manifold, while the ESPRIT does not. However, the ESPRIT algorithm needs multiple sensor doublets. The elements in each doublet must have identical radiation patterns and are separated by known constant spacings. Apart from that requirement the radiation patterns can be arbitrary. The fundamental idea of the ESPRIT algorithm can be simply explained as follows. The underlying antenna array is divided into two subarrays. Each subarray consists of the same number of elements. The element spacing in each subarray and the spacing between the subarrays are known. The mth element in the 1st subarray and the mth element in the 2nd subarray compose a “doublet”. In this case, the array response C1(φ) of the 1st array and the array response C2(φ) of the 2nd array can be related by C1(φ) = C1(φ)Φ(φ), where Φ(φ) = diag[exp{jψ1}, . . . , exp{jψD}]. Here, ψd is the phase difference between the signals received at the two elements in each doublet for the dth path. This phase difference is a known function of the AoA φd. It can be shown that the columns in C1(φ) and the columns