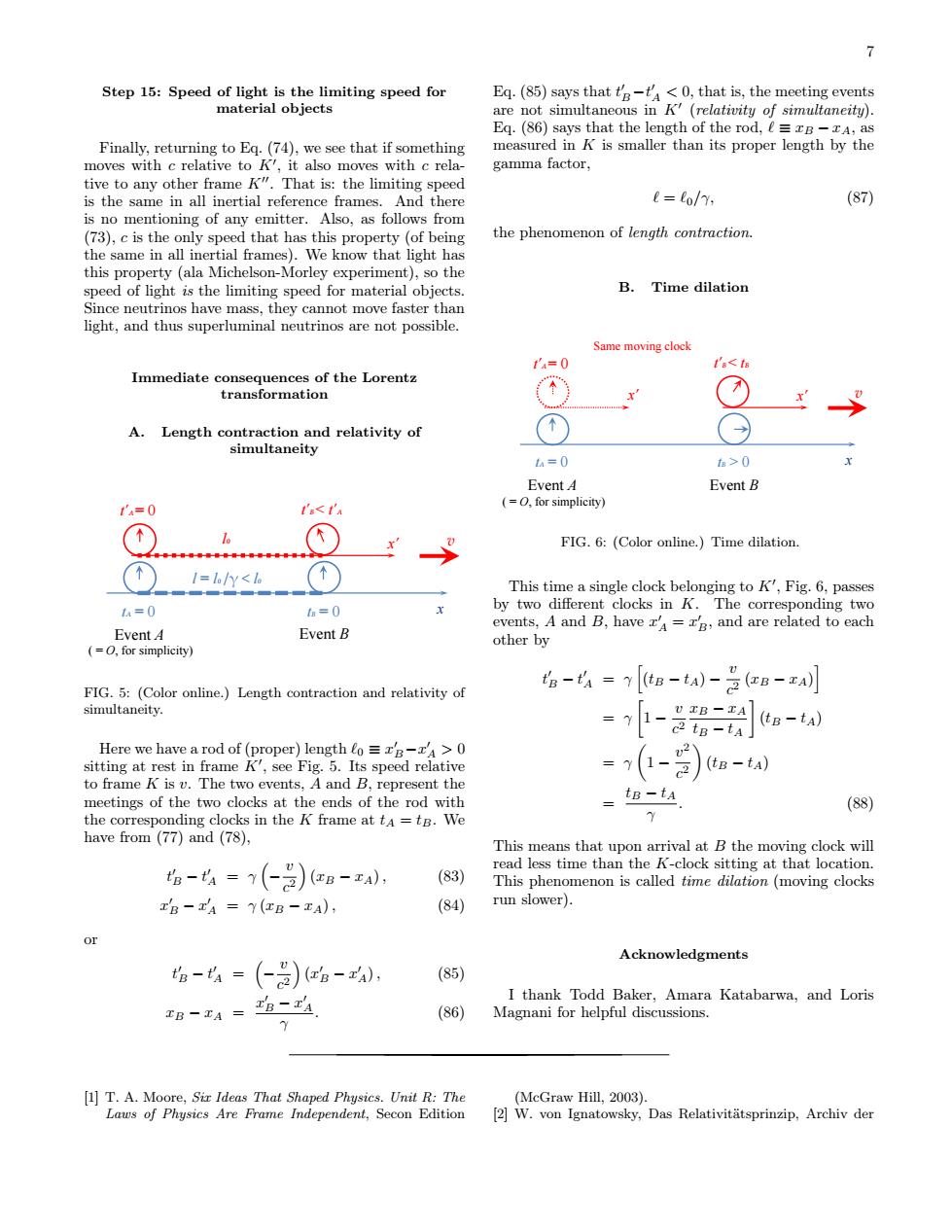
7 Step 15:Speed of light is the limiting speed for Eq.(85)says that t-t<0,that is,the meeting events material objects are not simultaneous in K'(relativity of simultaneity). Eq.(86)says that the length of the rod,e xB-zA,as Finally,returning to Eq.(74),we see that if something measured in K is smaller than its proper length by the moves with c relative to K,it also moves with c rela- gamma factor, tive to any other frame K".That is:the limiting speed is the same in all inertial reference frames.And there e=lo/y; (87) is no mentioning of any emitter.Also,as follows from (73),c is the only speed that has this property (of being the phenomenon of length contraction. the same in all inertial frames).We know that light has this property (ala Michelson-Morley experiment),so the speed of light is the limiting speed for material objects. B.Time dilation Since neutrinos have mass,they cannot move faster than light,and thus superluminal neutrinos are not possible. Same moving clock 1a=0 I'i<ts Immediate consequences of the Lorentz transformation A.Length contraction and relativity of simultaneity =0 ts>0 Event A Event B (=O,for simplicity） 1'A=0 FIG.6:(Color online.)Time dilation. This time a single clock belonging to K',Fig.6,passes =0 1a=0 by two different clocks in K.The corresponding two events,A and B,have r=B,and are related to each Event A Event B other by (=O,for simplicity) FIG.5:(Color online.)Length contraction and relativity of 名-4=7[ee-a-是eB-z simultaneity. =y1- U TB-TA c2tB-tA」 (tB-tA) Here we have a rod of (proper)length to =B-A>0 v- sitting at rest in frame K,see Fig.5.Its speed relative =（1-2)-t to frame K is v.The two events,A and B,represent the meetings of the two clocks at the ends of the rod with tB-iA (88) the corresponding clocks in the K frame at ta =tB.We Y have from (77)and (78), This means that upon arrival at B the moving clock will 指-么=7()e-小 read less time than the K-clock sitting at that location. (83) This phenomenon is called time dilation(moving clocks TB-TA Y(TB-IA), (84) run slower). or Acknowledgments 招-=()-， (85) I thank Todd Baker,Amara Katabarwa,and Loris TB-TA TB-TA (86) Magnani for helpful discussions. [1]T.A.Moore,Sir Ideas That Shaped Physics.Unit R:The (McGraw Hill,2003). Laws of Physics Are Frame Independent,Secon Edition [2]W.von Ignatowsky,Das Relativitatsprinzip,Archiv der7 Step 15: Speed of light is the limiting speed for material objects Finally, returning to Eq. (74), we see that if something moves with c relative to K0 , it also moves with c rela￾tive to any other frame K00. That is: the limiting speed is the same in all inertial reference frames. And there is no mentioning of any emitter. Also, as follows from (73), c is the only speed that has this property (of being the same in all inertial frames). We know that light has this property (ala Michelson-Morley experiment), so the speed of light is the limiting speed for material objects. Since neutrinos have mass, they cannot move faster than light, and thus superluminal neutrinos are not possible. Immediate consequences of the Lorentz transformation A. Length contraction and relativity of simultaneity x ’ v x Event A Event B ( = O, for simplicity) t’ A = 0 t’ B < t’ A tA = 0 tB = 0 l0 l = l0 /γ < l0 FIG. 5: (Color online.) Length contraction and relativity of simultaneity. Here we have a rod of (proper) length `0 ≡ x 0 B −x 0 A > 0 sitting at rest in frame K0 , see Fig. 5. Its speed relative to frame K is v. The two events, A and B, represent the meetings of the two clocks at the ends of the rod with the corresponding clocks in the K frame at tA = tB. We have from (77) and (78), t 0 B − t 0 A = γ  − v c 2  (xB − xA), (83) x 0 B − x 0 A = γ (xB − xA), (84) or t 0 B − t 0 A =  − v c 2  (x 0 B − x 0 A), (85) xB − xA = x 0 B − x 0 A γ . (86) Eq. (85) says that t 0 B −t 0 A < 0, that is, the meeting events are not simultaneous in K0 (relativity of simultaneity). Eq. (86) says that the length of the rod, ` ≡ xB − xA, as measured in K is smaller than its proper length by the gamma factor, ` = `0/γ, (87) the phenomenon of length contraction. B. Time dilation x ’ v x Event A Event B ( = O, for simplicity) t’ A = 0 t’ B < tB tA = 0 tB > 0 x ’ Same moving clock FIG. 6: (Color online.) Time dilation. This time a single clock belonging to K0 , Fig. 6, passes by two different clocks in K. The corresponding two events, A and B, have x 0 A = x 0 B, and are related to each other by t 0 B − t 0 A = γ h (tB − tA) − v c 2 (xB − xA) i = γ  1 − v c 2 xB − xA tB − tA  (tB − tA) = γ  1 − v 2 c 2  (tB − tA) = tB − tA γ . (88) This means that upon arrival at B the moving clock will read less time than the K-clock sitting at that location. This phenomenon is called time dilation (moving clocks run slower). Acknowledgments I thank Todd Baker, Amara Katabarwa, and Loris Magnani for helpful discussions. [1] T. A. Moore, Six Ideas That Shaped Physics. Unit R: The Laws of Physics Are Frame Independent, Secon Edition (McGraw Hill, 2003). [2] W. von Ignatowsky, Das Relativit¨atsprinzip, Archiv der