正在加载图片...
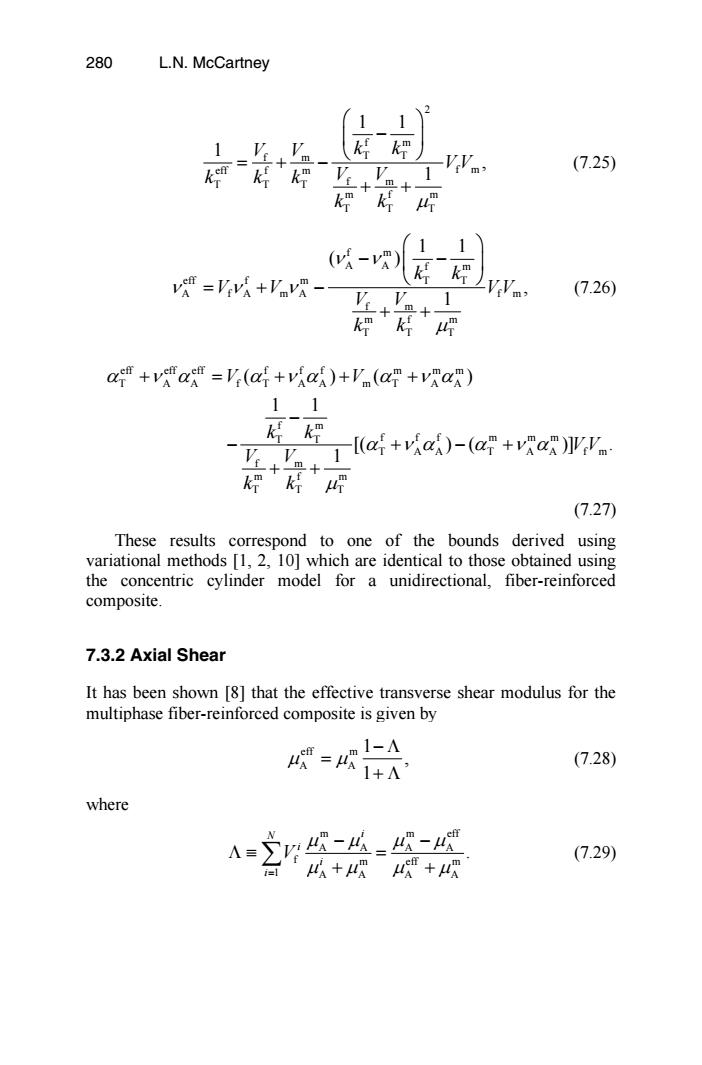
280 L.N.McCartney 1 ++ (7.25) m w- 711 vAn =VivA +VmVa- (7.26) ai+va=V(af+via)+V(a+vam) 11 @+a-安+ m十 (7.27) These results correspond to one of the bounds derived using variational methods [1,2,10]which are identical to those obtained using the concentric cylinder model for a unidirectional,fiber-reinforced composite 7.3.2 Axial Shear It has been shown [8]that the effective transverse shear modulus for the multiphase fiber-reinforced composite is given by =必会 (7.28) where A=之公-4-- (7.29) 台+吸+吸2 f m f m T T eff f m f m T TT f m mf m TTT 1 1 1 , 1 V V k k V V k kk V V k k µ ⎛ ⎞ ⎜ ⎟ − ⎝ ⎠ =+ − + + (7.25) f m A A f m eff f m T T A f A mA f m f m mf m TTT 1 1 ( ) , 1 k k V V V V V V k k ν ν ν ν ν µ ⎛ ⎞ − − ⎜ ⎟ ⎝ ⎠ =+ − + + (7.26) eff eff eff f f f m m m T A A f T AA m T A A f m T T f f f m mm T AA T A A fm f m mf m TTT ( )( ) 1 1 [( ) ( )] . 1 V V k k V V V V k k α ν α α να α να α να α να µ + =++ + − − + − + + + (7.27) These results correspond to one of the bounds derived using variational methods [1, 2, 10] which are identical to those obtained using the concentric cylinder model for a unidirectional, fiber-reinforced composite. 7.3.2 Axial Shear It has been shown [8] that the effective transverse shear modulus for the multiphase fiber-reinforced composite is given by eff m A A 1 , 1 µ µ − Λ = + Λ (7.28) where m meff AA AA f m eff m 1 AA A A . N i i i i V µµ µµ = µ µµµ − − Λ ≡ = + + ∑ (7.29) 280 L.N. McCartney