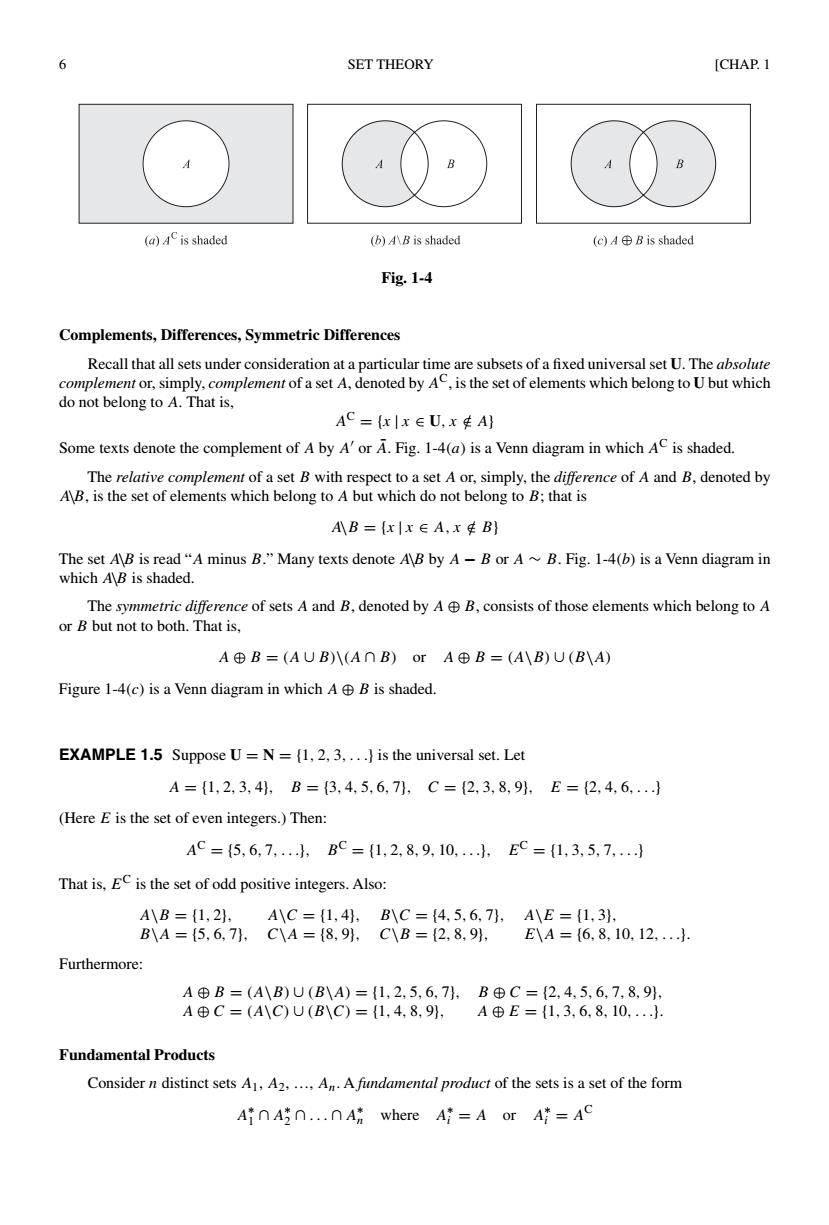
6 SET THEORY [CHAP.1 (a)Cis shaded (b)A\B is shaded (c)A⊕B is shaded Fig.1-4 Complements,Differences,Symmetric Differences Recall that all sets under consideration at a particular time are subsets of a fixed universal set U.The absolute complement or,simply,complement of a set A,denoted by AC,is the set of elements which belong to U but which do not belong to A.That is, AC={x|x∈U,x车A} Some texts denote the complement of A by A'or A.Fig.1-4(a)is a Venn diagram in which AC is shaded. The relative complement of a set B with respect to a set A or,simply,the difference of A and B,denoted by A B,is the set of elements which belong to A but which do not belong to B;that is A八B={x|x∈A,x年B) The set A B is read"A minus B."Many texts denote A B by A-B or A B.Fig.1-4(b)is a Venn diagram in which A B is shaded. The symmetric difference of sets A and B,denoted by A B,consists of those elements which belong to A or B but not to both.That is. A⊕B=(AUB)八(A∩B)orA⊕B=(A\B)U(B\A) Figure 1-4(c)is a Venn diagram in which AB is shaded. EXAMPLE 1.5 Suppose U=N=(1,2,3,...)is the universal set.Let A={1,2,3,41,B={3,4,5,6,71,C={2,3,8,91,E={2.4,6.} (Here E is the set of even integers.)Then: AC={5,6,7,,BC={1,2,8,9,10,,EC={1,3,5,7,.J That is,EC is the set of odd positive integers.Also: A\B={1,2,A\C={1,4,B\C={4,5,6,7,A\E={1,3, B\A={5,6,7),C\A={8,9,C\B={2,8,9},E\A={6,8,10,12,. Furthermore: A⊕B=(A\B)U(B\A)={1,2,5,6,7},B⊕C={2,4,5,6,7,8,9, A⊕C=(A\C)U(B\C)=1,4,8,9},A⊕E=1,36,8,10,.. Fundamental Products Consider n distinct sets A1,A2.....An.Afundamental product of the sets is a set of the form AinA2n...nA where A=A or A=AC6 SET THEORY [CHAP. 1 Fig. 1-4 Complements, Differences, Symmetric Differences Recall that all sets under consideration at a particular time are subsets of a fixed universal set U. The absolute complement or, simply, complement of a set A, denoted by AC, is the set of elements which belong to U but which do not belong to A. That is, AC = {x | x ∈ U,x /∈ A} Some texts denote the complement of A by A or A¯. Fig. 1-4(a) is a Venn diagram in which AC is shaded. The relative complement of a set B with respect to a set A or, simply, the difference of A and B, denoted by A\B, is the set of elements which belong to A but which do not belong to B; that is A\B = {x | x ∈ A, x /∈ B} The set A\B is read “A minus B.” Many texts denote A\B by A − B or A ∼ B. Fig. 1-4(b) is a Venn diagram in which A\B is shaded. The symmetric difference of sets A and B, denoted by A ⊕ B, consists of those elements which belong to A or B but not to both. That is, A ⊕ B = (A ∪ B)\(A ∩ B) or A ⊕ B = (A\B) ∪ (B\A) Figure 1-4(c) is a Venn diagram in which A ⊕ B is shaded. EXAMPLE 1.5 Suppose U = N = {1, 2, 3,...} is the universal set. Let A = {1, 2, 3, 4}, B = {3, 4, 5, 6, 7}, C = {2, 3, 8, 9}, E = {2, 4, 6,...} (Here E is the set of even integers.) Then: AC = {5, 6, 7,...}, BC = {1, 2, 8, 9, 10,...}, EC = {1, 3, 5, 7,...} That is, EC is the set of odd positive integers. Also: A\B = {1, 2}, A\C = {1, 4}, B\C = {4, 5, 6, 7}, A\E = {1, 3}, B\A = {5, 6, 7}, C\A = {8, 9}, C\B = {2, 8, 9}, E\A = {6, 8, 10, 12,...}. Furthermore: A ⊕ B = (A\B) ∪ (B\A) = {1, 2, 5, 6, 7}, B ⊕ C = {2, 4, 5, 6, 7, 8, 9}, A ⊕ C = (A\C) ∪ (B\C) = {1, 4, 8, 9}, A ⊕ E = {1, 3, 6, 8, 10,...}. Fundamental Products Consider n distinct sets A1, A2, …, An. A fundamental product of the sets is a set of the form A∗ 1 ∩ A∗ 2 ∩ ... ∩ A∗ n where A∗ i = A or A∗ i = AC