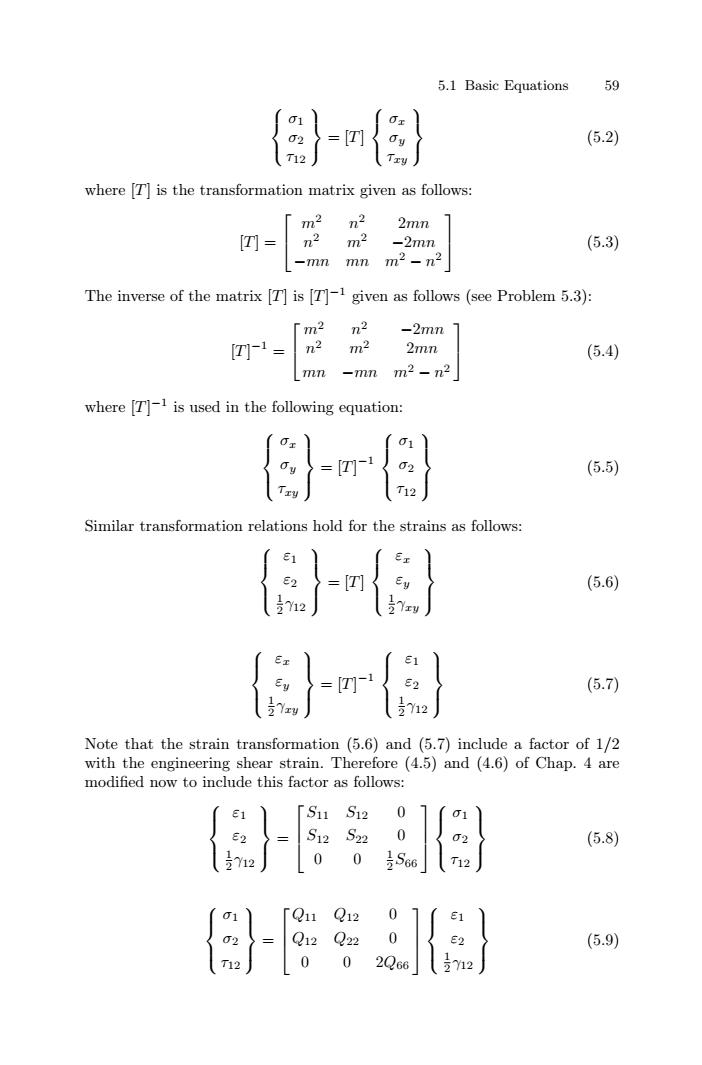
5.1 Basic Equations 59 }- (5.2) where [T is the transformation matrix given as follows: 3 2 2mn [T= n2 m2 -2mn (5.3) -mn mn m2-n2 The inverse of the matrix [T]is [T]-given as follows(see Problem 5.3): 「m2 n2 -2mn T]-1= n2 m2 2mn (5.4) m -mn m2-n2 where [T]is used in the following equation: (5.5) Similar transformation relations hold for the strains as follows: E1 E2 T (5.6) 312 =四1 (5.7) 12 Note that the strain transformation (5.6)and (5.7)include a factor of 1/2 with the engineering shear strain.Therefore (4.5)and (4.6)of Chap.4 are modified now to include this factor as follows: S12 (5.8) 0 11 Q12 Q22 (5.9) 0 2Q665.1 Basic Equations 59 ⎧ ⎨ ⎩ σ1 σ2 τ12 ⎫ ⎬ ⎭ = [T] ⎧ ⎨ ⎩ σx σy τxy ⎫ ⎬ ⎭ (5.2) where [T] is the transformation matrix given as follows: [T] = ⎡ ⎣ m2 n2 2mn n2 m2 −2mn −mn mn m2 − n2 ⎤ ⎦ (5.3) The inverse of the matrix [T] is [T] −1 given as follows (see Problem 5.3): [T] −1 = ⎡ ⎣ m2 n2 −2mn n2 m2 2mn mn −mn m2 − n2 ⎤ ⎦ (5.4) where [T] −1 is used in the following equation: ⎧ ⎪⎨ ⎪⎩ σx σy τxy ⎫ ⎪⎬ ⎪⎭ = [T] −1 ⎧ ⎪⎨ ⎪⎩ σ1 σ2 τ12 ⎫ ⎪⎬ ⎪⎭ (5.5) Similar transformation relations hold for the strains as follows: ⎧ ⎪⎨ ⎪⎩ ε1 ε2 1 2 γ12 ⎫ ⎪⎬ ⎪⎭ = [T] ⎧ ⎪⎨ ⎪⎩ εx εy 1 2 γxy ⎫ ⎪⎬ ⎪⎭ (5.6) ⎧ ⎪⎨ ⎪⎩ εx εy 1 2 γxy ⎫ ⎪⎬ ⎪⎭ = [T] −1 ⎧ ⎪⎨ ⎪⎩ ε1 ε2 1 2 γ12 ⎫ ⎪⎬ ⎪⎭ (5.7) Note that the strain transformation (5.6) and (5.7) include a factor of 1/2 with the engineering shear strain. Therefore (4.5) and (4.6) of Chap. 4 are modified now to include this factor as follows: ⎧ ⎪⎨ ⎪⎩ ε1 ε2 1 2 γ12 ⎫ ⎪⎬ ⎪⎭ = ⎡ ⎢ ⎣ S11 S12 0 S12 S22 0 0 0 1 2S66 ⎤ ⎥ ⎦ ⎧ ⎪⎨ ⎪⎩ σ1 σ2 τ12 ⎫ ⎪⎬ ⎪⎭ (5.8) ⎧ ⎪⎨ ⎪⎩ σ1 σ2 τ12 ⎫ ⎪⎬ ⎪⎭ = ⎡ ⎢ ⎣ Q11 Q12 0 Q12 Q22 0 0 02Q66 ⎤ ⎥ ⎦ ⎧ ⎪⎨ ⎪⎩ ε1 ε2 1 2 γ12 ⎫ ⎪⎬ ⎪⎭ (5.9)