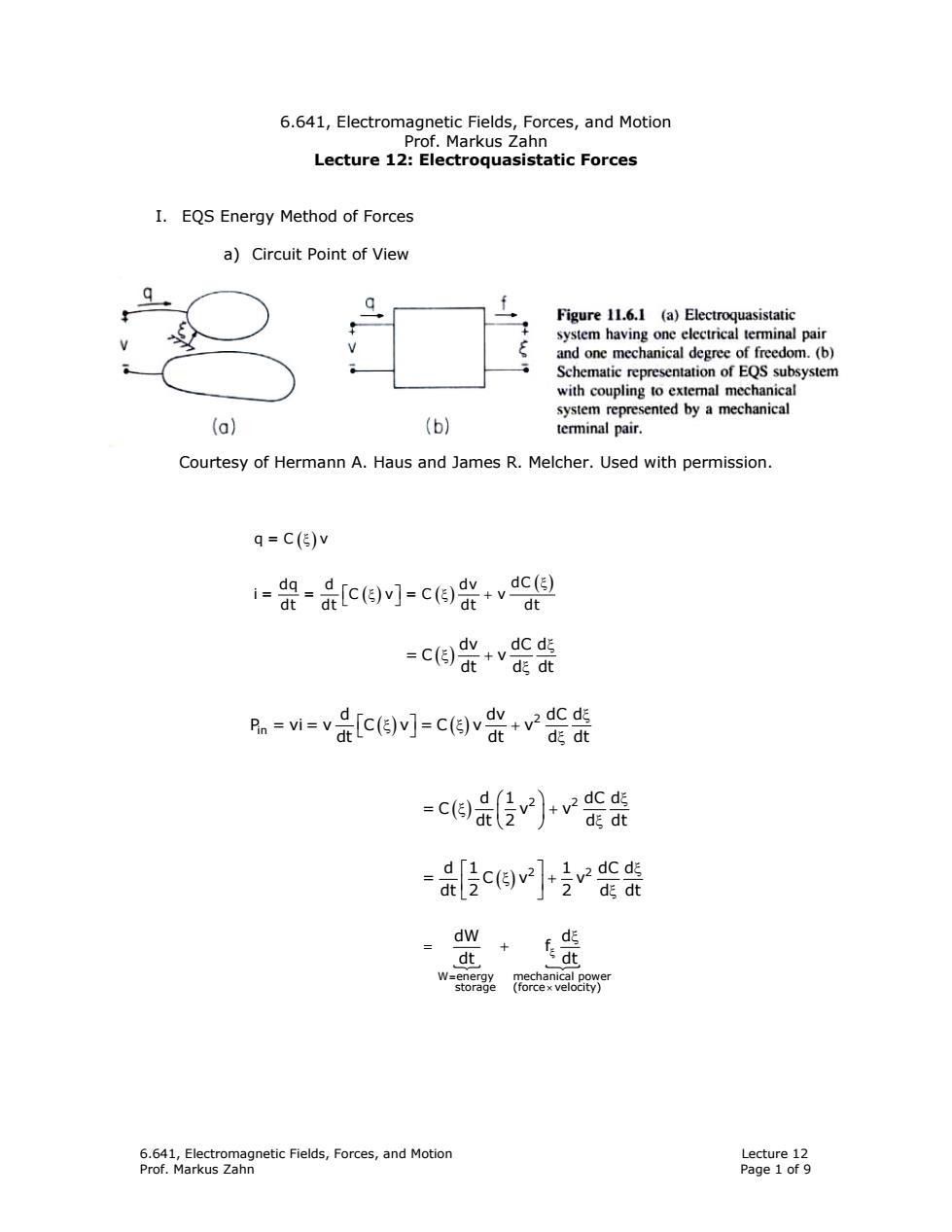
6.641,Electromagnetic Fields,Forces,and Motion Prof.Markus Zahn Lecture 12:Electroquasistatic Forces I.EQS Energy Method of Forces a)Circuit Point of View Figure 11.6.1 (a)Electroquasistatic system having one electrical terminal pair and one mechanical degree of freedom.(b) Schematic representation of EQS subsystem with coupling to extemal mechanical system represented by a mechanical (a) (b) terminal pair. Courtesy of Hermann A.Haus and James R.Melcher.Used with permission. q=C()v i-=品[cey]-ce0+vace dt =c附0+ve da dt R=m=v是[c(y-c张+E架 d dt =ce是va d dt -Bce +1v2 dc d d dt dw d dt W=energy mechanical power storage (force x velocity) 6.641,Electromagnetic Fields,Forces,and Motion Lecture 12 Prof.Markus Zahn Page 1 of 96.641, Electromagnetic Fields, Forces, and Motion Lecture 12 Prof. Markus Zahn Page 1 of 9 6.641, Electromagnetic Fields, Forces, and Motion Prof. Markus Zahn Lecture 12: Electroquasistatic Forces I. EQS Energy Method of Forces a) Circuit Point of View Courtesy of Hermann A. Haus and James R. Melcher. Used with permission. q=C v ( ) ξ () () (ξ) ⎡ ⎤ ξ ξ+ ⎣ ⎦ dq d dv dC i= = C v =C v dt dt dt dt ( ) ξ ξ + ξ dv dC d =C v dt d dt ( ) ⎛ ⎞ ξ ξ + ⎜ ⎟ ⎝ ⎠ ξ d 1 dC d 2 2 =C v v dt 2 d dt ( ) ⎡ ⎤ ξ ξ + ⎢ ⎥ ⎣ ⎦ ξ d 1 1 dC d 2 2 = Cv v dt 2 2 d dt N N W energy mechanical power storage (force velocity) dW d f dt dt ξ = × ξ = + () () ξ ⎡ ⎤ ξ ξ+ ⎣ ⎦ ξ 2 in d dv P = vi = v C v = C v v dt dt d dt dC d