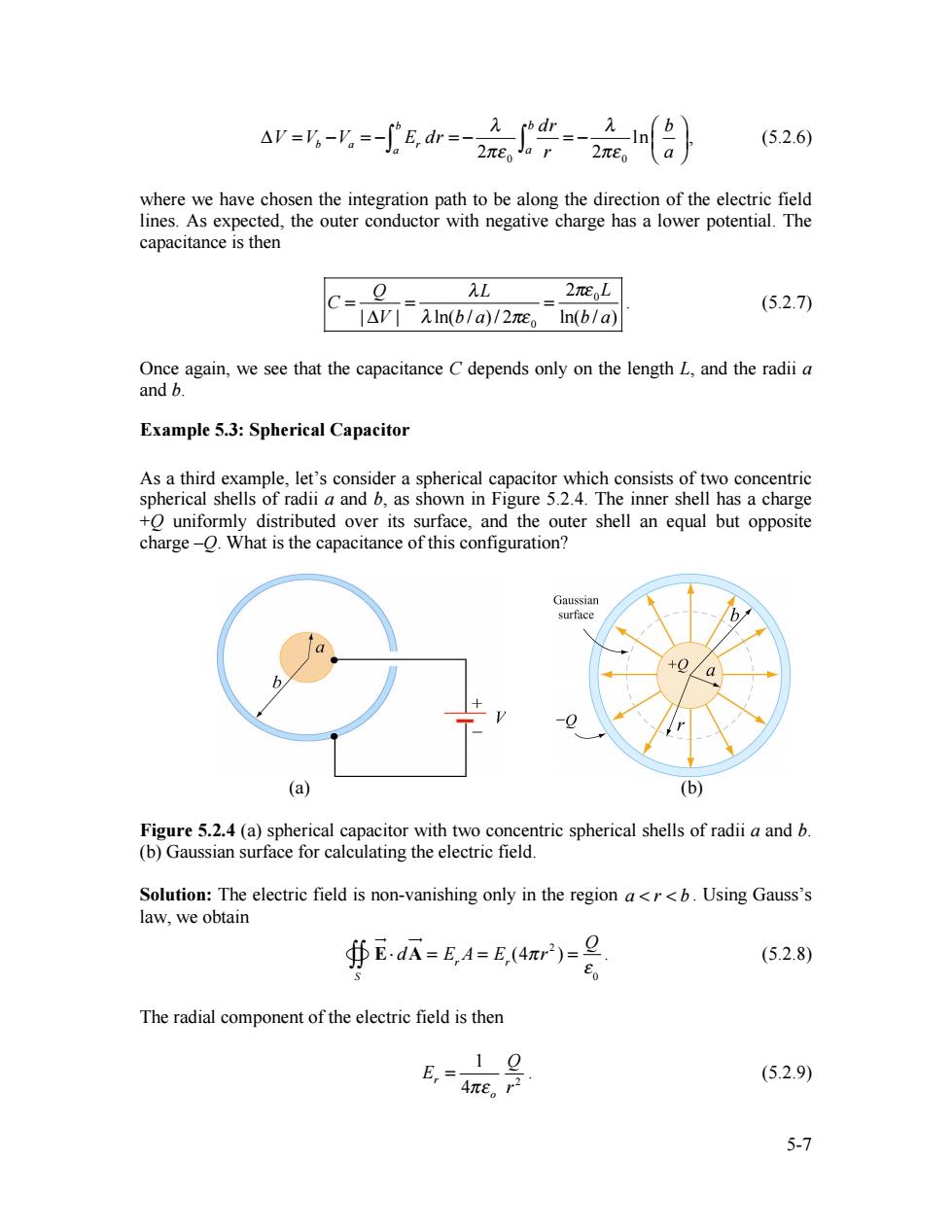
(5.2.6) where we have chosen the integration path to be along the direction of the electric field lines.As expected,the outer conductor with negative charge has a lower potential.The capacitance is then Q AL 2πeL C= (5.2.7) |△'|2ln(b/a)/2re。ln(b/a) Once again,we see that the capacitance C depends only on the length L,and the radii a and b. Example 5.3:Spherical Capacitor As a third example,let's consider a spherical capacitor which consists of two concentric spherical shells of radii a and b,as shown in Figure 5.2.4.The inner shell has a charge +O uniformly distributed over its surface,and the outer shell an equal but opposite charge-O.What is the capacitance of this configuration? Gaussian surface 0 (a) (b) Figure 5.2.4 (a)spherical capacitor with two concentric spherical shells of radii a and b. (b)Gaussian surface for calculating the electric field. Solution:The electric field is non-vanishing only in the region a<r<b.Using Gauss's law,we obtain ∯E,=E4-E4红)=号 (5.2.8) The radial component of the electric field is then E.= 1 (5.2.9) 4πe。r2 5-75-7 0 0 ln 2 2 b b a r a b a dr b V V V E dr r a ! ! "# "# $ % & = ' = ' = ' = ' ( ) * + , , , (5.2.6) where we have chosen the integration path to be along the direction of the electric field lines. As expected, the outer conductor with negative charge has a lower potential. The capacitance is then 0 0 2 | | ln( / )/ 2 ln( / ) Q L L C V b a b a # !" # !" = = = $ . (5.2.7) Once again, we see that the capacitance C depends only on the length L, and the radii a and b. Example 5.3: Spherical Capacitor As a third example, let’s consider a spherical capacitor which consists of two concentric spherical shells of radii a and b, as shown in Figure 5.2.4. The inner shell has a charge +Q uniformly distributed over its surface, and the outer shell an equal but opposite charge –Q. What is the capacitance of this configuration? (a) (b) Figure 5.2.4 (a) spherical capacitor with two concentric spherical shells of radii a and b. (b) Gaussian surface for calculating the electric field. Solution: The electric field is non-vanishing only in the region a < r < b . Using Gauss’s law, we obtain E !" ! dA !" S #"" = Er A = Er (4# r 2 ) = Q $ 0 . (5.2.8) The radial component of the electric field is then 2 1 4 r o Q E !" r = . (5.2.9)