正在加载图片...
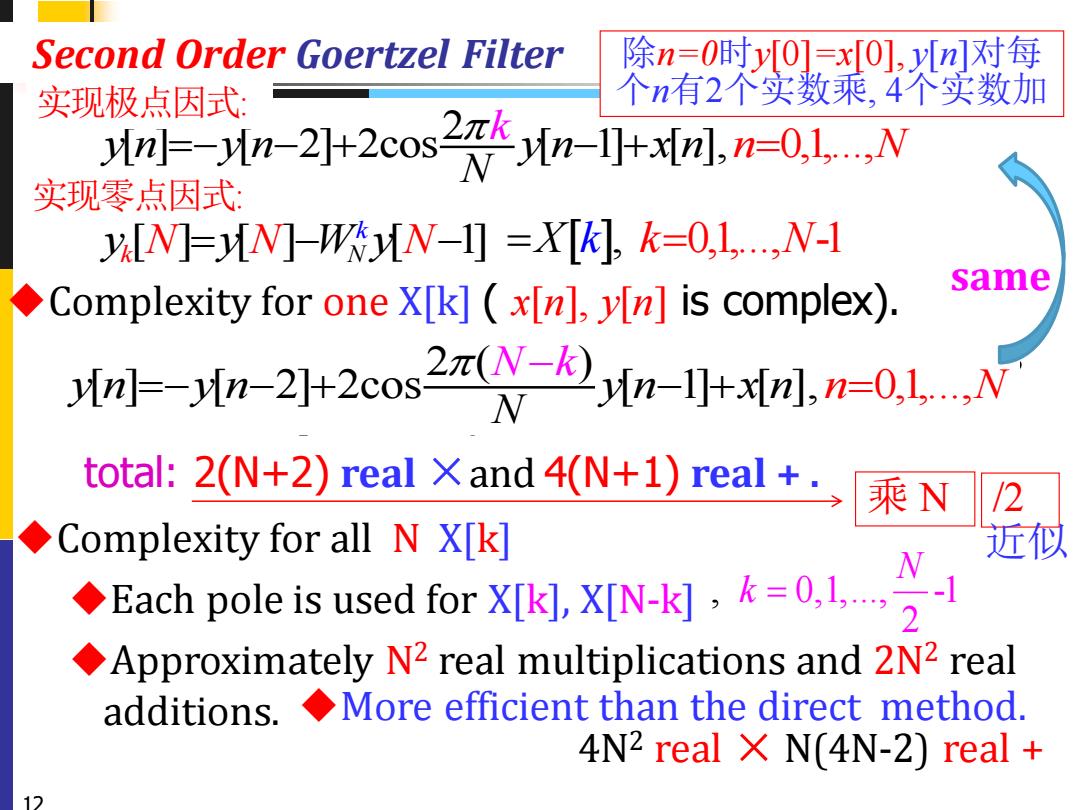
Second Order Goertzel Filter 除n=0时y[0]=xf0],ym对每 实现极点因式 个n有2个实数乘,4个实数加 -+2cos0.1. 实现零点因式: ylN]yN]WyN-1]=Xk,k=0,1,...,N-1 same Complexity for one x[k](x[n],y[n]is complex). yAnl=-yAn-2+2cos2z(N-Kyin-I+xfn.n-0.1N total:2(N+2)real X and 4(N+1)real + 乘N 12 Complexity for all N X[k] 近似 Each pole is used for X[k],X[N-k],k=0.1.... Approximately N2 real multiplications and 2N2 real additions.More efficient than the direct method. 4N2 real X N(4N-2)real12 Second Order Goertzel Filter ◆Complexity for one X[k] ( x[n], y[n] is complex). ◆Poles: 2N real multiplications and 4N real additions ◆Zeros: computed only once: 4 real ×and 4 real + . 2 y n y n y n x n [ ] [ 2] 2cos [ 1] [ ], 0,1,..., N n N k =− − + − + = [ ] [ ] [ 1] N k y y W k N = − − N Ny =Xk, k=0,1,..., -1 N total: 2(N+2) real ×and 4(N+1) real + . ◆Complexity for all N X[k] ◆Each pole is used for X[k], X[N-k] ◆Approximately N2 real multiplications and 2N2 real additions. 2 ( ) y n y n y n x n [ ] [ 2] 2cos [ 1] [ ], 0,1,..., N N k n N − =− − + − + = same ◆More efficient than the direct method. 除n=0时y[0]=x[0], y[n]对每 个n有2个实数乘, 4个实数加 乘 N , 0,1,..., -1 2 N k = 实现极点因式: 实现零点因式: /2 4N2 real × N(4N-2) real + Complexity of first Order Goertzel Filter for one X[k]: all 近似