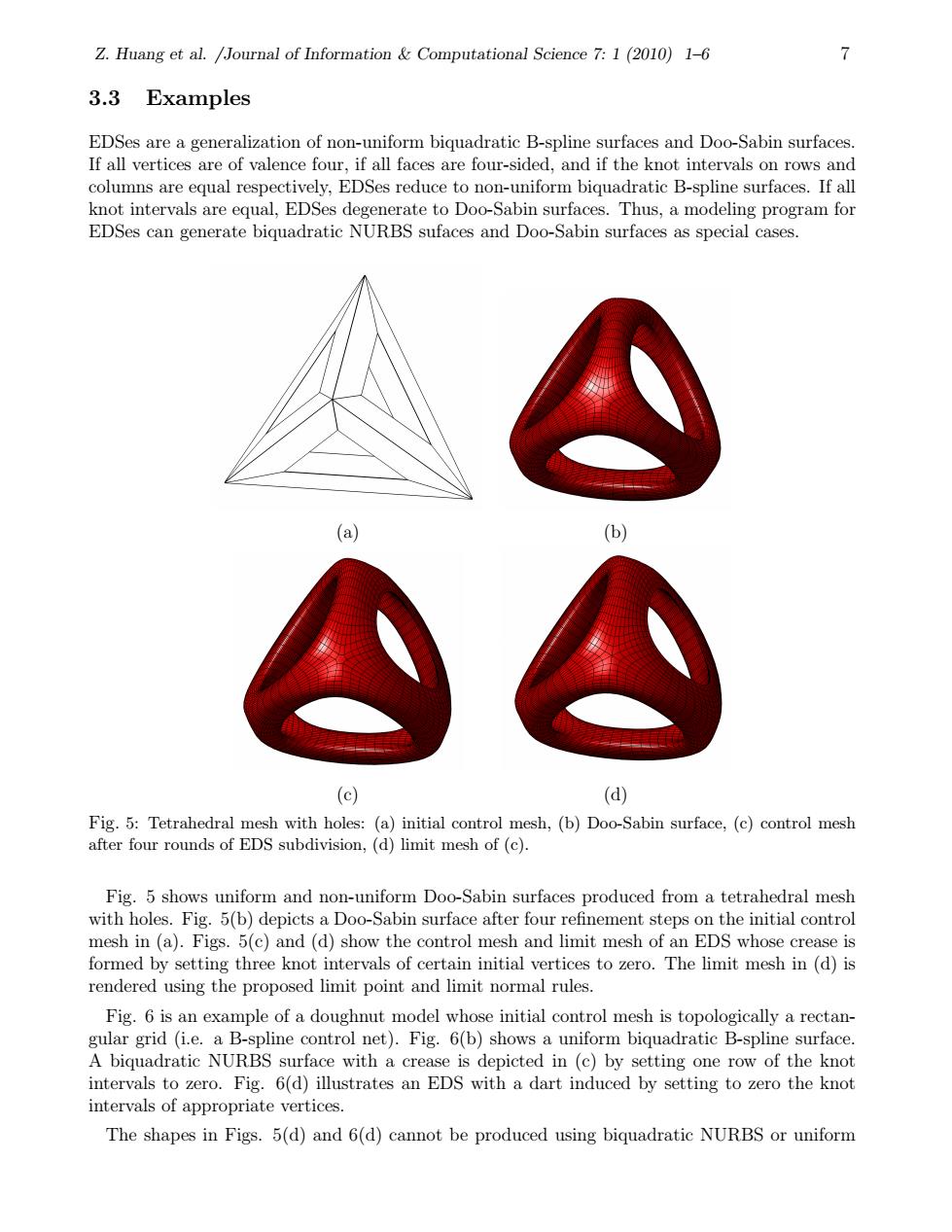
3.3 Examples es red (e) (d] 时份。oo8地te付am时 d (d) an d aa EDS with The shapes in Figs.5(d)and 6(d)camot be produced using biquadratic NURBSZ. Huang et al. /Journal of Information & Computational Science 7: 1 (2010) 1–6 7 3.3 Examples EDSes are a generalization of non-uniform biquadratic B-spline surfaces and Doo-Sabin surfaces. If all vertices are of valence four, if all faces are four-sided, and if the knot intervals on rows and columns are equal respectively, EDSes reduce to non-uniform biquadratic B-spline surfaces. If all knot intervals are equal, EDSes degenerate to Doo-Sabin surfaces. Thus, a modeling program for EDSes can generate biquadratic NURBS sufaces and Doo-Sabin surfaces as special cases. (a) (b) (c) (d) Fig. 5: Tetrahedral mesh with holes: (a) initial control mesh, (b) Doo-Sabin surface, (c) control mesh after four rounds of EDS subdivision, (d) limit mesh of (c). Fig. 5 shows uniform and non-uniform Doo-Sabin surfaces produced from a tetrahedral mesh with holes. Fig. 5(b) depicts a Doo-Sabin surface after four refinement steps on the initial control mesh in (a). Figs. 5(c) and (d) show the control mesh and limit mesh of an EDS whose crease is formed by setting three knot intervals of certain initial vertices to zero. The limit mesh in (d) is rendered using the proposed limit point and limit normal rules. Fig. 6 is an example of a doughnut model whose initial control mesh is topologically a rectan￾gular grid (i.e. a B-spline control net). Fig. 6(b) shows a uniform biquadratic B-spline surface. A biquadratic NURBS surface with a crease is depicted in (c) by setting one row of the knot intervals to zero. Fig. 6(d) illustrates an EDS with a dart induced by setting to zero the knot intervals of appropriate vertices. The shapes in Figs. 5(d) and 6(d) cannot be produced using biquadratic NURBS or uniform