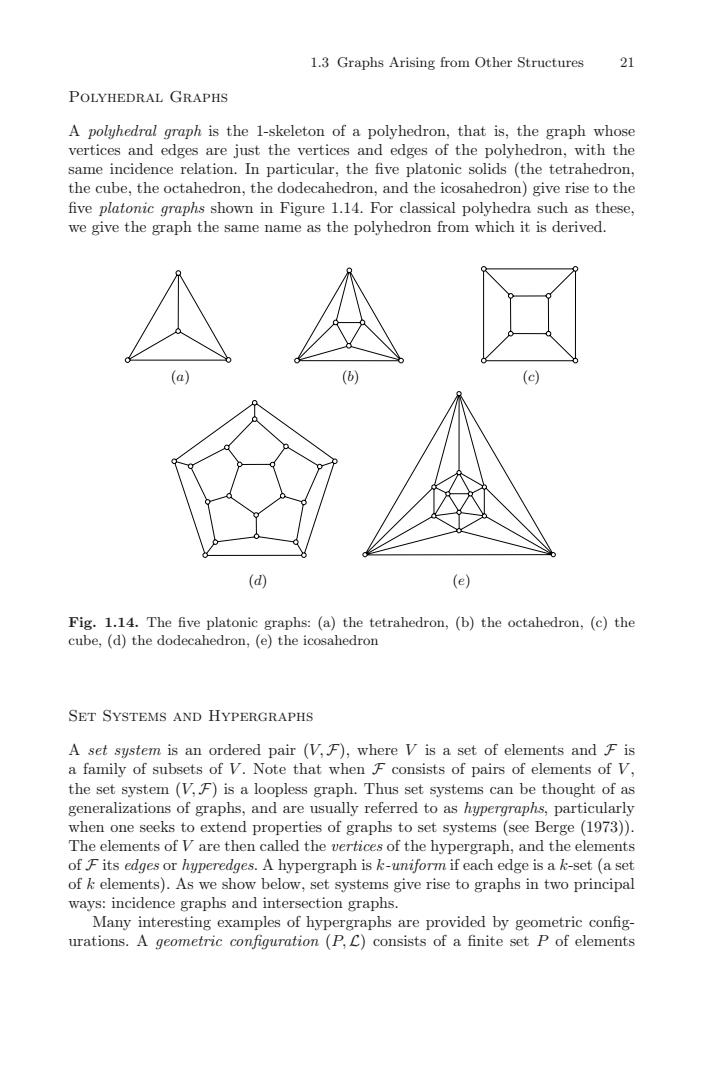
1.3GraphsArisingfromOtherStructures21POLYHEDRAL GRAPHSA polyhedral graph is the 1-skeleton of a polyhedron, that is, the graph whovertices and edges are just the vertices and edges of the polyhedron, with thesame incidence relation.In particular,the five platonic solids (the tetrahedronthe cube, the octahedron, the dodecahedron, and the icosahedron) give rise to thefive platonic graphs shown in Figure 1.14. For classical polyhedra such as these,we give the graph the same nameleasthepolyhedron from whichitisderivedAA(a)(c)(d)(e)Fig. 1.14. The five platonic graphs: (a) the tetrahedron, (b) the octahedron, (c) thecube, (d) the dodecahedron, (e) the icosahedronSET SYSTEMS AND HYPERGRAPHSered pair (VF),where V is a set of elements and F iA setsystemisaoa family of subsets of V. Note that when F consists of pairs of elements of Vthe set system (V, F) is a loopless graph. Thus set systems can be thought of asgeneralizations of graphs, and are usuallyreferred to as hypergraphs,particularlywhen one seeks to extend properties of graphs to set systems (see Berge (1973).The elements of V are then called the uertices of the hypergraph, and the elementsof F its edges or hyperedges. A hypergraph is k-uniform if each edge is a k-set (a setof k elements). As we show below, set systems give rise to graphs in two principalways incidence graphs and intersection graphsMany interesting examples of hypergraphs are provided by geometric configurations. A geometric configuration (P,C) consists of a finite set P of elements1.3 Graphs Arising from Other Structures 21 Polyhedral Graphs A polyhedral graph is the 1-skeleton of a polyhedron, that is, the graph whose vertices and edges are just the vertices and edges of the polyhedron, with the same incidence relation. In particular, the five platonic solids (the tetrahedron, the cube, the octahedron, the dodecahedron, and the icosahedron) give rise to the five platonic graphs shown in Figure 1.14. For classical polyhedra such as these, we give the graph the same name as the polyhedron from which it is derived. (a) (b) (c) (d) (e) Fig. 1.14. The five platonic graphs: (a) the tetrahedron, (b) the octahedron, (c) the cube, (d) the dodecahedron, (e) the icosahedron Set Systems and Hypergraphs A set system is an ordered pair (V, F), where V is a set of elements and F is a family of subsets of V . Note that when F consists of pairs of elements of V , the set system (V, F) is a loopless graph. Thus set systems can be thought of as generalizations of graphs, and are usually referred to as hypergraphs, particularly when one seeks to extend properties of graphs to set systems (see Berge (1973)). The elements of V are then called the vertices of the hypergraph, and the elements of F its edges or hyperedges. A hypergraph is k-uniform if each edge is a k-set (a set of k elements). As we show below, set systems give rise to graphs in two principal ways: incidence graphs and intersection graphs. Many interesting examples of hypergraphs are provided by geometric config￾urations. A geometric configuration (P,L) consists of a finite set P of elements