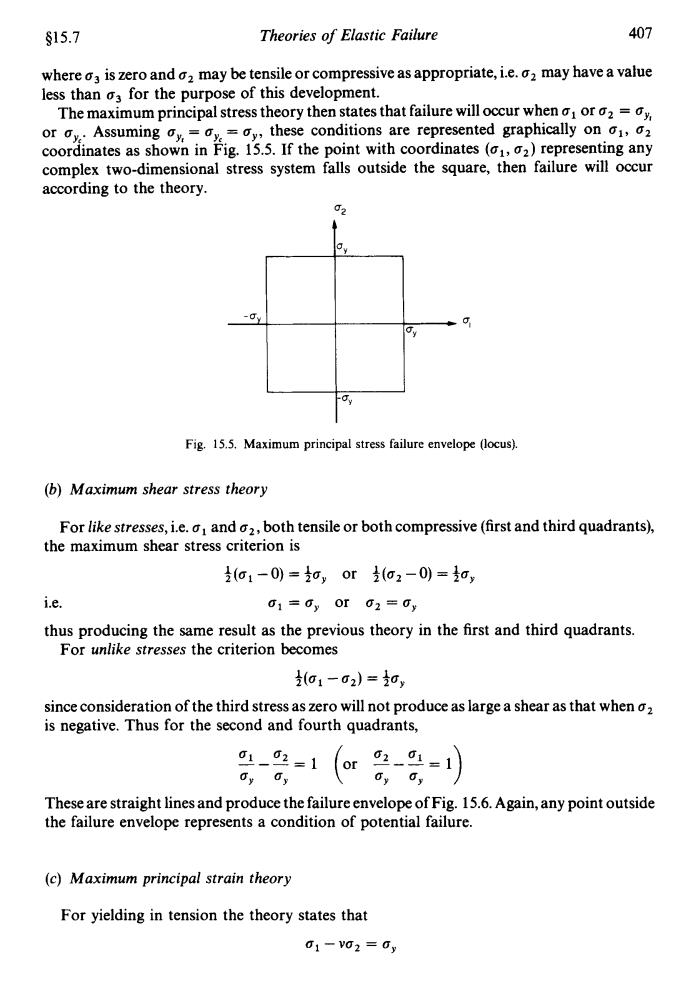
§15.7 Theories of Elastic Failure 407 where a is zero and may be tensile or compressive as appropriate,i.e.2 may have a value less than o3 for the purpose of this development. The maximum principal stress theory then states that failure will occur when a or 2=y or ay.Assuming oy=yoy,these conditions are represented graphically on 1,2 coordinates as shown in Fig.15.5.If the point with coordinates (1,2)representing any complex two-dimensional stress system falls outside the square,then failure will occur according to the theory. 02 -Oy Fig.15.5.Maximum principal stress failure envelope (locus). (b)Maximum shear stress theory For like stresses,i.e.and2,both tensile or both compressive(first and third quadrants), the maximum shear stress criterion is (o1-0)=o,or(o2-0)=o, i.e. 01=0y0r02=0y thus producing the same result as the previous theory in the first and third quadrants. For unlike stresses the criterion becomes (o1-02)=0y since consideration of the third stress as zero will not produce as large a shear as that when o2 is negative.Thus for the second and fourth quadrants, These are straight lines and produce the failure envelope of Fig.15.6.Again,any point outside the failure envelope represents a condition of potential failure. (c)Maximum principal strain theory For yielding in tension the theory states that 01-vo2=0y$15.7 Theories of Elastic Failure 407 where a3 is zero and a2 may be tensile or compressive as appropriate, i.e. a2 may have a value less than a3 for the purpose of this development. The maximum principal stress theory then states that failure will occur when a1 or a2 = a,,, or a,,,. Assuming a,,, = a,,, = a,,, these conditions are represented graphically on aI, a2 coordinates as shown in Fig. 15.5. If the point with coordinates (al, a2) representing any complex two-dimensional stress system falls outside the square, then failure will occur according to the theory. 02 t Fig. 15.5. Maximum principal stress failure envelope (locus). (b) Maximum shear stress theory For like stresses, i.e. a1 and a2, both tensile or both compressive (first and third quadrants), the maximum shear stress criterion is +(al -0) = $0, or $(a2 -0) =+ay i.e. a1 = ay or a2 =ay thus producing the same result as the previous theory in the first and third quadrants. For unlike stresses the criterion becomes +(a1 - 62) = 3.y since consideration of the third stress as zero will not produce as large a shear as that when a2 is negative. Thus for the second and fourth quadrants, These are straight lines and produce the failure envelope of Fig. 15.6. Again, any point outside the failure envelope represents a condition of potential failure. (c) Maximum principal strain theory For yielding in tension the theory states that 61 --a2 = by