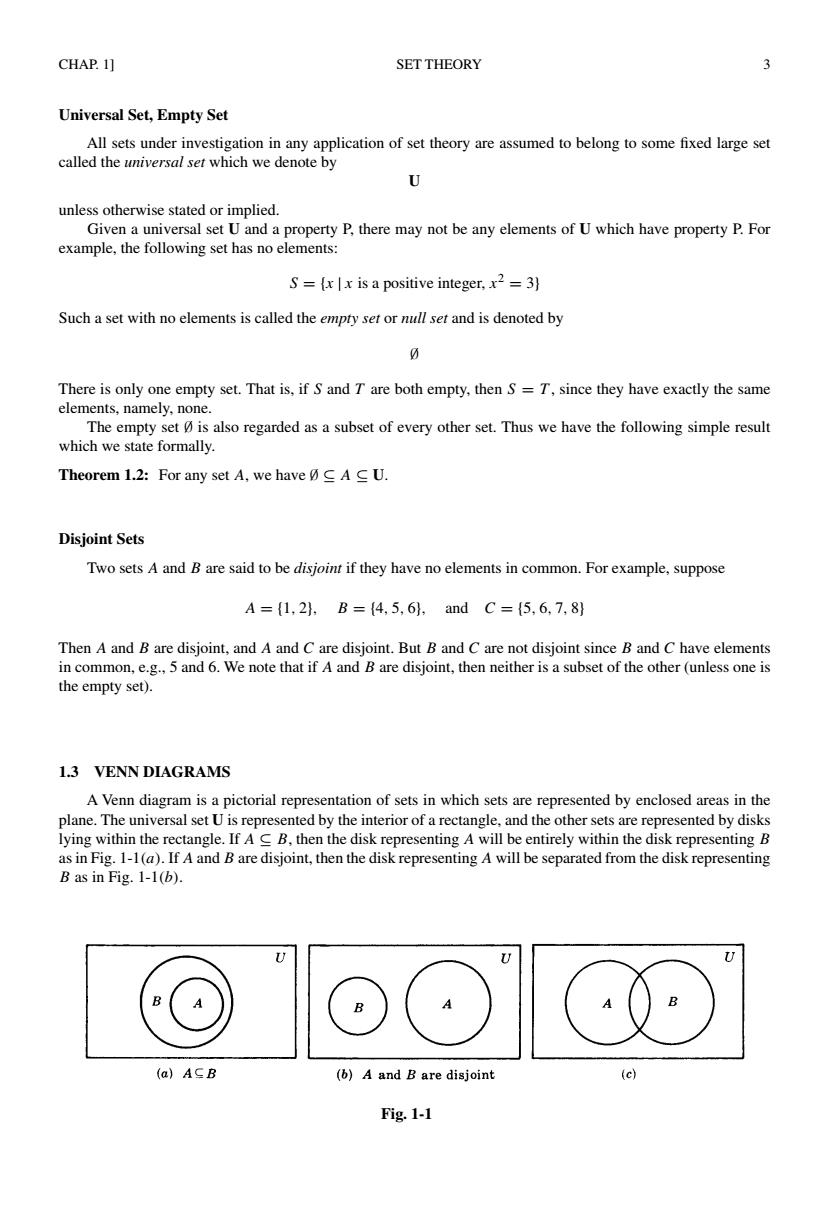
CHAP.1] SET THEORY 3 Universal Set,Empty Set All sets under investigation in any application of set theory are assumed to belong to some fixed large set called the universal set which we denote by ◇ unless otherwise stated or implied. Given a universal set U and a property P,there may not be any elements of U which have property P.For example,the following set has no elements: S=xx is a positive integer,x2=3} Such a set with no elements is called the empty set or null set and is denoted by 分 There is only one empty set.That is,if S and T are both empty,then S=T,since they have exactly the same elements,namely,none. The empty set is also regarded as a subset of every other set.Thus we have the following simple result which we state formally. Theorem 1.2:For any set A,we have 0A C U. Disjoint Sets Two sets A and B are said to be disjoint if they have no elements in common.For example,suppose A={1,2,B={4,5,6,andC={5,6,7,8} Then A and B are disjoint,and A and C are disjoint.But B and C are not disjoint since B and C have elements in common,e.g.,5 and 6.We note that if A and B are disjoint,then neither is a subset of the other (unless one is the empty set). 1.3 VENN DIAGRAMS A Venn diagram is a pictorial representation of sets in which sets are represented by enclosed areas in the plane.The universal set U is represented by the interior of a rectangle,and the other sets are represented by disks lying within the rectangle.If A C B,then the disk representing A will be entirely within the disk representing B as in Fig.1-1(a).If A and B are disjoint,then the disk representing A will be separated from the disk representing B as in Fig.1-1(b). B (a)ACB (b)A and B are disjoint (c) Fig.1-1CHAP. 1] SET THEORY 3 Universal Set, Empty Set All sets under investigation in any application of set theory are assumed to belong to some fixed large set called the universal set which we denote by U unless otherwise stated or implied. Given a universal set U and a property P, there may not be any elements of U which have property P. For example, the following set has no elements: S = {x | x is a positive integer, x2 = 3} Such a set with no elements is called the empty set or null set and is denoted by ∅ There is only one empty set. That is, if S and T are both empty, then S = T , since they have exactly the same elements, namely, none. The empty set ∅ is also regarded as a subset of every other set. Thus we have the following simple result which we state formally. Theorem 1.2: For any set A, we have ∅ ⊆ A ⊆ U. Disjoint Sets Two sets A and B are said to be disjoint if they have no elements in common. For example, suppose A = {1, 2}, B = {4, 5, 6}, and C = {5, 6, 7, 8} Then A and B are disjoint, and A and C are disjoint. But B and C are not disjoint since B and C have elements in common, e.g., 5 and 6. We note that if A and B are disjoint, then neither is a subset of the other (unless one is the empty set). 1.3 VENN DIAGRAMS A Venn diagram is a pictorial representation of sets in which sets are represented by enclosed areas in the plane. The universal set U is represented by the interior of a rectangle, and the other sets are represented by disks lying within the rectangle. If A ⊆ B, then the disk representing A will be entirely within the disk representing B as in Fig. 1-1(a). If A and B are disjoint, then the disk representing A will be separated from the disk representing B as in Fig. 1-1(b). Fig. 1-1