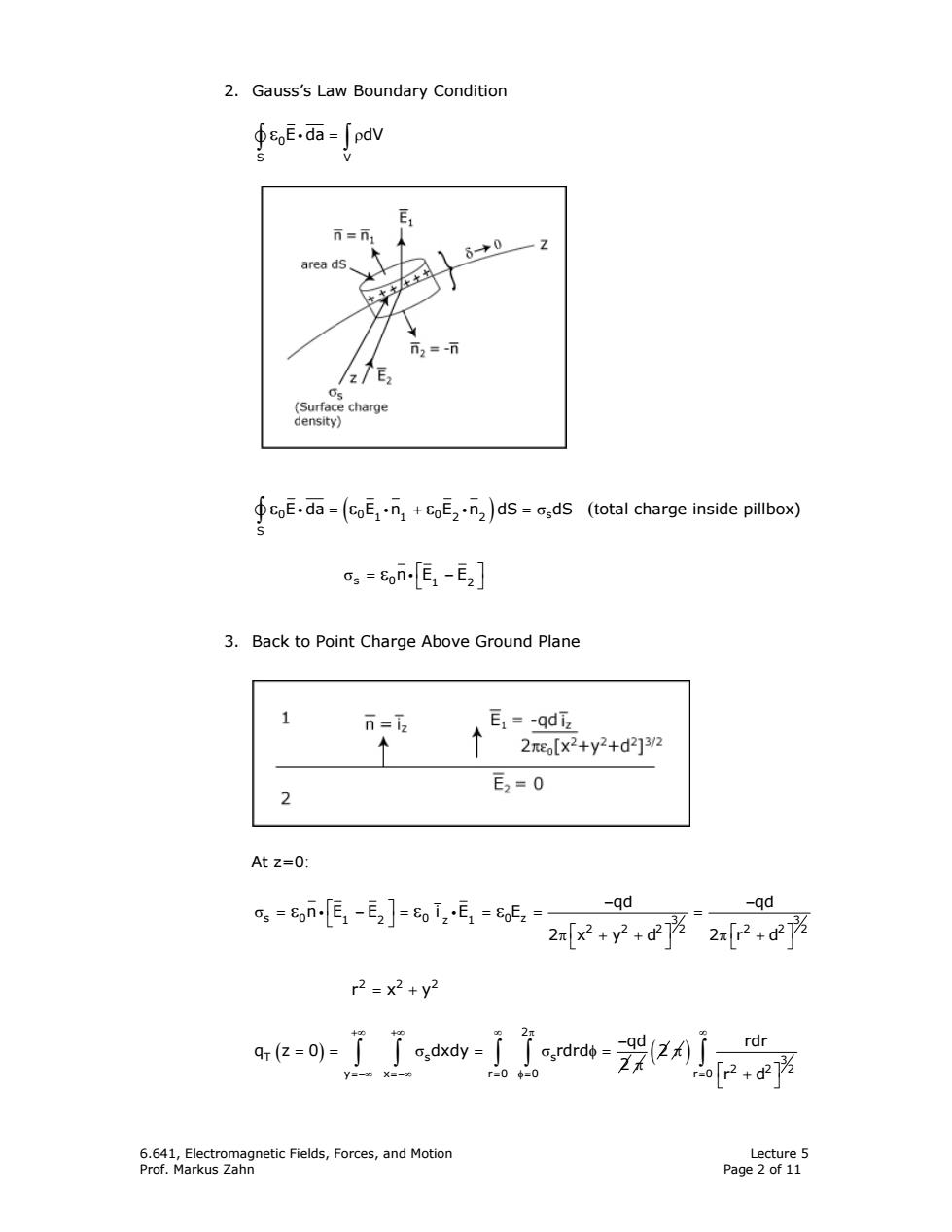
2.Gauss's Law Boundary Condition ∮E.da=jpav 万=1 area ds 土在土寸 i2=-万 E2 Os (Surface charge density) ()dsd(total charge inside plbox) o,=on…[月，-E2] 3.Back to Point Charge Above Ground Plane n=2 E =-qdiz 个 2e[x2+y2+d2]32 E2=0 2 At z=0: -6]6i.242 -qd -gd 2-[x2+y+d72[P+ r2=x2+y2 a-11ww-wzn 6.641,Electromagnetic Fields,Forces,and Motion Lecture 5 Prof.Markus Zahn Page 2 of 116.641, Electromagnetic Fields, Forces, and Motion Lecture 5 Prof. Markus Zahn Page 2 of 11 2. Gauss’s Law Boundary Condition 0 S V ε E da dV = ρ ∫ ∫ i v 0 00 s ( ) 11 22 S εεε E da E n E n dS dS = + =σ ∫ i ii v (total charge inside pillbox) s 0 1 2 σ= − nE E ⎡ ⎤ ⎣ ⎦ ε i 3. Back to Point Charge Above Ground Plane _ s 0 0 0z 1 2 z1 3 3 2 2 22 2 22 qd qd nE E i E E 2x y d 2r d − − σ= − = = = = ⎡ ⎤ ⎣ ⎦ π ++ π + ⎡ ⎤ ⎡⎤ ⎣ ⎦ ⎣⎦ ε εε i i = + 2 22 r xy ( ) 2 T ss y x r0 0 qd q z 0 dxdy rdrd 2 +∞ +∞ ∞ π = −∞ = −∞ = φ= − = = σ = σ φ= ∫ ∫ ∫∫ π ( ) 2 π 3 2 22 r 0 rdr r d ∞ = ⎡ ⎤ + ⎣ ⎦ ∫ At z=0: