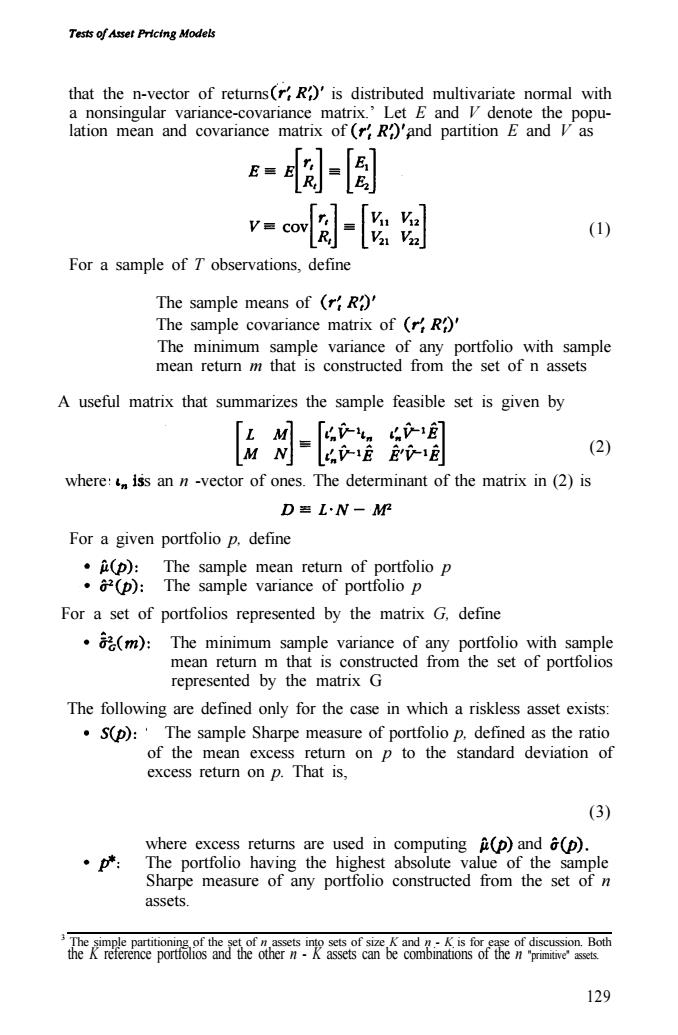
Tests of Asset Pricing Models that the n-vector of returns(R)'is distributed multivariate normal with a nonsingular variance-covariance matrix.'Let E and denote the popu- lation mean and covariance matrix of (R)'and partition E and V as E=E R E V=COV (1) For a sample of T observations,define The sample means of (rR)' The sample covariance matrix of (rR)' The minimum sample variance of any portfolio with sample mean return m that is constructed from the set of n assets A useful matrix that summarizes the sample feasible set is given by [&E器阊 (2) where:iss an n -vector of ones.The determinant of the matrix in (2)is D=L·N-MP For a given portfolio p.define .(p):The sample mean return of portfolio p .2(p):The sample variance of portfolio p For a set of portfolios represented by the matrix G,define .(m):The minimum sample variance of any portfolio with sample mean return m that is constructed from the set of portfolios represented by the matrix G The following are defined only for the case in which a riskless asset exists: S(p):The sample Sharpe measure of portfolio p.defined as the ratio of the mean excess return on p to the standard deviation of excess return on p.That is, (3) where excess returns are used in computing (p)and (p). ·* The portfolio having the highest absolute value of the sample Sharpe measure of any portfolio constructed from the set of n assets. The simple partitioning of the set of n assets into sets of size K and nK.is for ease of discussion.Both the K reference portfolios and the other n-K assets can be combinations of the n "primitive"assets. 129that the n-vector of returns is distributed multivariate normal with a nonsingular variance-covariance matrix.’ Let E and V denote the popu￾lation mean and covariance matrix of and partition E and V as (1) For a sample of T observations, define The sample means of The sample covariance matrix of The minimum sample variance of any portfolio with sample mean return m that is constructed from the set of n assets A useful matrix that summarizes the sample feasible set is given by (2) where is an n -vector of ones. The determinant of the matrix in (2) is For a given portfolio p, define The sample mean return of portfolio p The sample variance of portfolio p For a set of portfolios represented by the matrix G, define The minimum sample variance of any portfolio with sample mean return m that is constructed from the set of portfolios represented by the matrix G The following are defined only for the case in which a riskless asset exists: The sample Sharpe measure of portfolio p, defined as the ratio of the mean excess return on p to the standard deviation of excess return on p. That is, (3) where excess returns are used in computing and The portfolio having the highest absolute value of the sample Sharpe measure of any portfolio constructed from the set of n assets. 3 The simple partitioning of the set of n assets into sets of size K and n - K is for ease of discussion. Both the K reference portfolios and the other n - K assets can be combinations of the n "primitive" assets. 129