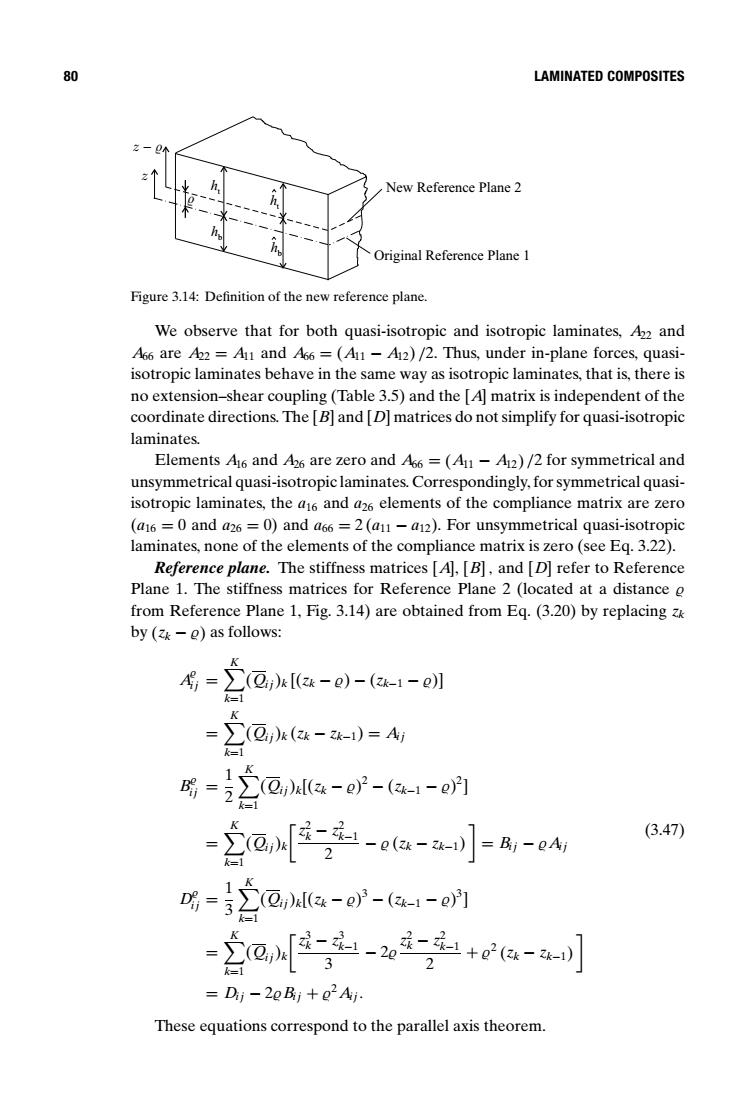
80 LAMINATED COMPOSITES New Reference Plane 2 Original Reference Plane 1 Figure 3.14:Definition of the new reference plane. We observe that for both quasi-isotropic and isotropic laminates,A2 and A66 are A2 =A11 and A66=(A11-A12)/2.Thus,under in-plane forces,quasi- isotropic laminates behave in the same way as isotropic laminates,that is,there is no extension-shear coupling(Table 3.5)and the [A]matrix is independent of the coordinate directions.The [B]and [D]matrices do not simplify for quasi-isotropic laminates. Elements A6 and A are zero and A66=(An-A2)/2 for symmetrical and unsymmetrical quasi-isotropic laminates.Correspondingly,for symmetrical quasi- isotropic laminates,the a16 and a26 elements of the compliance matrix are zero (a16=0 and a26 =0)and a66=2(a1-a12).For unsymmetrical quasi-isotropic laminates,none of the elements of the compliance matrix is zero (see Eq.3.22). Reference plane.The stiffness matrices [A],B],and [D]refer to Reference Plane 1.The stiffness matrices for Reference Plane 2 (located at a distance o from Reference Plane 1,Fig.3.14)are obtained from Eq.(3.20)by replacing by (z-)as follows: G=2a,kIa-e-a-1-®1 k=】 =20,ka-W=4 房-2O,hla-or-a1-o时1 -2a,[-ea-a小-a- (3.47) %=32a,s-e驴-(a- Dij-20Bj+o2Aj. These equations correspond to the parallel axis theorem.80 LAMINATED COMPOSITES New Reference Plane 2 Original Reference Plane 1  z z –  t h b h t h b h Figure 3.14: Definition of the new reference plane. We observe that for both quasi-isotropic and isotropic laminates, A22 and A66 are A22 = A11 and A66 = (A11 − A12) /2. Thus, under in-plane forces, quasi￾isotropic laminates behave in the same way as isotropic laminates, that is, there is no extension–shear coupling (Table 3.5) and the [A] matrix is independent of the coordinate directions. The [B] and [D] matrices do not simplify for quasi-isotropic laminates. Elements A16 and A26 are zero and A66 = (A11 − A12) /2 for symmetrical and unsymmetrical quasi-isotropic laminates. Correspondingly, for symmetrical quasi￾isotropic laminates, the a16 and a26 elements of the compliance matrix are zero (a16 = 0 and a26 = 0) and a66 = 2 (a11 − a12). For unsymmetrical quasi-isotropic laminates, none of the elements of the compliance matrix is zero (see Eq. 3.22). Reference plane. The stiffness matrices [A], [B] , and [D] refer to Reference Plane 1. The stiffness matrices for Reference Plane 2 (located at a distance  from Reference Plane 1, Fig. 3.14) are obtained from Eq. (3.20) by replacing zk by (zk − ) as follows: A i j = * K k=1 (Qi j)k [(zk − ) − (zk−1 − )] = * K k=1 (Qi j)k (zk − zk−1) = Ai j B i j = 1 2 * K k=1 (Qi j)k[(zk − ) 2 − (zk−1 − ) 2 ] = * K k=1 (Qi j)k z2 k − z2 k−1 2 −  (zk − zk−1) ! = Bi j − Ai j D i j = 1 3 * K k=1 (Qi j)k[(zk − ) 3 − (zk−1 − ) 3 ] = * K k=1 (Qi j)k z3 k − z3 k−1 3 − 2 z2 k − z2 k−1 2 + 2 (zk − zk−1) ! = Di j − 2Bi j + 2Ai j . (3.47) These equations correspond to the parallel axis theorem