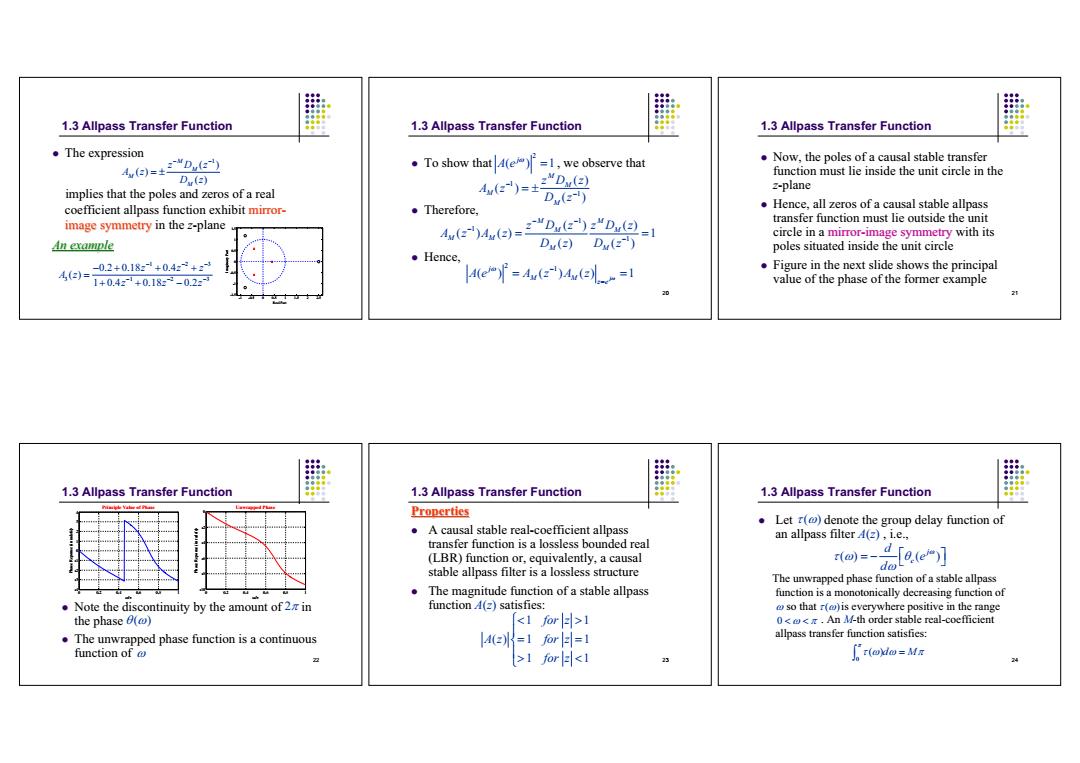
1.3 Allpass Transfer Function 1.3 Allpass Transfer Function 1.3 Allpass Transfer Function ·The expression 4e=±De .To show that)=1,we observe that Now,the poles of a causal stable transfer D(=) implies that the poles and zeros of a real 4(e)=t"De倒 function must lie inside the unit circle in the z-plane D(2-) coefficient allpass function exhibit mirror- 。Therefore, Hence,all zeros of a causal stable allpass 4u()()-Du(D( transfer function must lie outside the unit image symmetry in the z-plane circle in a mirror-image symmetry with its An example Du(z)Du(2) poles situated inside the unit circle 。Hence,, -0.2+0.182+0.422+23 4(e)=4u(=)4u()=1 Figure in the next slide shows the principal A()= 1+0.4z+0.1823-0.22 value of the phase of the former example 1.3 Allpass Transfer Function 1.3 Allpass Transfer Function 1.3 Allpass Transfer Function Properties Let r(@)denote the group delay function of A causal stable real-coefficient allpass an allpass filter A(),i.e., transfer function is a lossless bounded real (LBR)function or,equivalently,a causal (o)=-4[o.(] stable allpass filter is a lossless structure do The unwrapped phase function of a stable allpass The magnitude function of a stable allpass function is a monotonically decreasing function of ·Note the discontinuity by the amount of2πin function A(z)satisfies: so that r(@)is everywhere positive in the range the phase (@ <1 for >1 0<<.An M-th order stable real-coefficient The unwrapped phase function is a continuous 4(z=1r=1 allpass transfer function satisfies: function of >1 for <1 23 oylo-Ms19 -1 -0.5 0 0.5 1 1.5 2 2.5 -1.5 -1 -0.5 0 0.5 1 1.5 Real Part Imaginary Part 1.3 Allpass Transfer Function The expression implies that the poles and zeros of a real coefficient allpass function exhibit mirror￾image symmetry in the z-plane An example An example 1 ( ) ( ) ( ) M M M M z D z A z D z     1 23 3 1 23 0.2 0.18 0.4 ( ) 1 0.4 0.18 0.2 z z z A z z z z        20 1.3 Allpass Transfer Function To show that , we observe that Therefore, Hence, 1 1 ( ) ( ) ( ) M M M M z D z A z D z     2 () 1 j A e  1 1 1 ( ) () ( ) () 1 () ( ) M M M M M M M M z D z zD z Az Az Dz Dz       2 1 ( ) ( ) () 1 j j M M z e Ae A z A z     21 1.3 Allpass Transfer Function Now, the poles of a causal stable transfer function must lie inside the unit circle in the z-plane Hence, all zeros of a causal stable allpass transfer function must lie outside the unit circle in a mirror-image symmetry with its poles situated inside the unit circle Figure in the next slide shows the principal value of the phase of the former example 22 1.3 Allpass Transfer Function Note the discontinuity by the amount of in the phase The unwrapped phase function is a continuous function of 2  ( ) 0 0.2 0.4 0.6 0.8 1 -4 -3 -2 -1 0 1 2 3 4 / Phase Reponse (in rads/s) Principle Value of Phase 0 0.2 0.4 0.6 0.8 1 -10 -8 -6 -4 -2 0 / Phase Reponse (in rads/s) Unwrapped Phase 23 1.3 Allpass Transfer Function Properties Properties A causal stable real-coefficient allpass transfer function is a lossless bounded real (LBR) function or, equivalently, a causal stable allpass filter is a lossless structure The magnitude function of a stable allpass function A(z) satisfies: 1 1 () 1 1 1 1 for z A z for z for z        24 1.3 Allpass Transfer Function Let t(w) denote the group delay function of an allpass filter A(z) , i.e., The unwrapped phase function of a stable allpass function is a monotonically decreasing function of w so that t(w) is everywhere positive in the range . An M-th order stable real-coefficient allpass transfer function satisfies:  ( ) () ( ) j c d e d         0    ( ) 0 ( )d M