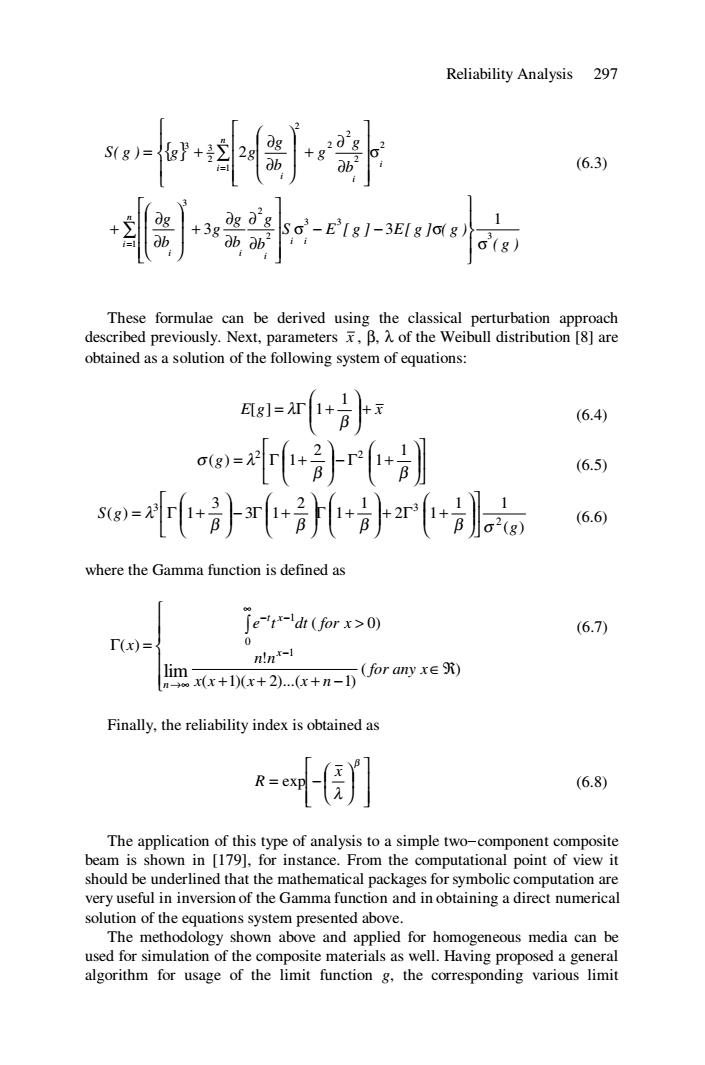
Reliability Analysis 297 2 S8)={ + 28 +8 (6.3) dg ag∂g +38 ab,ab, So-E'[g ]-3E[g lo(g) (g) These formulae can be derived using the classical perturbation approach described previously.Next,parameters B.A of the Weibull distribution [8]are obtained as a solution of the following system of equations: (6.4) 計r (6.5) 5()- +}2r小司 (6.6) where the Gamma function is defined as Jerldr (for x>0 (6.7) T(x)= 0 nn-1 lim (for any xe∈) nex(x+1)x+2)(x+n-1) Finally,the reliability index is obtained as ej (6.8) The application of this type of analysis to a simple two-component composite beam is shown in [179],for instance.From the computational point of view it should be underlined that the mathematical packages for symbolic computation are very useful in inversion of the Gamma function and in obtaining a direct numerical solution of the equations system presented above. The methodology shown above and applied for homogeneous media can be used for simulation of the composite materials as well.Having proposed a general algorithm for usage of the limit function g,the corresponding various limitReliability Analysis 297 { } ( g ) S E [ g ] E[ g ] ( g ) b g b g g b g b g g b g S( g ) g g n i i i i i i n i i i i 3 1 3 3 2 2 3 1 2 2 2 2 2 2 3 3 1 3 3 2 ⎪ σ ⎭ ⎪ ⎬ ⎫ σ − − σ ⎥ ⎥ ⎥ ⎦ ⎤ ⎢ ⎢ ⎢ ⎣ ⎡ ∂ ∂ ∂ ∂ + ⎟ ⎟ ⎠ ⎞ ⎜ ⎜ ⎝ ⎛ ∂ ∂ + ⎪ ⎩ ⎪ ⎨ ⎧ σ ⎥ ⎥ ⎥ ⎦ ⎤ ⎢ ⎢ ⎢ ⎣ ⎡ ∂ ∂ + ⎟ ⎟ ⎠ ⎞ ⎜ ⎜ ⎝ ⎛ ∂ ∂ = + ∑ ∑ = = (6.3) These formulae can be derived using the classical perturbation approach described previously. Next, parameters x , β, λ of the Weibull distribution [8] are obtained as a solution of the following system of equations: E g + x ⎟ ⎟ ⎠ ⎞ ⎜ ⎜ ⎝ ⎛ = Γ + β λ 1 [ ] 1 (6.4) ⎥ ⎦ ⎤ ⎢ ⎣ ⎡ ⎟ ⎟ ⎠ ⎞ ⎜ ⎜ ⎝ ⎛ − Γ + ⎟ ⎟ ⎠ ⎞ ⎜ ⎜ ⎝ ⎛ = Γ + β β σ λ 1 1 2 ( ) 1 2 2 g (6.5) ( ) 1 1 2 1 1 1 2 3 1 3 ( ) 1 2 3 3 g S g β β β β σ λ ⎥ ⎦ ⎤ ⎢ ⎣ ⎡ ⎟ ⎟ ⎠ ⎞ ⎜ ⎜ ⎝ ⎛ + Γ + ⎟ ⎟ ⎠ ⎞ ⎜ ⎜ ⎝ ⎛ Γ + ⎟ ⎟ ⎠ ⎞ ⎜ ⎜ ⎝ ⎛ − Γ + ⎟ ⎟ ⎠ ⎞ ⎜ ⎜ ⎝ ⎛ = Γ + (6.6) where the Gamma function is defined as ⎪ ⎪ ⎩ ⎪ ⎪ ⎨ ⎧ ∈ℜ + + + − > Γ = − →∞ ∞ − − ∫ ( ) ( 1)( 2)...( 1) ! ( 0) ( ) 1 0 1 lim for any x x x x x n n n e t dt for x x x n t x (6.7) Finally, the reliability index is obtained as ⎥ ⎥ ⎦ ⎤ ⎢ ⎢ ⎣ ⎡ ⎟ ⎠ ⎞ ⎜ ⎝ ⎛ = − β λ x R exp (6.8) The application of this type of analysis to a simple two-component composite beam is shown in [179], for instance. From the computational point of view it should be underlined that the mathematical packages for symbolic computation are very useful in inversion of the Gamma function and in obtaining a direct numerical solution of the equations system presented above. The methodology shown above and applied for homogeneous media can be used for simulation of the composite materials as well. Having proposed a general algorithm for usage of the limit function g, the corresponding various limit