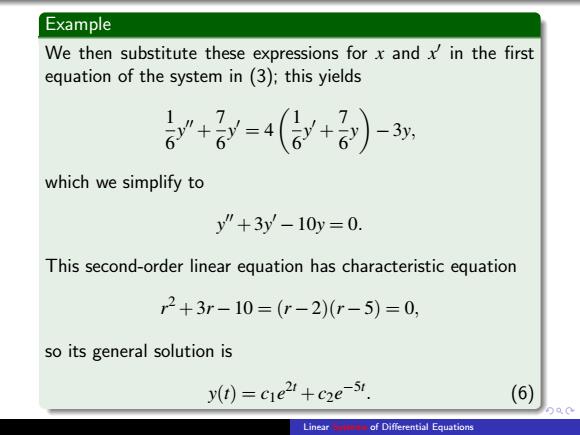
Example We then substitute these expressions for x andx in the first equation of the system in(3);this yields +名=4（信日+）- which we simplify to y"+3y-10y=0. This second-order linear equation has characteristic equation 2+3r-10=(r-2)(r-5)=0, so its general solution is y(t)=cle2+c2e-51. (6) Linear of Differential EquationsExample We then substitute these expressions for x and x 0 in the first equation of the system in (3); this yields 1 6 y 00 + 7 6 y 0 = 4  1 6 y 0 + 7 6 y  −3y, which we simplify to y 00 +3y 0 −10y = 0. This second-order linear equation has characteristic equation r 2 +3r −10 = (r −2)(r −5) = 0, so its general solution is y(t) = c1e 2t +c2e −5t . (6) Linear Systems of Differential Equations