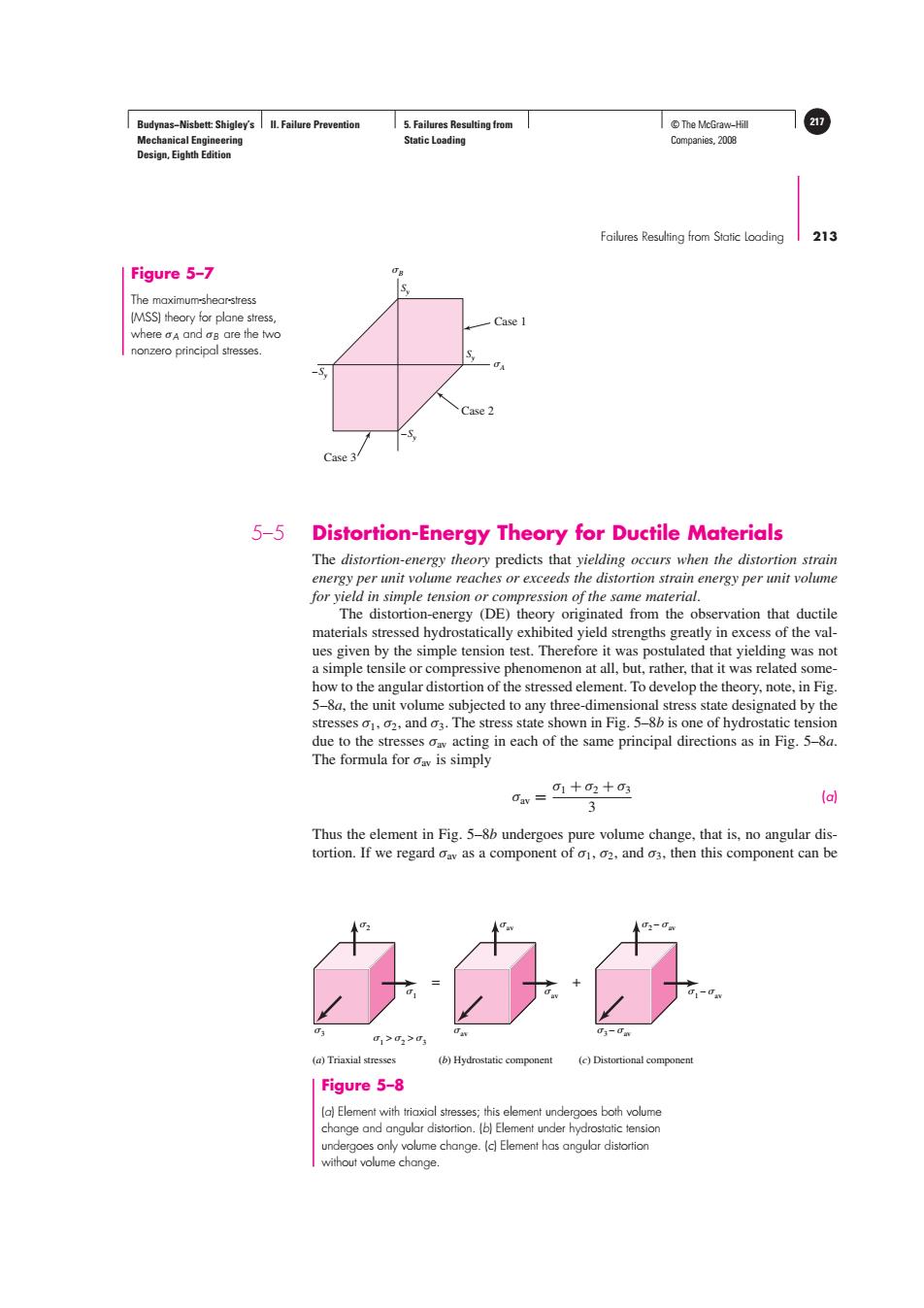
Budynas-Nisbett:Shigley's ll.Failure Prevention 5.Failures Resulting from T©The McGraw-Hill 21m Mechanical Engineering Static Loading Companies,2008 Design,Eighth Edition Failures Resulting from Static Loading 213 Figure 5-7 B The maximum-shear-stress MSS)theory for plane stress, where aA and ag are the two nonzero principal stresses. Case 2 Case 3 5-5 Distortion-Energy Theory for Ductile Materials The distortion-energy theory predicts that yielding occurs when the distortion strain energy per unit volume reaches or exceeds the distortion strain energy per unit volume for yield in simple tension or compression of the same material. The distortion-energy (DE)theory originated from the observation that ductile materials stressed hydrostatically exhibited yield strengths greatly in excess of the val- ues given by the simple tension test.Therefore it was postulated that yielding was not a simple tensile or compressive phenomenon at all,but,rather,that it was related some- how to the angular distortion of the stressed element.To develop the theory,note,in Fig. 5-8a,the unit volume subjected to any three-dimensional stress state designated by the stresses o1.02,and o3.The stress state shown in Fig.5-8b is one of hydrostatic tension due to the stresses ov acting in each of the same principal directions as in Fig.5-8a. The formula for oav is simply oav =1+02+03 (a) 3 Thus the element in Fig.5-8b undergoes pure volume change,that is,no angular dis- tortion.If we regard oav as a component of o1,02,and o3,then this component can be I>02>0 (a Triaxial stresses (b)Hydrostatic component (c)Distortional componen Figure 5-8 (a)Element with triaxial stresses;this element undergoes both volume change and angular distortion.(b)Element under hydrostatic tension undergoes only volume change.(c]Element has angular distortion without volume change.Budynas−Nisbett: Shigley’s Mechanical Engineering Design, Eighth Edition II. Failure Prevention 5. Failures Resulting from Static Loading © The McGraw−Hill 217 Companies, 2008 Failures Resulting from Static Loading 213 5–5 Distortion-Energy Theory for Ductile Materials The distortion-energy theory predicts that yielding occurs when the distortion strain energy per unit volume reaches or exceeds the distortion strain energy per unit volume for yield in simple tension or compression of the same material. The distortion-energy (DE) theory originated from the observation that ductile materials stressed hydrostatically exhibited yield strengths greatly in excess of the val￾ues given by the simple tension test. Therefore it was postulated that yielding was not a simple tensile or compressive phenomenon at all, but, rather, that it was related some￾how to the angular distortion of the stressed element. To develop the theory, note, in Fig. 5–8a, the unit volume subjected to any three-dimensional stress state designated by the stresses σ1, σ2, and σ3. The stress state shown in Fig. 5–8b is one of hydrostatic tension due to the stresses σav acting in each of the same principal directions as in Fig. 5–8a. The formula for σav is simply σav = σ1 + σ2 + σ3 3 (a) Thus the element in Fig. 5–8b undergoes pure volume change, that is, no angular dis￾tortion. If we regard σav as a component of σ1, σ2, and σ3, then this component can be B A –Sy Sy Sy –Sy Case 2 Case 3 Case 1 Figure 5–7 The maximum-shear-stress (MSS) theory for plane stress, where σA and σB are the two nonzero principal stresses. Figure 5–8 (a) Element with triaxial stresses; this element undergoes both volume change and angular distortion. (b) Element under hydrostatic tension undergoes only volume change. (c) Element has angular distortion without volume change. 3 1 > 2 > 3 2 1 (a) Triaxial stresses (b) Hydrostatic component 3 – av 2 – av 1 – av (c) Distortional component av av av = +