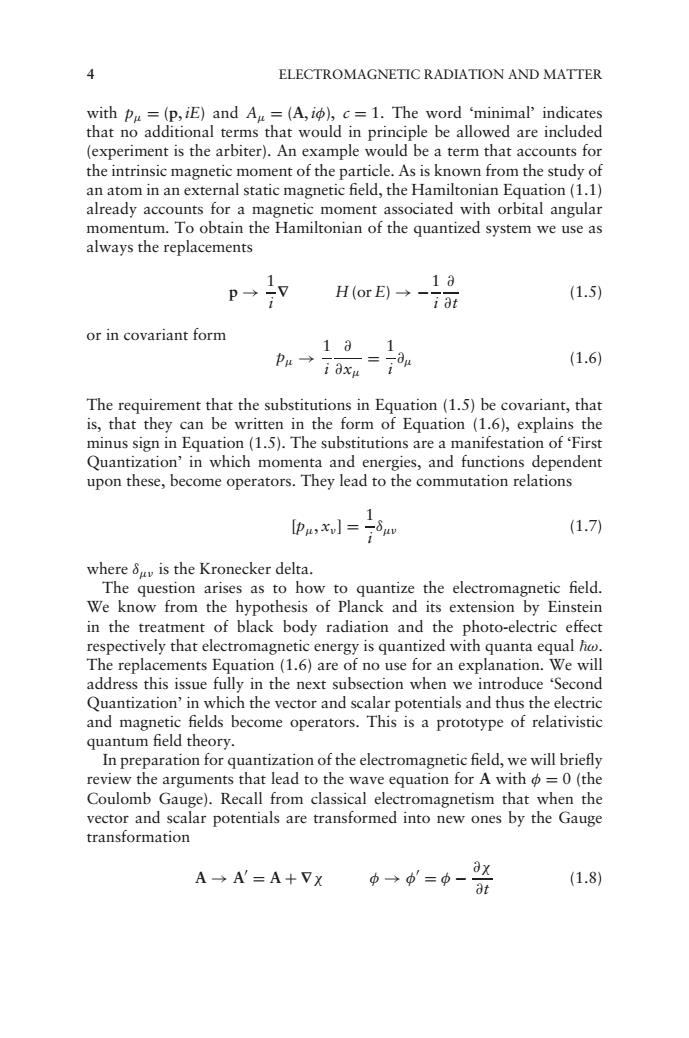
4 ELECTROMAGNETIC RADIATION AND MATTER with Pu=(p,iE)and A=(A,i),c=1.The word 'minimal'indic cate that no additional terms that would in principle be allowed are included (experiment is the arbiter).An example would be a term that accounts for the intrinsic magnetic moment of the particle.As is known from the study of etic field.the Hamilt ian Fo a n nagnetic me ment a sociated momentum.To obtain the Hamiltonian of the quantized system we use as always the replacements p→ Ha固+-品 (1.5) or in covariant form 18 (1.6 The requirement that the substitutions in Equation(1.5)be covariant,that is,that they can be written in the form of Equation (1.6),explains the minus sign in Equation(1.5).The substitutions are a manifestation of 'First Quantization'in which momenta and energies,and functions dependent upon these,become operators.They lead to the commutation relations (1.7刀 where is the Kronecker delta. The stion a ses sas to how to We know from the s of p lanck and tein in the treatment of black body radiation and the photo-electric effect respectively that electromagnetic energy is quantized with quanta equal ho. The replacements Equation(1.6)are of no use for an explanation.We will address this issue fully in the next subsection when we introduce'Second which the vector and scaar poten ntials and thus the elec and magn fields become operators.This is a prototype of relativistic In preparation for quantization of the electromagnetic field,we will briefly review the arguments that lead to the wave equation for A with =0(the Coulomb Gauge).Recall from classical electromagnetism that when the vector and scalar r potentials are transformed into new ones by the Gauge transformation A→A'=A+VX 中→=0-船 (1.8) 4 ELECTROMAGNETIC RADIATION AND MATTER with pµ = (p, iE) and Aµ = (A, iφ), c = 1. The word ‘minimal’ indicates that no additional terms that would in principle be allowed are included (experiment is the arbiter). An example would be a term that accounts for the intrinsic magnetic moment of the particle. As is known from the study of an atom in an external static magnetic field, the Hamiltonian Equation (1.1) already accounts for a magnetic moment associated with orbital angular momentum. To obtain the Hamiltonian of the quantized system we use as always the replacements p → 1 i ∇ H (or E) → −1 i ∂ ∂t (1.5) or in covariant form pµ → 1 i ∂ ∂xµ = 1 i ∂µ (1.6) The requirement that the substitutions in Equation (1.5) be covariant, that is, that they can be written in the form of Equation (1.6), explains the minus sign in Equation (1.5). The substitutions are a manifestation of ‘First Quantization’ in which momenta and energies, and functions dependent upon these, become operators. They lead to the commutation relations [pµ, xν ] = 1 i δµν (1.7) where δµν is the Kronecker delta. The question arises as to how to quantize the electromagnetic field. We know from the hypothesis of Planck and its extension by Einstein in the treatment of black body radiation and the photo-electric effect respectively that electromagnetic energy is quantized with quanta equal
ω. The replacements Equation (1.6) are of no use for an explanation. We will address this issue fully in the next subsection when we introduce ‘Second Quantization’ in which the vector and scalar potentials and thus the electric and magnetic fields become operators. This is a prototype of relativistic quantum field theory. In preparation for quantization of the electromagnetic field, we will briefly review the arguments that lead to the wave equation for A with φ = 0 (the Coulomb Gauge). Recall from classical electromagnetism that when the vector and scalar potentials are transformed into new ones by the Gauge transformation A → A = A + ∇χ φ → φ = φ − ∂χ ∂t (1.8)