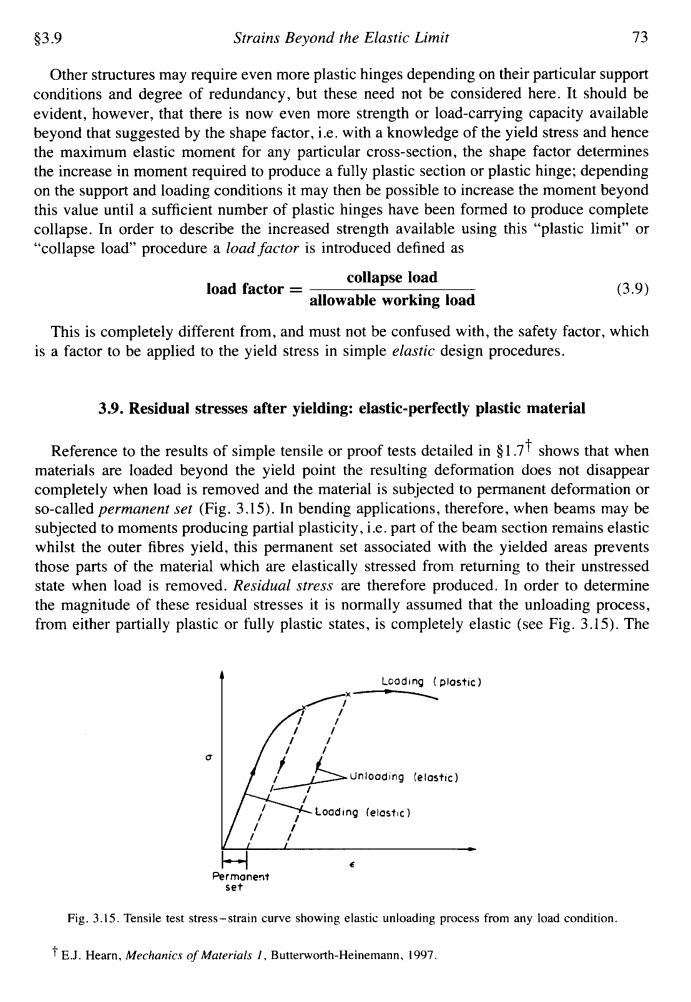
$3.9 Strains Beyond the Elastic Limit 73 Other structures may require even more plastic hinges depending on their particular support conditions and degree of redundancy,but these need not be considered here.It should be evident,however,that there is now even more strength or load-carrying capacity available beyond that suggested by the shape factor,i.e.with a knowledge of the yield stress and hence the maximum elastic moment for any particular cross-section,the shape factor determines the increase in moment required to produce a fully plastic section or plastic hinge:depending on the support and loading conditions it may then be possible to increase the moment beyond this value until a sufficient number of plastic hinges have been formed to produce complete collapse.In order to describe the increased strength available using this "plastic limit"or "collapse load"procedure a load factor is introduced defined as load factor= collapse load (3.9) allowable working load This is completely different from,and must not be confused with,the safety factor,which is a factor to be applied to the yield stress in simple elastic design procedures. 3.9.Residual stresses after yielding:elastic-perfectly plastic material Reference to the results of simple tensile or proof tests detailed in $1.7 shows that when materials are loaded beyond the yield point the resulting deformation does not disappear completely when load is removed and the material is subjected to permanent deformation or so-called permanent set (Fig.3.15).In bending applications,therefore,when beams may be subjected to moments producing partial plasticity,i.e.part of the beam section remains elastic whilst the outer fibres yield,this permanent set associated with the yielded areas prevents those parts of the material which are elastically stressed from returning to their unstressed state when load is removed.Residual stress are therefore produced.In order to determine the magnitude of these residual stresses it is normally assumed that the unloading process, from either partially plastic or fully plastic states,is completely elastic (see Fig.3.15).The Lcoding plastic) Unlooding(elostic)】 -Loading(elastic】 Permanent set Fig.3.15.Tensile test stress-strain curve showing elastic unloading process from any load condition. fE.J.Hearn,Mechanics of Materials 1,Butterworth-Heinemann.1997.53.9 Strains Beyond the Elastic Limit 73 Other structures may require even more plastic hinges depending on their particular support conditions and degree of redundancy, but these need not be considered here. It should be evident, however, that there is now even more strength or load-carrying capacity available beyond that suggested by the shape factor, i.e. with a knowledge of the yield stress and hence the maximum elastic moment for any particular cross-section, the shape factor determines the increase in moment required to produce a fully plastic section or plastic hinge; depending on the support and loading conditions it may then be possible to increase the moment beyond this value until a sufficient number of plastic hinges have been formed to produce complete collapse. In order to describe the increased strength available using this “plastic limit” or “collapse load” procedure a load factor is introduced defined as collapse load allowable working load load factor = (3.9) This is completely different from, and must not be confused with, the safety factor, which is a factor to be applied to the yield stress in simple elastic design procedures. 3.9. Residual stresses after yielding: elastic-perfectly plastic material Reference to the results of simple tensile or proof tests detailed in 8 1 .7t shows that when materials are loaded beyond the yield point the resulting deformation does not disappear completely when load is removed and the material is subjected to permanent deformation or so-called permanent set (Fig. 3.15). In bending applications, therefore, when beams may be subjected to moments producing partial plasticity, i.e. part of the beam section remains elastic whilst the outer fibres yield, this permanent set associated with the yielded areas prevents those parts of the material which are elastically stressed from returning to their unstressed state when load is removed. Residual stress are therefore produced. In order to determine the magnitude of these residual stresses it is normally assumed that the unloading process, from either partially plastic or fully plastic states, is completely elastic (see Fig. 3.15). The 4 U c H c Permanent set Fig. 3.15. Tensile test stress-strain curve showing elastic unloading process from any load condition. t E.J. Hearn, Mechanics of Materials I, Buttenvorth-Heinemann. 1997