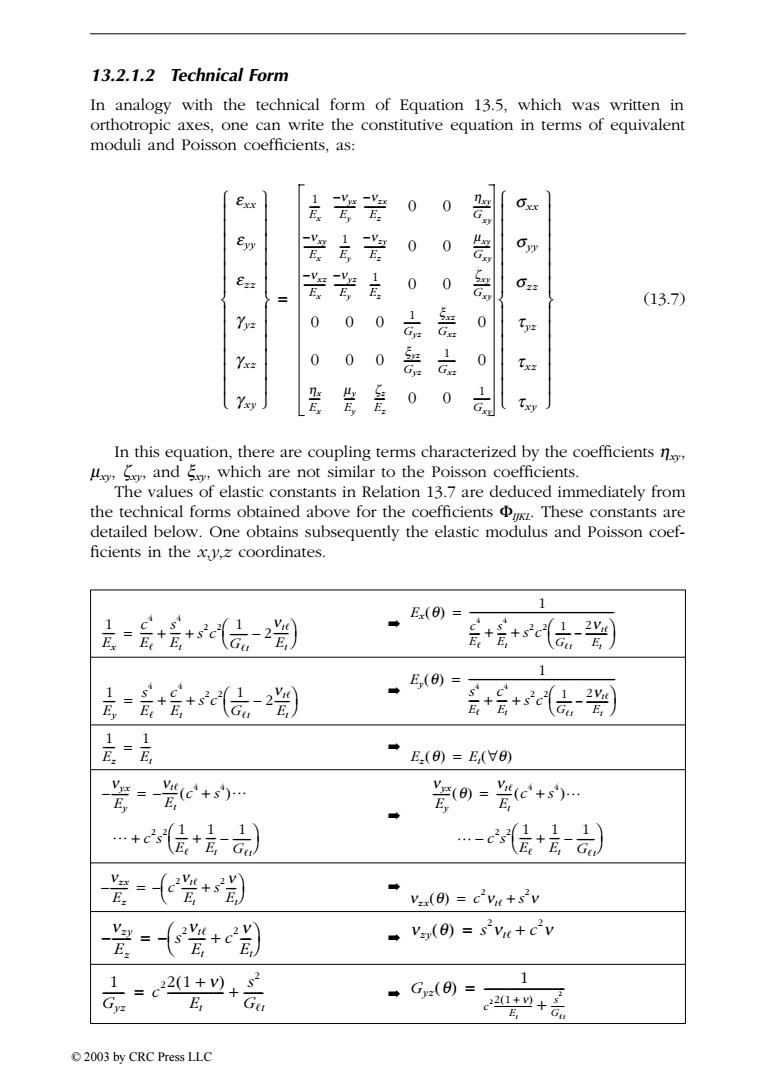
13.2.1.2 Technical Form In analogy with the technical form of Equation 13.5,which was written in orthotropic axes,one can write the constitutive equation in terms of equivalent moduli and Poisson coefficients,as: Exx 尝 0 0 Oxx 苦 尝 0 0 会 Oyy Ezz 0 0 02z (13.7) 0 00 器 0 a 0 0 0 兽 0 txz 兰 0 0 G In this equation,there are coupling terms characterized by the coefficients no oand which are not similar to the Poisson coefficients. The values of elastic constants in Relation 13.7 are deduced immediately from the technical forms obtained above for the coefficients y.These constants are detailed below.One obtains subsequently the elastic modulus and Poisson coef- ficients in the x,y,z coordinates. E(0= ++品曾 、,()= 1 ++尝) E,()=E,() 20,=管c+ +层+馆品) -位+言) 完-{党+) v=(0)=c'vn+sv 空=尝+) v(0)=s'vn+c'v + 1 G()= E 220+业+ E 2003 by CRC Press LLC13.2.1.2 Technical Form In analogy with the technical form of Equation 13.5, which was written in orthotropic axes, one can write the constitutive equation in terms of equivalent moduli and Poisson coefficients, as: (13.7) In this equation, there are coupling terms characterized by the coefficients hxy, mxy, zxy, and xxy, which are not similar to the Poisson coefficients. The values of elastic constants in Relation 13.7 are deduced immediately from the technical forms obtained above for the coefficients FIJKL. These constants are detailed below. One obtains subsequently the elastic modulus and Poisson coef- ficients in the x,y,z coordinates. ➡ ➡ ➡ ➡ ➡ ➡ ➡ exx e yy e zz g yz g xz Ó g xy ˛ Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ì ˝ Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ï ¸ 1 Ex ----- –nyx Ey ---------- –nzx Ez ---------- 0 0 hxy Gxy -------- –nxy Ex ---------- 1 Ey ---- –nzy Ez ---------- 0 0 mxy Gxy -------- –nxz Ex ---------- –nyz Ey ---------- 1 Ez ---- 0 0 zxy Gxy -------- 0 00 1 Gyz -------- xxz Gxz -------- 0 0 00 xyz Gyz -------- 1 Gxz -------- 0 hx Ex ----- my Ey ----- z z Ez ---- 0 0 1 Gxy -------- sxx syy szz t yz txz Ó txy ˛ Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ì ˝ Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ô Ï ¸ = 1 Ex ----- c 4 E
---- s 4 Et ---- s 2 c 2 1 G
t ------- 2 nt
Et – ------ Ë ¯ Ê ˆ = + + Ex( ) q 1 c 4 E
----- s 4 Et ---- s 2 c 2 1 G
t ------- 2nt
Et – ---------- Ë ¯ Ê ˆ + + = --------------------------------------------------------- 1 Ey ---- s 4 E
---- c 4 Et ---- s 2 c 2 1 G
t ------- 2 nt
Et – ------ Ë ¯ Ê ˆ = + + Ey( ) q 1 s 4 E
----- c 4 Et ----- s 2 c 2 1 G
t ------- 2nt
Et – ---------- Ë ¯ Ê ˆ + + = ---------------------------------------------------------- 1 Ez ---- 1 Et = ---- Ez( ) q = Et( ) "q nyx Ey –------ nt
Et ------ c 4 s 4 = – ( ) + º º c 2 s 2 1 E
---- 1 Et ---- 1 G
t + – ------- Ë ¯ Ê ˆ + nyx Ey ------( ) q nt
Et ------ c 4 s 4 = ( ) + º º c 2 s 2 1 E
---- 1 Et ---- 1 G
t + – ------- Ë ¯ Ê ˆ – nzx Ez –------- c 2nt
Et ------ s 2 n Et + ---- Ë ¯ Ê ˆ = – nzx( ) q c 2 nt
s 2 = + n nzy Ez –------ s 2nt
Et ------ c 2 n Et + ---- Ë ¯ Ê ˆ = – nzy( ) q s 2 nt
c 2 = + n 1 Gyz ------- c 2 2 1( ) + n Et -------------------- s 2 G
t = + ------- Gyz( ) q 1 c 2 2 1( ) + n Et -------------------- s 2 G
t + ------- = ---------------------------- TX846_Frame_C13 Page 269 Monday, November 18, 2002 12:29 PM © 2003 by CRC Press LLC