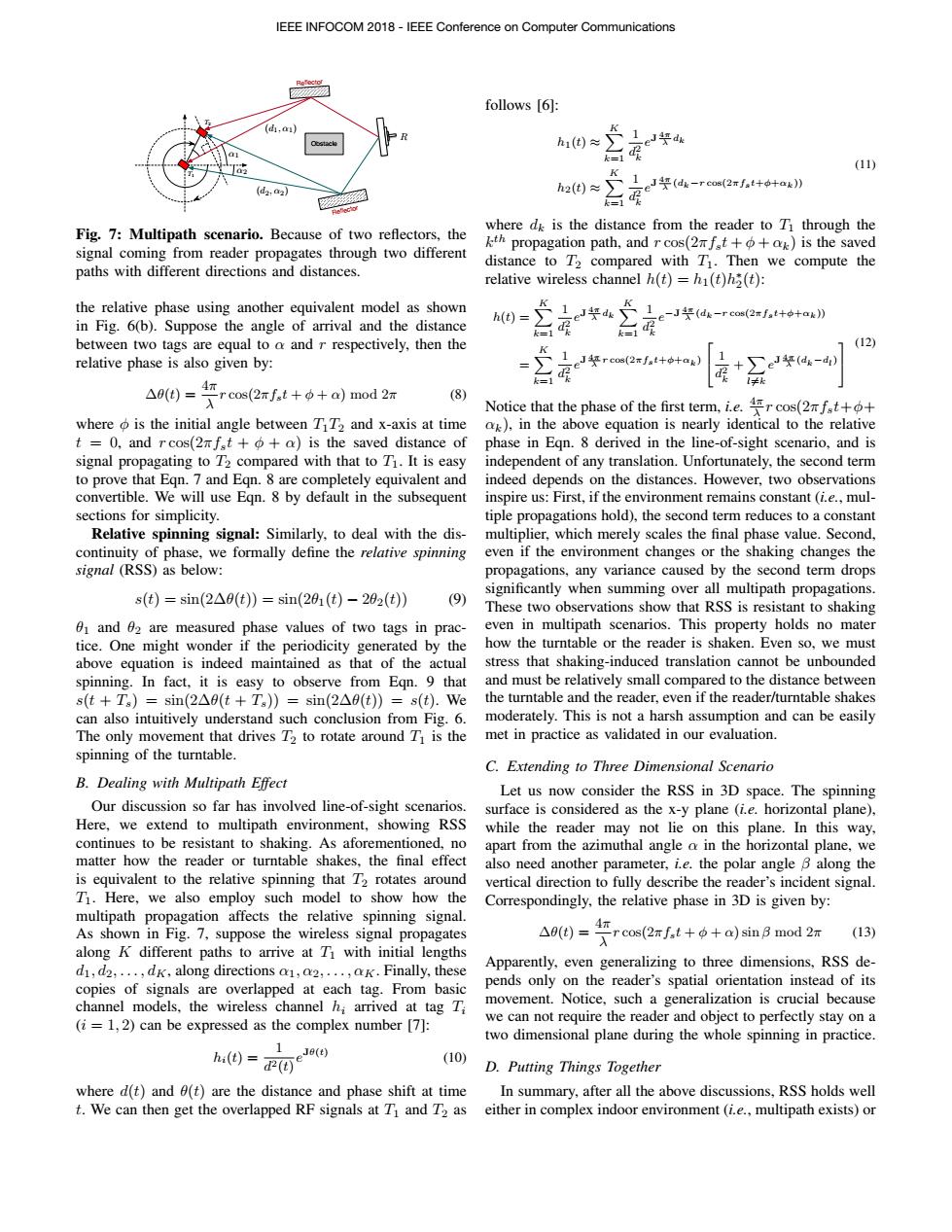
IEEE INFOCOM 2018-IEEE Conference on Computer Communications follows [6]: 1的≈5c奖 (11) (,a2 h2(t)≈∑ 9 where d is the distance from the reader to T through the Fig.7:Multipath scenario.Because of two reflectors,the signal coming from reader propagates through two different kth propagation path,and r cos(2f+)is the saved distance to T2 compared with T.Then we compute the paths with different directions and distances. relative wireless channel h(t)=h1(t)h(t): the relative phase using another equivalent model as shown K 1eJd 1 -J经(dk-rcos(2xfat+中+ak) in Fig.6(b).Suppose the angle of arrival and the distance h(t)= between two tags are equal to a and r respectively,then the (12) K relative phase is also given by: 马9reos(2mt++a) +e (dx-dp) ≠k △9④=rcos(2mft++amod2x (8) Notice that the phase of the first term,i.e.r cos(2ft where o is the initial angle between TT2 and x-axis at time )in the above equation is nearly identical to the relative t =0,and rcos(2mft++a)is the saved distance of phase in Eqn.8 derived in the line-of-sight scenario,and is signal propagating to T2 compared with that to T1.It is easy independent of any translation.Unfortunately,the second term to prove that Eqn.7 and Eqn.8 are completely equivalent and indeed depends on the distances.However,two observations convertible.We will use Egn.8 by default in the subsequent inspire us:First,if the environment remains constant (i.e.,mul- sections for simplicity. tiple propagations hold),the second term reduces to a constant Relative spinning signal:Similarly,to deal with the dis-multiplier,which merely scales the final phase value.Second. continuity of phase,we formally define the relative spinning even if the environment changes or the shaking changes the signal (RSS)as below: propagations,any variance caused by the second term drops significantly when summing over all multipath propagations. s(t)=sin(2△9t)=sin(291(t)-202(t) (9) These two observations show that RSS is resistant to shaking 1 and 02 are measured phase values of two tags in prac- even in multipath scenarios.This property holds no mater tice.One might wonder if the periodicity generated by the how the turntable or the reader is shaken.Even so,we must above equation is indeed maintained as that of the actual stress that shaking-induced translation cannot be unbounded spinning.In fact,it is easy to observe from Eqn.9 that and must be relatively small compared to the distance between s(t+T)=sin(20(t+T.))=sin(2A0(t))=s(t).We the turntable and the reader,even if the reader/turntable shakes can also intuitively understand such conclusion from Fig.6. moderately.This is not a harsh assumption and can be easily The only movement that drives T2 to rotate around Ti is the met in practice as validated in our evaluation. spinning of the turntable. C.Extending to Three Dimensional Scenario B.Dealing with Multipath Effect Let us now consider the RSS in 3D space.The spinning Our discussion so far has involved line-of-sight scenarios. surface is considered as the x-y plane (i.e.horizontal plane). Here,we extend to multipath environment,showing RSS while the reader may not lie on this plane.In this way, continues to be resistant to shaking.As aforementioned,no apart from the azimuthal angle o in the horizontal plane,we matter how the reader or turntable shakes,the final effect also need another parameter,i.e.the polar angle B along the is equivalent to the relative spinning that 72 rotates around vertical direction to fully describe the reader's incident signal. T1.Here,we also employ such model to show how the Correspondingly,the relative phase in 3D is given by: multipath propagation affects the relative spinning signal. As shown in Fig.7,suppose the wireless signal propagates △0)=4红7 cos(2ft++a)sin B mod 2 (13) along K different paths to arrive at Ti with initial lengths di,d2,...,dk,along directions 1,a2,...,aK.Finally,these Apparently,even generalizing to three dimensions,RSS de- copies of signals are overlapped at each tag.From basic pends only on the reader's spatial orientation instead of its channel models,the wireless channel h;arrived at tag Ti movement.Notice,such a generalization is crucial because (i=1,2)can be expressed as the complex number [7]: we can not require the reader and object to perfectly stay on a two dimensional plane during the whole spinning in practice. h() (10) D.Putting Things Together where d(t)and 0(t)are the distance and phase shift at time In summary,after all the above discussions,RSS holds well t.We can then get the overlapped RF signals at T and T2 as either in complex indoor environment(i.e.,multipath exists)orT1 T2 R Obstacle (d1, ↵1) (d2, ↵2) Reflector Reflector ↵1 ↵2 Fig. 7: Multipath scenario. Because of two reflectors, the signal coming from reader propagates through two different paths with different directions and distances. the relative phase using another equivalent model as shown in Fig. 6(b). Suppose the angle of arrival and the distance between two tags are equal to ↵ and r respectively, then the relative phase is also given by: ∆✓(t) = 4⇡ λ r cos(2⇡fst + φ + ↵) mod 2⇡ (8) where φ is the initial angle between T1T2 and x-axis at time t = 0, and r cos(2⇡fst + φ + ↵) is the saved distance of signal propagating to T2 compared with that to T1. It is easy to prove that Eqn. 7 and Eqn. 8 are completely equivalent and convertible. We will use Eqn. 8 by default in the subsequent sections for simplicity. Relative spinning signal: Similarly, to deal with the dis￾continuity of phase, we formally define the relative spinning signal (RSS) as below: s(t) = sin(2∆✓(t)) = sin(2✓1(t) − 2✓2(t)) (9) ✓1 and ✓2 are measured phase values of two tags in prac￾tice. One might wonder if the periodicity generated by the above equation is indeed maintained as that of the actual spinning. In fact, it is easy to observe from Eqn. 9 that s(t + Ts) = sin(2∆✓(t + Ts)) = sin(2∆✓(t)) = s(t). We can also intuitively understand such conclusion from Fig. 6. The only movement that drives T2 to rotate around T1 is the spinning of the turntable. B. Dealing with Multipath Effect Our discussion so far has involved line-of-sight scenarios. Here, we extend to multipath environment, showing RSS continues to be resistant to shaking. As aforementioned, no matter how the reader or turntable shakes, the final effect is equivalent to the relative spinning that T2 rotates around T1. Here, we also employ such model to show how the multipath propagation affects the relative spinning signal. As shown in Fig. 7, suppose the wireless signal propagates along K different paths to arrive at T1 with initial lengths d1, d2,...,dK, along directions ↵1, ↵2,..., ↵K. Finally, these copies of signals are overlapped at each tag. From basic channel models, the wireless channel hi arrived at tag Ti (i = 1, 2) can be expressed as the complex number [7]: hi(t) = 1 d2(t) e J✓(t) (10) where d(t) and ✓(t) are the distance and phase shift at time t. We can then get the overlapped RF signals at T1 and T2 as follows [6]: h1(t) ⇡ XK k=1 1 d2 k eJ 4⇡ λ dk h2(t) ⇡ XK k=1 1 d2 k eJ 4⇡ λ (dk−r cos(2⇡fst+φ+↵k)) (11) where dk is the distance from the reader to T1 through the kth propagation path, and r cos(2⇡fst + φ + ↵k) is the saved distance to T2 compared with T1. Then we compute the relative wireless channel h(t) = h1(t)h⇤ 2(t): h(t) = XK k=1 1 d2 k eJ 4⇡ λ dk XK k=1 1 d2 k e−J 4⇡ λ (dk−r cos(2⇡fst+φ+↵k)) = XK k=1 1 d2 k eJ 4⇡ λ r cos(2⇡fst+φ+↵k) 2 4 1 d2 k +X l6=k eJ 4⇡ λ (dk−dl) 3 5 (12) Notice that the phase of the first term, i.e. 4⇡ λ r cos(2⇡fst+φ+ ↵k), in the above equation is nearly identical to the relative phase in Eqn. 8 derived in the line-of-sight scenario, and is independent of any translation. Unfortunately, the second term indeed depends on the distances. However, two observations inspire us: First, if the environment remains constant (i.e., mul￾tiple propagations hold), the second term reduces to a constant multiplier, which merely scales the final phase value. Second, even if the environment changes or the shaking changes the propagations, any variance caused by the second term drops significantly when summing over all multipath propagations. These two observations show that RSS is resistant to shaking even in multipath scenarios. This property holds no mater how the turntable or the reader is shaken. Even so, we must stress that shaking-induced translation cannot be unbounded and must be relatively small compared to the distance between the turntable and the reader, even if the reader/turntable shakes moderately. This is not a harsh assumption and can be easily met in practice as validated in our evaluation. C. Extending to Three Dimensional Scenario Let us now consider the RSS in 3D space. The spinning surface is considered as the x-y plane (i.e. horizontal plane), while the reader may not lie on this plane. In this way, apart from the azimuthal angle ↵ in the horizontal plane, we also need another parameter, i.e. the polar angle β along the vertical direction to fully describe the reader’s incident signal. Correspondingly, the relative phase in 3D is given by: ∆✓(t) = 4⇡ λ r cos(2⇡fst + φ + ↵) sin β mod 2⇡ (13) Apparently, even generalizing to three dimensions, RSS de￾pends only on the reader’s spatial orientation instead of its movement. Notice, such a generalization is crucial because we can not require the reader and object to perfectly stay on a two dimensional plane during the whole spinning in practice. D. Putting Things Together In summary, after all the above discussions, RSS holds well either in complex indoor environment (i.e., multipath exists) or IEEE INFOCOM 2018 - IEEE Conference on Computer Communications