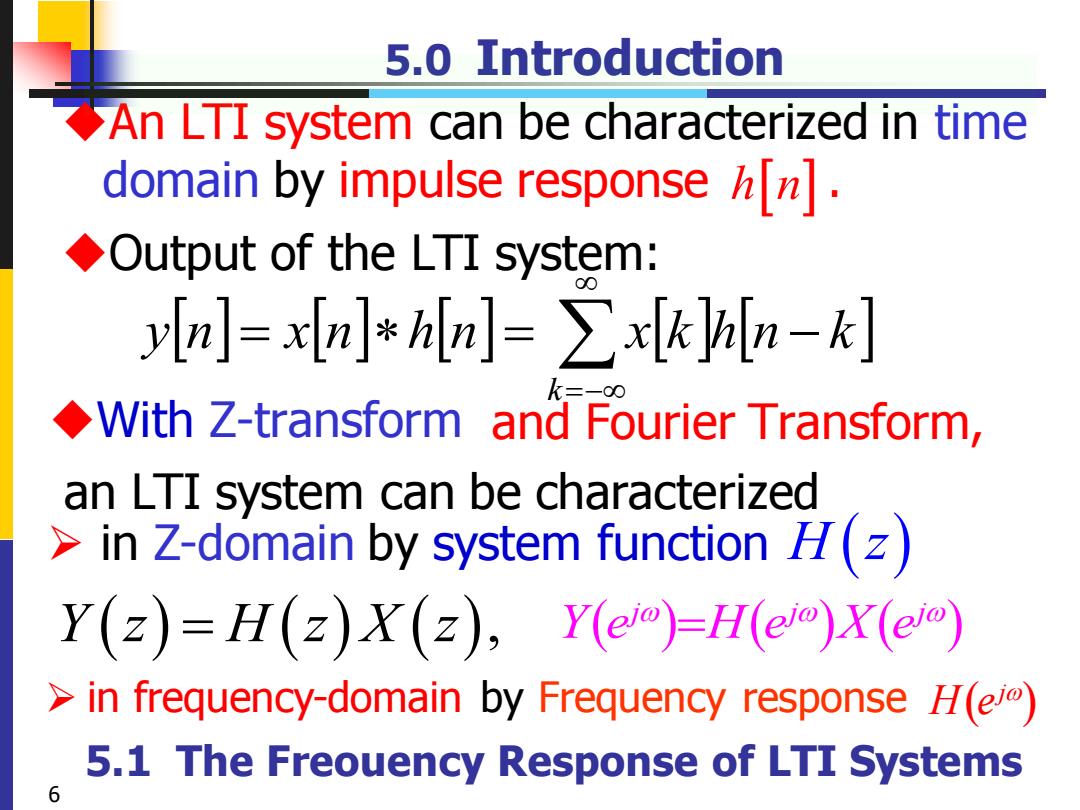
5.0 Introduction An LTI system can be characterized in time domain by impulse response[]. Output of the LTI system: yIn]=xIn]*hin]=>xkhin-k] With Z-transform and Fourier Transform, an LTI system can be characterized >in Z-domain by system function H(z) Y(z)=H(z)X(z),Y(e)=H()x(e) in frequency-domain by Frequency response H(e) 5.1 The Freouency Response of LTI Systems 65.0 Introduction ◆An LTI system can be characterized in time domain by impulse response . ◆Output of the LTI system: h n              =− =  = − k y n x n h n x k h n k Y z H z X z ( ) = ( ) ( ), ➢ in Z-domain by system function ➢ in frequency-domain by Frequency response ( ) ( ) ( ) j j j Y e H e X e    = ◆With Z-transform an LTI system can be characterized H z( ) ( ) H ej and Fourier Transform, 6 5.1 The Freouency Response of LTI Systems