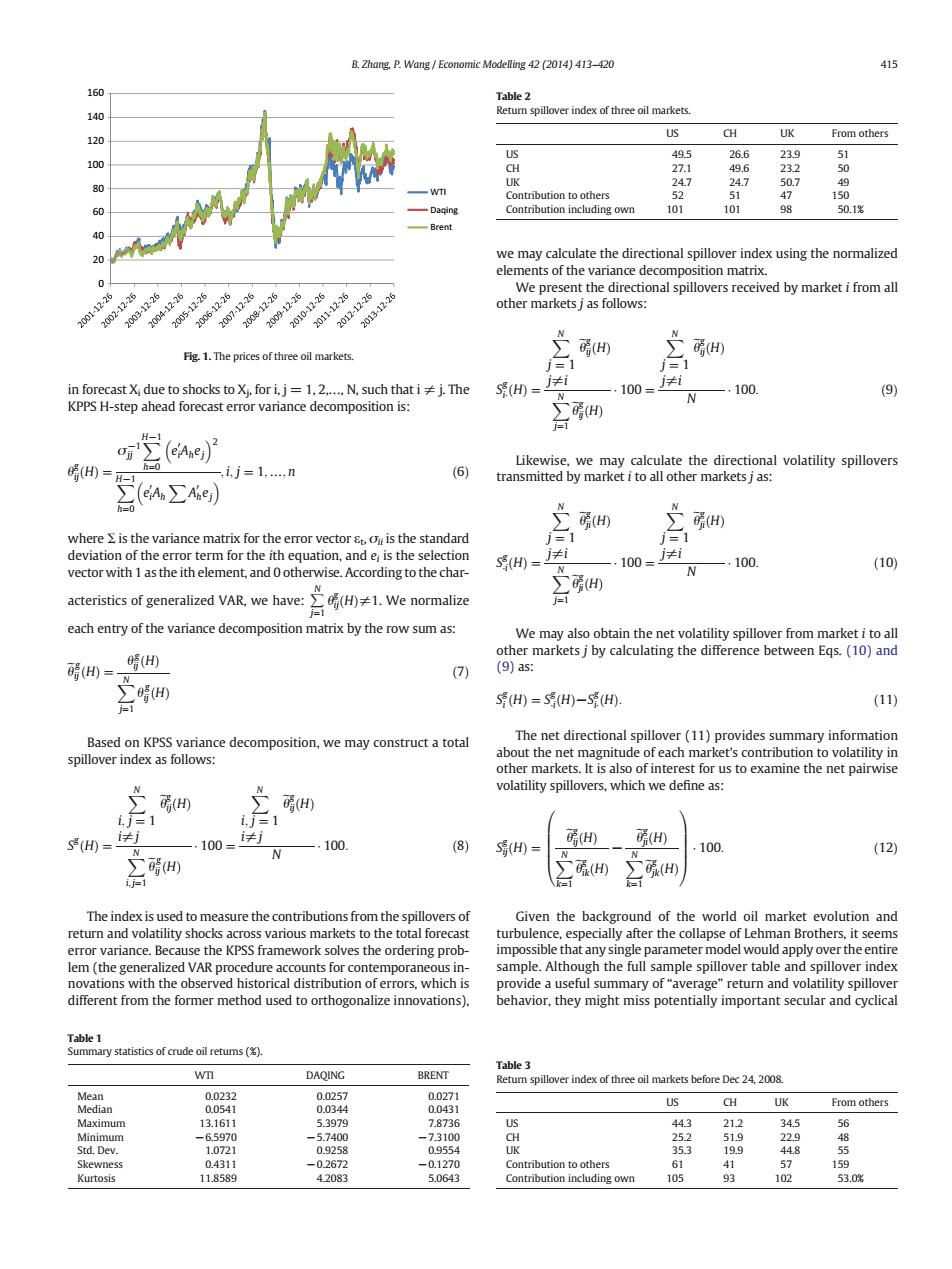
B.Zhang,P.Wang Economic Modelling 42 (2014)413-420 415 160 Table 2 Return spillover index of three oil markets. 120 US CH UK From others US 49.5 26.6 23.9 CH 27.1 49.6 23.2 50 UK 247 24.7 50.7 49 w Contnbution to others 52 51 47 Contribution including own 101 101 98 50.1% Brent 40 we may calculate the directional spillover index using the normalized elements of the variance decomposition matrix. We present the directional spillovers received by market i from all 2002-12-26 001-12-26 2004-12-26 200312-26 200512-26 207-12-26 2006-12-26 209-12-26 2008-12-26 202-12-26 2011-12-26 2010-12-26 2013-1226 other markets j as follows: N N (H) Fig.1.The prices of three oil markets H i=1 in forecast X due to shocks to Xj.for i.j=1.2.....N,such that i j.The (H) j≠i 100 ≠i 100 (9) KPPS H-step ahead forecast error variance decomposition is: H- 听(eg)2 Likewise,we may calculate the directional volatility spillovers (H= =0 i,j=1,,n (6) transmitted by market i to all other markets j as: h=0 (H) 疏(H) where E is the variance matrix for the error vector st.ou is the standard i= deviation of the error term for the ith equation,and e,is the selection j≠i j≠i S(H) 100= ,100 (10) vector with 1 as the ith element,and 0 otherwise.According to the char- N (H) acteristics of generalized VAR,we have:(H)1.We normalize each entry of the variance decomposition matrix by the row sum as: We may also obtain the net volatility spillover from market i to all other markets j by calculating the difference between Eqs.(10)and 醋(H= H (7 (9)as: ∑H S(H)=S(H)-S (H). (11) Based on KPSS variance decomposition,we may construct a total The net directional spillover (11)provides summary information spillover index as follows: about the net magnitude of each market's contribution to volatility in other markets.It is also of interest for us to examine the net pairwise volatility spillovers,which we define as: H (H） i,j=1 i.j=1 S(H= 100= i≠j ,100 (8) 丽 N (H)= 100 (12) H) Ok(H) （ i.j-1 =1 The index is used to measure the contributions from the spillovers of Given the background of the world oil market evolution and return and volatility shocks across various markets to the total forecast turbulence,especially after the collapse of Lehman Brothers,it seems error variance.Because the KPSS framework solves the ordering prob- impossible that any single parameter model would apply over the entire lem (the generalized VAR procedure accounts for contemporaneous in- sample.Although the full sample spillover table and spillover index novations with the observed historical distribution of errors,which is provide a useful summary of "average"return and volatility spillover different from the former method used to orthogonalize innovations) behavior,they might miss potentially important secular and cyclical Table 1 Summary statistics of crude oil retums () Table 3 wn DAQING BRENT Return spillover index of three oil markets before Dec 24,2008. Mean 0.0232 0.0257 0.0271 Median 0.0541 0.0344 0.0431 US CH UK From others Maximum 13.1611 53979 7.8736 US 44.3 21.2 34.5 56 Minimum -6.5970 -5.7400 -7.3100 CH 252 51.9 22.9 Std.Dev. 1.0721 0.9258 0.9554 UK 35.3 19.9 44.8 Skewness 0.4311 -02672 -0.1270 Contribution to others 61 41 57 159 Kurtosis 11.8589 42083 5.0643 Contribution including own 105 93 102 53.0%in forecast Xi due to shocks to Xj, for i, j = 1, 2,…, N, such that i ≠ j. The KPPS H-step ahead forecast error variance decomposition is: θ g ijð Þ¼ H σ−1 jj H X−1 h¼0 e 0 iAhej  2 H X−1 h¼0 e 0 iAh XA0 hej  ; i; j ¼ 1; …; n ð6Þ where Σ is the variance matrix for the error vector εt, σii is the standard deviation of the error term for the ith equation, and ei is the selection vector with 1 as the ith element, and 0 otherwise. According to the char￾acteristics of generalized VAR, we have: ∑ N j¼1 θ g ijð Þ H ≠1. We normalize each entry of the variance decomposition matrix by the row sum as: eθ g ijð Þ¼ H θ g ijð Þ H XN j¼1 θ g ijð Þ H ð7Þ Based on KPSS variance decomposition, we may construct a total spillover index as follows: S g ð Þ¼ H XN i; j ¼ 1 i≠j eθ g ijð Þ H XN i; j¼1 eθ g ijð Þ H 100 ¼ XN i; j ¼ 1 i≠j eθ g ijð Þ H N 100: ð8Þ The index is used to measure the contributions from the spillovers of return and volatility shocks across various markets to the total forecast error variance. Because the KPSS framework solves the ordering prob￾lem (the generalized VAR procedure accounts for contemporaneous in￾novations with the observed historical distribution of errors, which is different from the former method used to orthogonalize innovations), we may calculate the directional spillover index using the normalized elements of the variance decomposition matrix. We present the directional spillovers received by market i from all other markets j as follows: S g i ð Þ¼ H XN j ¼ 1 j≠i eθ g ijð Þ H XN j¼1 eθ g ijð Þ H 100 ¼ XN j ¼ 1 j≠i eθ g ijð Þ H N 100: ð9Þ Likewise, we may calculate the directional volatility spillovers transmitted by market i to all other markets j as: S g i ð Þ H ¼ XN j ¼ 1 j≠i eθ g jið Þ H XN j¼1 eθ g jið Þ H 100 ¼ XN j ¼ 1 j≠i eθ g jið Þ H N 100: ð10Þ We may also obtain the net volatility spillover from market i to all other markets j by calculating the difference between Eqs. (10) and (9) as: S g i ð Þ¼ H S g i ð Þ H −S g i ð Þ H : ð11Þ The net directional spillover (11) provides summary information about the net magnitude of each market's contribution to volatility in other markets. It is also of interest for us to examine the net pairwise volatility spillovers, which we define as: S g ijð Þ¼ H eθ g ijð Þ H XN k¼1 eθ g ikð Þ H − eθ g jið Þ H XN k¼1 eθ g jkð Þ H 0 BBBB@ 1 CCCCA 100: ð12Þ Given the background of the world oil market evolution and turbulence, especially after the collapse of Lehman Brothers, it seems impossible that any single parameter model would apply over the entire sample. Although the full sample spillover table and spillover index provide a useful summary of “average” return and volatility spillover behavior, they might miss potentially important secular and cyclical 0 20 40 60 80 100 120 140 160 WTI Daqing Brent Fig. 1. The prices of three oil markets. Table 1 Summary statistics of crude oil returns (%). WTI DAQING BRENT Mean 0.0232 0.0257 0.0271 Median 0.0541 0.0344 0.0431 Maximum 13.1611 5.3979 7.8736 Minimum −6.5970 −5.7400 −7.3100 Std. Dev. 1.0721 0.9258 0.9554 Skewness 0.4311 −0.2672 −0.1270 Kurtosis 11.8589 4.2083 5.0643 Table 2 Return spillover index of three oil markets. US CH UK From others US 49.5 26.6 23.9 51 CH 27.1 49.6 23.2 50 UK 24.7 24.7 50.7 49 Contribution to others 52 51 47 150 Contribution including own 101 101 98 50.1% Table 3 Return spillover index of three oil markets before Dec 24, 2008. US CH UK From others US 44.3 21.2 34.5 56 CH 25.2 51.9 22.9 48 UK 35.3 19.9 44.8 55 Contribution to others 61 41 57 159 Contribution including own 105 93 102 53.0% B. Zhang, P. Wang / Economic Modelling 42 (2014) 413–420 415