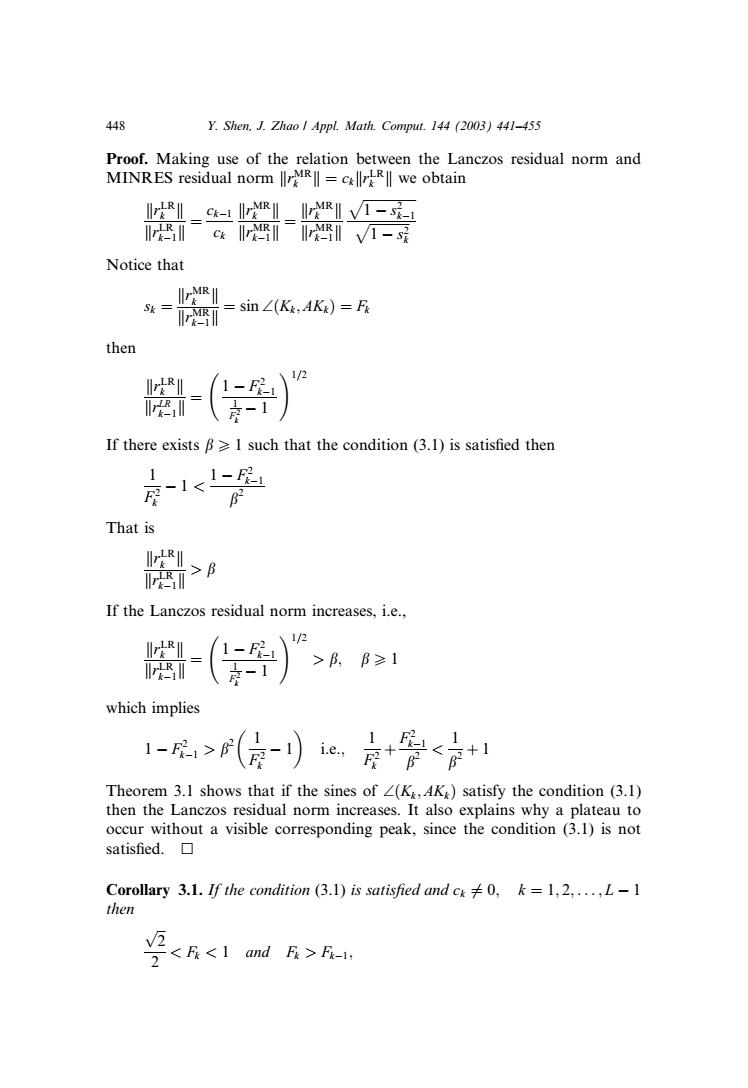
448 Y.Shen,J.Zhao Appl.Math.Comput.144 (2003)441-455 Proof.Making use of the relation between the Lanczos residual norm and MINRES residual norm MR‖l=C&lTER‖we obtain RI C-1 MRI RII V1--1 Ck 1- Notice that 会 T sin∠(Kk,AKe)=F then F21 -1 If there exists B>1 such that the condition (3.1)is satisfied then That is >8 T If the Lanczos residual norm increases,i.e., >B,B≥1 which implies 1-民>（房-ie, Theorem 3.1 shows that if the sines of (K,AK)satisfy the condition (3.1) then the Lanczos residual norm increases.It also explains why a plateau to occur without a visible corresponding peak,since the condition (3.1)is not satisfied.▣ Corollary 3.1.If the condition (3.1)is satisfied and c&0,k =1,2,...,L-I then 2<R<I and F>F-1,Proof. Making use of the relation between the Lanczos residual norm and MINRES residual norm krMR k k ¼ ckkrLR k k we obtain krLR k k krLR k1k ¼ ck1 ck krMR k k krMR k1k ¼ krMR k k krMR k1k ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi 1  s2 k1 p ffiffiffiffiffiffiffiffiffiffiffiffi 1  s2 k p Notice that sk ¼ krMR k k krMR k1k ¼ sin \ðKk ; AKk Þ ¼ Fk then krLR k k krLR k1k ¼ 1  F 2 k1 1 F 2 k  1 !1=2 If there exists b P1 such that the condition (3.1) is satisfied then 1 F 2 k  1 < 1  F 2 k1 b2 That is krLR k k krLR k1k > b If the Lanczos residual norm increases, i.e., krLR k k krLR k1k ¼ 1  F 2 k1 1 F 2 k  1 !1=2 > b; b P1 which implies 1  F 2 k1 > b2 1 F 2 k  1 i:e:; 1 F 2 k þ F 2 k1 b2 < 1 b2 þ 1 Theorem 3.1 shows that if the sines of \ðKk ; AKk Þ satisfy the condition (3.1) then the Lanczos residual norm increases. It also explains why a plateau to occur without a visible corresponding peak, since the condition (3.1) is not satisfied. Corollary 3.1. Ifthe condition (3.1) is satisfied and ck 6¼ 0; k ¼ 1; 2; ... ; L  1 then ffiffiffi 2 p 2 < Fk < 1 and Fk > Fk1; 448 Y. Shen, J. Zhao / Appl. Math. Comput. 144 (2003) 441–455