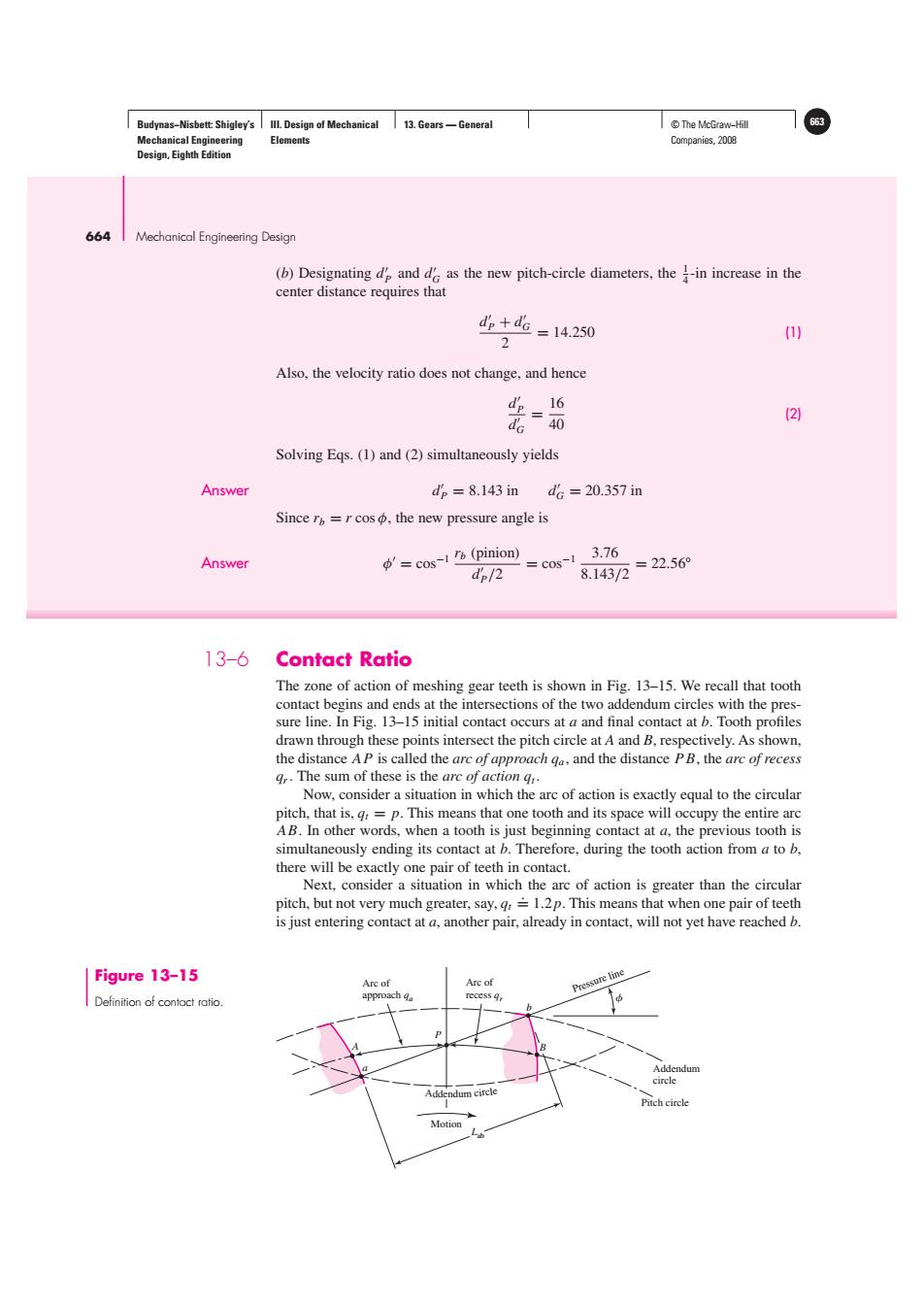
Budynas-Nisbett:Shigley's Ill.Design of Mechanical 13.Gears-General ©The McGraw-Hil 63 Mechanical Engineering Elements Companies,2008 Design,Eighth Edition 664 Mechanical Engineering Design (b)Designating de and d as the new pitch-circle diameters,the -in increase in the center distance requires that d,+d6=14.250 2 () Also,the velocity ratio does not change,and hence 2 Solving Eqs.(1)and(2)simultaneously yields Answer dp=8.143ind6=20.357in Since ro=rcos中，the new pressure angle is Answer =cos-1 (pinion) dp/2 COS-1_ .76 =22.56° .143/2 13-6 Contact Ratio The zone of action of meshing gear teeth is shown in Fig.13-15.We recall that tooth contact begins and ends at the intersections of the two addendum circles with the pres- sure line.In Fig.13-15 initial contact occurs at a and final contact at b.Tooth profiles drawn through these points intersect the pitch circle at A and B,respectively.As shown, the distance AP is called the arc of approach qa.and the distance PB,the arc of recess q.The sum of these is the arc of action q. Now,consider a situation in which the arc of action is exactly equal to the circular pitch,that is.q=p.This means that one tooth and its space will occupy the entire arc AB.In other words,when a tooth is just beginning contact at a,the previous tooth is simultaneously ending its contact at b.Therefore,during the tooth action from a to b, there will be exactly one pair of teeth in contact. Next,consider a situation in which the arc of action is greater than the circular pitch,but not very much greater,say,1.2p.This means that when one pair of teeth is just entering contact at a,another pair,already in contact,will not yet have reached b. Figure 13-15 Arc of Arc of Pressure line Definition of contact ratio. appro 中 Addendum circle Pitch circle MotionBudynas−Nisbett: Shigley’s Mechanical Engineering Design, Eighth Edition III. Design of Mechanical Elements 13. Gears — General © The McGraw−Hill 663 Companies, 2008 664 Mechanical Engineering Design Lab Motion A a b B Addendum circle Pressure line Pitch circle Addendum circle Arc of approach qa Arc of recess qr P Figure 13–15 Definition of contact ratio. (b) Designating d P and d G as the new pitch-circle diameters, the 1 4 -in increase in the center distance requires that d P + d G 2 = 14.250 (1) Also, the velocity ratio does not change, and hence d P d G = 16 40 (2) Solving Eqs. (1) and (2) simultaneously yields Answer d P = 8.143 in d G = 20.357 in Since rb = r cos φ, the new pressure angle is Answer φ = cos−1 rb (pinion) d P/2 = cos−1 3.76 8.143/2 = 22.56◦ 13–6 Contact Ratio The zone of action of meshing gear teeth is shown in Fig. 13–15. We recall that tooth contact begins and ends at the intersections of the two addendum circles with the pres￾sure line. In Fig. 13–15 initial contact occurs at a and final contact at b. Tooth profiles drawn through these points intersect the pitch circle at A and B, respectively. As shown, the distance AP is called the arc of approach qa , and the distance P B, the arc of recess qr. The sum of these is the arc of action qt . Now, consider a situation in which the arc of action is exactly equal to the circular pitch, that is, qt = p. This means that one tooth and its space will occupy the entire arc AB. In other words, when a tooth is just beginning contact at a, the previous tooth is simultaneously ending its contact at b. Therefore, during the tooth action from a to b, there will be exactly one pair of teeth in contact. Next, consider a situation in which the arc of action is greater than the circular pitch, but not very much greater, say, qt . = 1.2p. This means that when one pair of teeth is just entering contact at a, another pair, already in contact, will not yet have reached b.