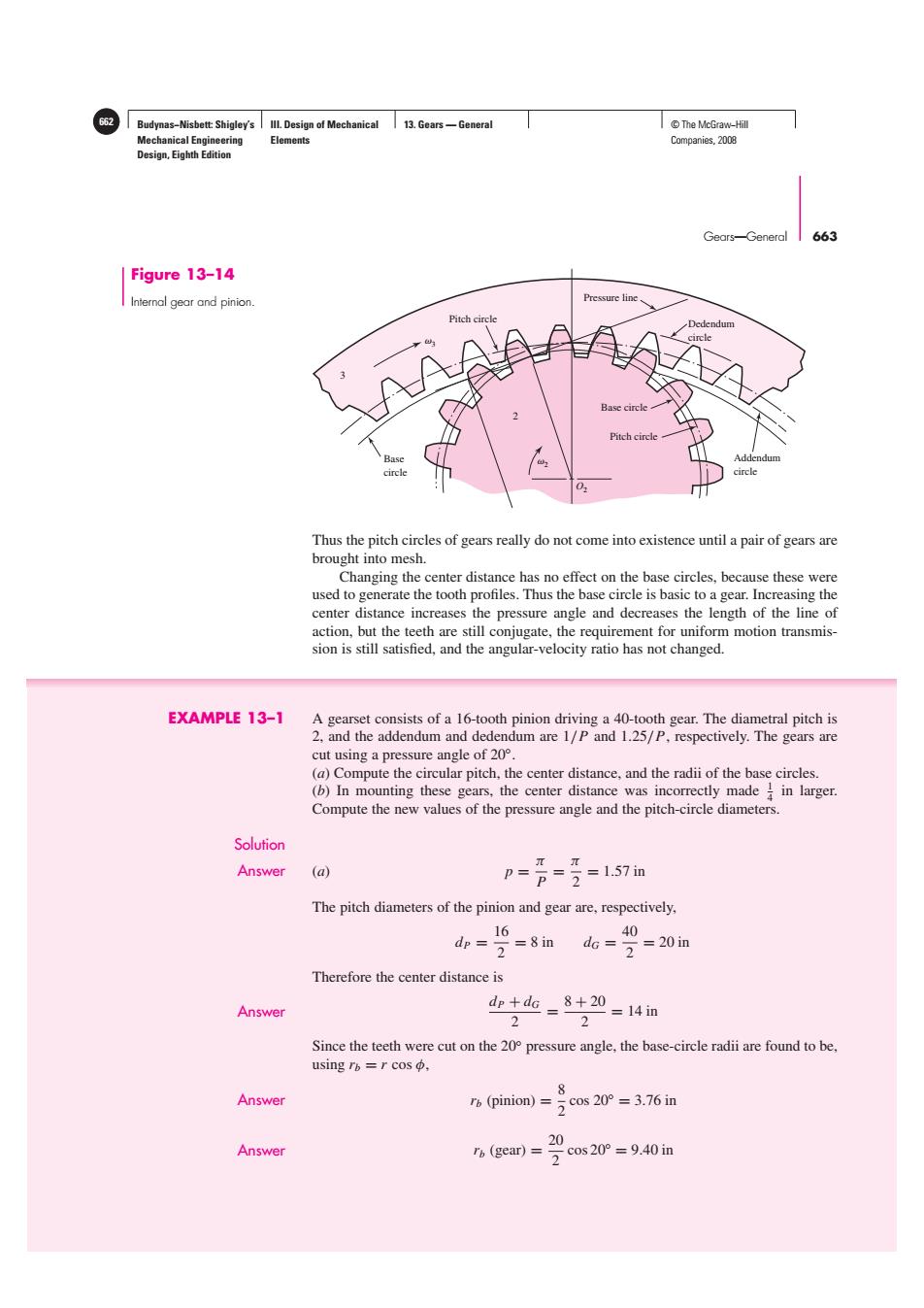
662 Budynas-Nisbett:Shigley's Ill.Design of Mechanical 13.Gears-General T©The McGraw-Hil Mechanical Engineering Elements Companies,2008 Design,Eighth Edition Gears-General 663 Figure 13-14 Internal gear and pinion. Pressure line Pitch circk Pitch circle Addendum circle Thus the pitch circles of gears really do not come into existence until a pair of gears are brought into mesh. Changing the center distance has no effect on the base circles,because these were used to generate the tooth profiles.Thus the base circle is basic to a gear.Increasing the center distance increases the pressure angle and decreases the length of the line of action,but the teeth are still conjugate,the requirement for uniform motion transmis- sion is still satisfied,and the angular-velocity ratio has not changed. EXAMPLE 13-1 A gearset consists of a 16-tooth pinion driving a 40-tooth gear.The diametral pitch is 2.and the addendum and dedendum are 1/P and 1.25/P,respectively.The gears are cut using a pressure angle of 20. (a)Compute the circular pitch,the center distance,and the radii of the base circles. (b)In mounting these gears,the center distance was incorrectly made in larger. Compute the new values of the pressure angle and the pitch-circle diameters. Solution ππ Answer (a) =2=1.57in p= The pitch diameters of the pinion and gear are,respectively, 16 40 d=2=8ind6=2=20in Therefore the center distance is Answer dp+dc=8+20 2 =14in 2 Since the teeth were cut on the 20 pressure angle,the base-circle radii are found to be, using ro=rcosφ， 8 Answer n(pinion）=2cos20°=3.76in 20 Answer ro (gear)= cos20°-9.40inBudynas−Nisbett: Shigley’s Mechanical Engineering Design, Eighth Edition III. Design of Mechanical Elements 13. Gears — General 662 © The McGraw−Hill Companies, 2008 Gears—General 663 Thus the pitch circles of gears really do not come into existence until a pair of gears are brought into mesh. Changing the center distance has no effect on the base circles, because these were used to generate the tooth profiles. Thus the base circle is basic to a gear. Increasing the center distance increases the pressure angle and decreases the length of the line of action, but the teeth are still conjugate, the requirement for uniform motion transmis￾sion is still satisfied, and the angular-velocity ratio has not changed. EXAMPLE 13–1 A gearset consists of a 16-tooth pinion driving a 40-tooth gear. The diametral pitch is 2, and the addendum and dedendum are 1/P and 1.25/P, respectively. The gears are cut using a pressure angle of 20◦. (a) Compute the circular pitch, the center distance, and the radii of the base circles. (b) In mounting these gears, the center distance was incorrectly made 1 4 in larger. Compute the new values of the pressure angle and the pitch-circle diameters. Solution Answer (a) p = π P = π 2 = 1.57 in The pitch diameters of the pinion and gear are, respectively, dP = 16 2 = 8 in dG = 40 2 = 20 in Therefore the center distance is Answer dP + dG 2 = 8 + 20 2 = 14 in Since the teeth were cut on the 20◦ pressure angle, the base-circle radii are found to be, using rb = r cos φ, Answer rb (pinion) = 8 2 cos 20◦ = 3.76 in Answer rb (gear) = 20 2 cos 20◦ = 9.40 in Pitch circle Base circle 2 Base circle Pitch circle Pressure line Dedendum circle Addendum circle 3 2 3 O2 Figure 13–14 Internal gear and pinion.