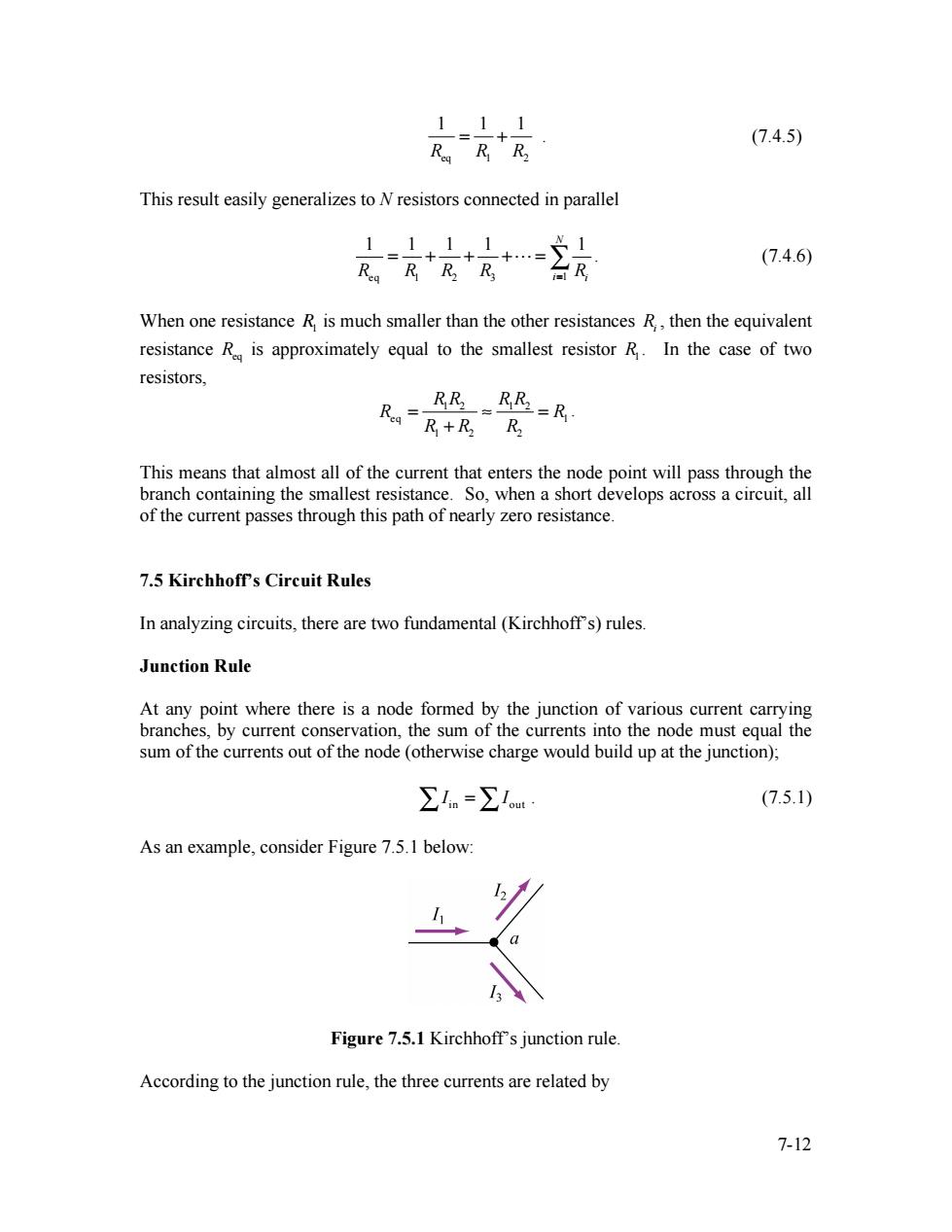
111 (7.4.5) Reg R R2 This result easily generalizes to N resistors connected in parallel 1_1+1+1+=】 (7.4.6) Reg R R2 R3 When one resistance R is much smaller than the other resistances R,then the equivalent resistance R is approximately equal to the smallest resistor R.In the case of two resistors, RR:-RR--R Reg=R+R R2 This means that almost all of the current that enters the node point will pass through the branch containing the smallest resistance.So,when a short develops across a circuit,all of the current passes through this path of nearly zero resistance 7.5 Kirchhoffs Circuit Rules In analyzing circuits,there are two fundamental(Kirchhoff's)rules. Junction Rule At any point where there is a node formed by the junction of various current carrying branches,by current conservation,the sum of the currents into the node must equal the sum of the currents out of the node (otherwise charge would build up at the junction); ∑1n=∑1out (7.5.1) As an example,consider Figure 7.5.1 below: Figure 7.5.1 Kirchhoff's junction rule. According to the junction rule,the three currents are related by 7-127-12 eq 1 2 1 1 1 R R R = + . (7.4.5) This result easily generalizes to N resistors connected in parallel eq 1 2 3 1 1 1 1 1 1 N i R R R R = Ri = + + + = ∑ . (7.4.6) When one resistance ￾ R1 is much smaller than the other resistances Ri , then the equivalent resistance Req is approximately equal to the smallest resistor R1 . In the case of two resistors, 1 2 1 2 eq 1 1 2 2 R R R R R R R R R = ≈ = + . This means that almost all of the current that enters the node point will pass through the branch containing the smallest resistance. So, when a short develops across a circuit, all of the current passes through this path of nearly zero resistance. 7.5 Kirchhoff’s Circuit Rules In analyzing circuits, there are two fundamental (Kirchhoff’s) rules. Junction Rule At any point where there is a node formed by the junction of various current carrying branches, by current conservation, the sum of the currents into the node must equal the sum of the currents out of the node (otherwise charge would build up at the junction); in out ∑I = ∑I . (7.5.1) As an example, consider Figure 7.5.1 below: Figure 7.5.1 Kirchhoff’s junction rule. According to the junction rule, the three currents are related by