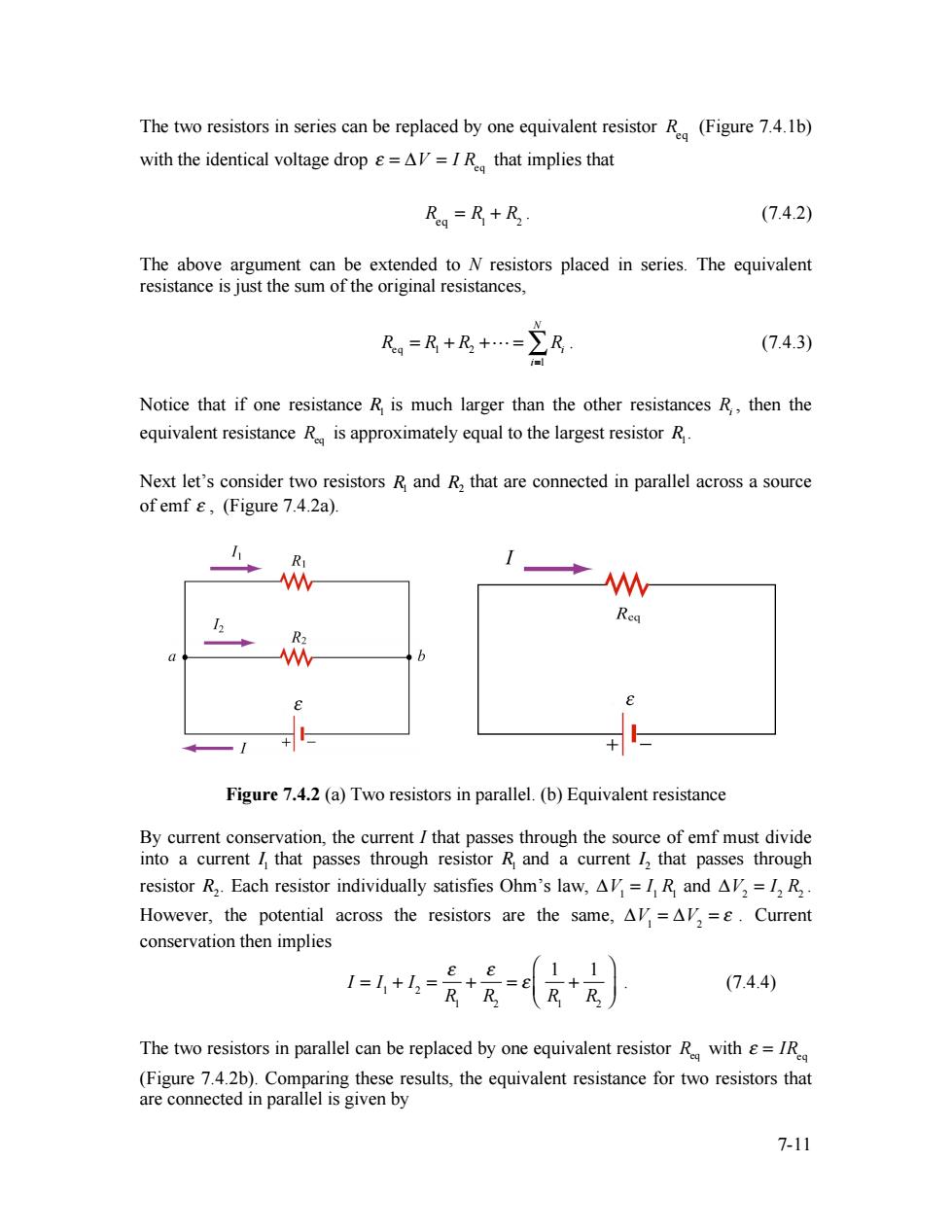
The two resistors in series can be replaced by one equivalent resistor R(Figure 7.4.1b) with the identical voltage drop =AV=IR that implies that Reg =R+R (7.4.2) The above argument can be extended to N resistors placed in series.The equivalent resistance is just the sum of the original resistances, R=R+R+=立R (7.4.3) Notice that if one resistance R is much larger than the other resistances R,then the equivalent resistance R is approximately equal to the largest resistor R. Next let's consider two resistors R and R,that are connected in parallel across a source of emf g,(Figure 7.4.2a). R W Reg Figure 7.4.2 (a)Two resistors in parallel.(b)Equivalent resistance By current conservation,the current that passes through the source of emf must divide into a current I that passes through resistor R and a current 1,that passes through resistor R2.Each resistor individually satisfies Ohm's law,AV=IR and AV2=12 R. However,the potential across the resistors are the same,AV=AV,=g.Current conservation then implies (7.4.4) The two resistors in parallel can be replaced by one equivalent resistor R with e=IR (Figure 7.4.2b).Comparing these results,the equivalent resistance for two resistors that are connected in parallel is given by 7-117-11 The two resistors in series can be replaced by one equivalent resistor Req (Figure 7.4.1b) with the identical voltage drop ε = ΔV = I Req that implies that Req = R1 + R2 . (7.4.2) The above argument can be extended to N resistors placed in series. The equivalent resistance is just the sum of the original resistances, eq 1 2 1 N i i R R R R = = + + = ∑ . (7.4.3) Notice that if one resistance ￾ R1 is much larger than the other resistances Ri , then the equivalent resistance Req is approximately equal to the largest resistor ￾ R1. Next let’s consider two resistors ￾ R1 and ￾ R2 that are connected in parallel across a source of emf ε , (Figure 7.4.2a). Figure 7.4.2 (a) Two resistors in parallel. (b) Equivalent resistance By current conservation, the current I that passes through the source of emf must divide into a current ￾ I 1 that passes through resistor ￾ R1 and a current ￾ I2 that passes through resistor ￾ R2. Each resistor individually satisfies Ohm’s law, ΔV1 = I 1 R1 and ΔV2 = I2 R2 . However, the potential across the resistors are the same, ΔV1 = ΔV2 = ε . Current conservation then implies I = I 1 + I2 = ε R1 + ε R2 = ε 1 R1 + 1 R2 ⎛ ⎝ ⎜ ⎞ ⎠ ⎟ . (7.4.4) The two resistors in parallel can be replaced by one equivalent resistor Req with ε = IReq (Figure 7.4.2b). Comparing these results, the equivalent resistance for two resistors that are connected in parallel is given by