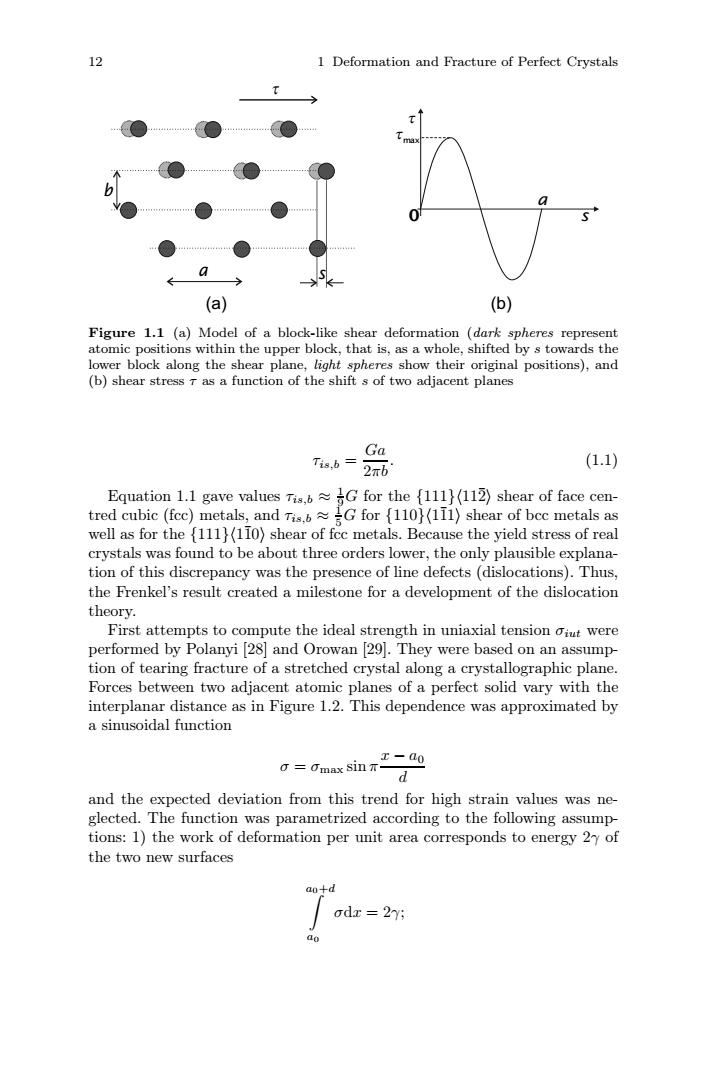
12 1 Deformation and Fracture of Perfect Crystals (a) (b) Figure 1.1 (a)Model of a block-like shear deformation (dark spheres represent atomic positions within the upper block,that is,as a whole,shifted by s towards the lower block along the shear plane,light spheres show their original positions),and (b)shear stress T as a function of the shift s of two adjacent planes Ga Tis,b= 2xb (1.1) Equation 1.1 gave valuesis,G for the {111)(112)shear of face cen- tred cubic (fcc)metals,and Tis,G for {110)(111)shear of bcc metals as well as for the {111(110)shear of fcc metals.Because the yield stress of real crystals was found to be about three orders lower,the only plausible explana- tion of this discrepancy was the presence of line defects (dislocations).Thus, the Frenkel's result created a milestone for a development of the dislocation theory. First attempts to compute the ideal strength in uniaxial tension oiut were performed by Polanyi [28 and Orowan 29.They were based on an assump- tion of tearing fracture of a stretched crystal along a crystallographic plane. Forces between two adjacent atomic planes of a perfect solid vary with the interplanar distance as in Figure 1.2.This dependence was approximated by a sinusoidal function =dmax sinnto d and the expected deviation from this trend for high strain values was ne- glected.The function was parametrized according to the following assump- tions:1)the work of deformation per unit area corresponds to energy 2y of the two new surfaces ao+d odx 2y; ao12 1 Deformation and Fracture of Perfect Crystals a s b a s 0 max (a) (b) Figure 1.1 (a) Model of a block-like shear deformation (dark spheres represent atomic positions within the upper block, that is, as a whole, shifted by s towards the lower block along the shear plane, light spheres show their original positions), and (b) shear stress τ as a function of the shift s of two adjacent planes τis,b = Ga 2πb . (1.1) Equation 1.1 gave values τis,b ≈ 1 9G for the {111}11¯2 shear of face cen￾tred cubic (fcc) metals, and τis,b ≈ 1 5G for {110}1¯11 shear of bcc metals as well as for the {111}1¯10 shear of fcc metals. Because the yield stress of real crystals was found to be about three orders lower, the only plausible explana￾tion of this discrepancy was the presence of line defects (dislocations). Thus, the Frenkel’s result created a milestone for a development of the dislocation theory. First attempts to compute the ideal strength in uniaxial tension σiut were performed by Polanyi [28] and Orowan [29]. They were based on an assump￾tion of tearing fracture of a stretched crystal along a crystallographic plane. Forces between two adjacent atomic planes of a perfect solid vary with the interplanar distance as in Figure 1.2. This dependence was approximated by a sinusoidal function σ = σmax sin π x − a0 d and the expected deviation from this trend for high strain values was ne￾glected. The function was parametrized according to the following assump￾tions: 1) the work of deformation per unit area corresponds to energy 2γ of the two new surfaces a0+d a0 σdx = 2γ;